Q: How much of physics can be deduced from previous equations/axioms?

Posted on August 17, 2011 by The Physicist

The original question was: I was wondering quite how much of physics (mainly regarding classical mechanics but all branches!) can be deduced from previous equations/axioms. I don’t mean going as far back as what axioms we have to take in maths, but I’ve always loved proofs deriving equations from others. And obviously some values need to be experimentally derived, like g=9.81, or the value of a coefficient of friction. But you can still derive equations which feature these without knowing their value.  So how was F=ma derived etc?

Physicist: That is really profound!  It depends on where you’d be willing to draw the line.  In terms of what you might learn in a physics class: most of it.  In each physics class (although it doesn’t always seem like it) there are usually only a handful of equations to learn, and many of those can be derived (in later classes).

In that particular example, force is actually defined to be mass times acceleration.
Before Newton the words “force” and “energy” were thrown around, but not defined rigorously.  There’s a long tradition of scientists grabbing words that have a vague meaning in everyday language and giving them (forcing upon them) solid mathematical definitions, like: energy, jerk, information, temperature, significant, curl, almost-everywhere, …

But the basic laws like “for every action there’s an equal but opposite reaction”, and the associated equations, are probably a lot like the g=9.81 thing; they need to be experimentally discovered.

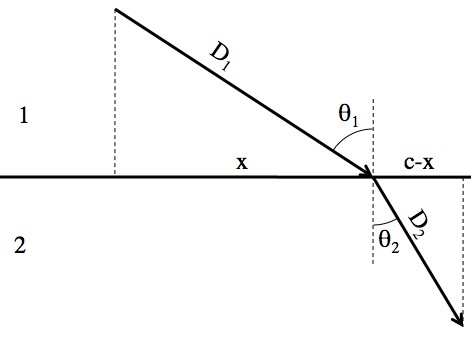
For example (when m is mass and v is velocity),

m, mv, and mv2 (matter, momentum, and kinetic energy) are  conserved, but mv3 is not conserved, and doesn’t even get a name.  There’s no particularly good reason why mv3 isn’t conserved, or why mv2 is.

You can go back and forth, and argue the why’s, but at the end of the day there will always be a list of true but un-derivable laws called “first principles”.  These are just the simple, generally agreed upon, but unprovable statements of physics.  Like “charge creates an electric field” or “mass creates gravity” or “momentum is conserved”.

You can find a few unprovable-but-true statements by saying almost anything, and then asking “why?” over and over until you start to loop.

So, technically everything is either an axiom or is derived from one.  Most of the big advancements in physics come from discovering the new, and absolutely not derivable, axioms of the universe.  Like “the speed of light is constant, no matter what” or “there’s a limit to how well momentum and position can be simultaneously measured”.  Nobody saw those coming.

What’s terrible is that it’s (often) impossible to tell which laws and universal constants are fundamental, and which are derived from other fundamental things.  For example, near the end of the 19th century Maxwell (of Maxwell equations fame) derived the speed of light, c,  from \epsilon_0 and \mu_0, the electric and magnetic permittivity of space (which describe how strong the electric and magnetic forces are).  So you’d expect that one, or both, of \epsilon_0 and \mu_0 must be fundamental, and c must be derived.

However!  In the 20th century Einstein rewrote electromagnetism in terms of relativity (in fact, this was the topic of the original relativity paper).  Using relativity and Coulomb’s law (which describes the relationship between distance, charge, and electric force) you can derive anything you’d want to know about magnetism, including \mu_0.  So clearly c and \epsilon_0 are fundamental, while \mu_0 is derived.

Point is: it’s hard to say what’s derived and what’s not, but what we can say is that there are a bunch of laws that just kinda “are”, and can’t be explained by more fundamental laws.  At last count there are a couple dozen fundamental constants (like the ones in the example above) that can’t be explained in terms of each other, or any laws.  Although most physicists would probably agree that there are plenty, I’ve never heard of anyone actually attempting to count the number of fundamental laws that are out there.

Trying to count the fundamental laws seems like a good way to goad physicists into a fist fight.

Posted in -- By the Physicist, Philosophical | 12 Comments

Q: If God were all-seeing and all-knowing, the double-slit experiment wouldn’t work, would it? Wouldn’t God’s observation of the location of the photon collapse its probability wave function?

Posted on August 14, 2011 by The Physicist

Physicist: Deep…

It depends on how entangled God is with the rest of us. If He can make the observation, and then keep a completely straight face forever (never leak any information about the result in any way), then His mighty observation would not collapse the wave, from our perspective.

That’s why sparrows can be in so many places! (Matthew 10:29)

When you hear about “observations”, in the context of quantum mechanics, it usually takes the form of some smart-looking, glasses-wearing, dude saying things like “a particle is in a ‘super-position’ of states until someone observes it, and then suddenly it’s in only one state”.  The misconception here is that the universe as a whole (which includes people and Gods and whatnot) is in one state, and only tiny things can be in many states.

The traditional thought experiment for talking about this is “Schrödinger’s Cat”.  Schrödinger puts his cat into a purfectly information-proof box, so that there’s no way to see what’s going on inside.  Also, he rigs up something deadly that has some chance of killing the cat in the next day or so.  Before the box is opened, the cat is both alive and dead, but afterward the cat is either alive or dead.  The cat undergoes “wave function collapse”.

This is a metaphor for, for example, the photons in the double slit experiment.  Each can demonstrably go through both slits, but if you determine which slit a photon goes through, then it only goes through one.

Unfortunately, this has been taken as a rallying cry for a lot of bad physics.  A better thought experiment (and one which gets closer to explaining the answer to the question) is “Schrödinger’s Graduate Student”.

Repeat the cat in a box experiment, but this time Schrödinger finds that, overcome by quantum grief, he cannot bring himself to open the box.  Instead, he goes out for tea or something and has a graduate student complete the experiment for him.  Once done, the student then finds Schrödinger and reports the results.

But, since Prof. Schrödinger hasn’t observed the graduate student yet, from his perspective the cat and student are still in a super-position of states.  The only difference is that now they’re entangled.  They’re in a combination of the “dead-cat/horrified-student” and “living-cat/cautiously-optimistic-student” states.  When the student finally reports the results to Schrödinger, then the cat/student wave function collapses (from his perspective).

In practice of course, there’s information leaking all over the place.  Considering all of the different paths that information can take: chemicals, air movement, stray photons, gravity, etc. it’s really hard to construct “information-proof-anythings”.  If someone on the far side of the planet sneezes you’ll be entangled with that event within moments.  That’s not saying as much as it sounds like it is.  Essentially, you don’t have to worry much about “big and nearby” events (like sneezing on Earth) being in multiple states.

So, say there’s a God, and say that he/she/it/they/we observes every quantum event.  As far as each of us are concerned, unless God somehow conveys the information (burning bush, cheeky glance) he’ll/she’ll/it’ll/they’ll/we’ll be in the same position as the graduate student was before he delivered the results to Schrödinger.

So, in order to keep a quantum event many-stated and quantumy (from our perspective), all that an all-seeing God needs to do is play it cool, and not reveal any clues, whatsoever, about that event.

Posted in -- By the Physicist, Philosophical, Quantum Theory | 23 Comments

Q: How do those “executive ball clicker” things work?

Posted on August 10, 2011 by The Physicist

The original question was: With the game for executives, consisting of several metal balls hanging in a straight, horizontal row (a “Newton’s cradle”), when one ball is lifted and let loose to hit the rest of the row, only one ball at the other end gets swung away in its arc. And so the is case with two balls with two balls,  and with three balls the response is with three balls, etc.

The question: Why the reaction to one ball is always one ball and not two balls to a shorter distance, or the reaction to three balls, one, or two balls to a greater distance, etc?

Physicist: For those readers who haven’t seen one of these since the 80’s, here’s what we’re talking about.

How do CEO's get any work done?

In every collision you have conservation of momentum.  But, when things collide they can heat up, produce sound, or otherwise lose a little kinetic energy.  For example, when you throw two blobs of clay straight at each other: they start out moving, but after the collision they stick together and barely move.  The collisions that lose energy like that are called “inelastic collisions”.

When a collision conserves energy it’s called an “elastic collision”.  As you might imagine, steel balls don’t change shape much when they collide: the collisions are elastic and do conserve kinetic energy almost perfectly.

For a momentum-and-energy-conserving-collision between two balls, one of which is stationary, you can show that the only way for everything to balance out is for the balls to “switch”.

To extend this to the full multi-ball executive ball clicker, you can think of the whole thing as just a string of two-ball collisions.  Ball one hits ball two hits ball three … and each time all of the movement is transferred from one ball to the next.  The exact same idea is responsible even when you swing several balls at the same time: it’s just a string of very rapid two-ball collisions.

If you were to build one of those executive ball clickers with rubber or wooden balls (which would absorb a little kinetic energy) you’d find that you’d get all of the balls moving, instead of just the one on the far end.  The one-ball-in/one-ball-out thing only works with very solid balls.

Answer gravy: The nice thing about a Newton’s Cradle is that the impacts happen in only one direction, which makes the math much easier.

The before (left) and after (right) velocities. Showing that executive ball clickers work boils down to showing that v1 = 0.

If the mass of every ball is m, the velocity of the original moving ball before the collision is w_1 and the velocities of the two balls after the collision are v_1, v_2, then:

Conservation of momentum can be written: mw_1 = mv_1+mv_2

Conservation of energy can be written: \frac{1}{2}m w_1^2= \frac{1}{2}m v_1^2+\frac{1}{2}m v_2^2

You can clean these up a bit to get w_1 = v_1+v_2 and w_1^2=v_1^2+v_2^2

\begin{array}{ll}\left\{\begin{array}{ll}w_1 = v_1+v_2\\w_1^2=v_1^2+v_2^2\end{array}\right. \\\Rightarrow\left\{\begin{array}{ll}w_1^2 = (v_1+v_2)^2\\w_1^2=v_1^2+v_2^2\end{array}\right. \\\Rightarrow (v_1+v_2)^2=v_1^2+v_2^2\\\Rightarrow v_1^2+2v_1v_2+v_2^2=v_1^2+v_2^2\\\Rightarrow 2v_1v_2=0\\\Rightarrow v_1=0 \textrm{ or }v_2=0\\\end{array}

Using that result, and remembering that w1 = v1+v2, the only two possible solutions are “v1=w1, v2=0″ or “v1=0, v2=w1“.  The first solution would imply that the first ball ignores the second and moves through it.  The second solution is what you see: the impact switches which ball is moving and which is stationary.

In the case of an inelastic collision you have a larger initial kinetic energy and a smaller final kinetic energy.  Energy conservation can then be written: E_{initial} = E_{final}+\ell or \frac{1}{2}m w_1^2=\frac{1}{2}m v_1^2+\frac{1}{2}m v_2^2+\ell, where \ell is the energy lost to heat or sound.  Running through the same math as above you’ll find that v_1v_2 = \frac{\ell}{m}.  Suddenly that last “one of them has to be zero” step doesn’t work, and you find that the kinetic energy has to be divided up between the balls.

This question was surprisingly tricky.  The fact that the balls are arranged in a line turns out to be very important.  If the impacts aren’t in a straight line, or if the first ball hits more than one other ball at a time, then the “executive ball clicker effect” goes away.

Posted in -- By the Physicist, Math, Physics | 18 Comments

Q: Why is cold fusion so difficult?

Posted on August 7, 2011 by The Physicist

Physicist: In a nutshell, fusion is about fusing small atoms, specifically hydrogen, together to form larger atoms, specifically helium.  In the processes a lot of energy is released.  However, pressing hydrogen together hard enough to fuse it is very difficult, especially considering that the more you succeed, the bigger an explosion you’ll have.

Cold fusion, means doing this in a controlled, consistent way on a small scale, like a power plant, as opposed to something like a bomb.

The energy associated with two like charges is inversely proportional to their distance.  That is, if you bring two charges a certain distance, D, apart it’ll take X energy.  To half that distance to D/2 will take 2X energy, to half that again to D/4 would take 4X energy, and so on.

Protons (the important players) are so small that you can keep halfing the distance for a long time, so the energy it takes to bring them together is huge.  However, if they get close enough the nuclear strong force kicks in, overwhelms the electric force, and slams them together (releasing far more energy than initially went into forcing them together).

The only way to get them that close is to get them moving really fast.  Fast enough, and with enough energy, that by the time their mutual electric repulsion has had a chance to throw them apart, they’ve come close enough for the strong force to work it’s magic.  The best way to get protons (ionized hydrogen) to move that fast is to heat it (heat is random movement, and a lot of heat is fast random movement).  In order to get protons moving fast enough requires temperatures in excess of at least 5,000,000°C.  However, at temperatures (and speeds) like that things tend to fly apart immediately, so you need to find some way of keeping the pressure very high to keep the gasses in the same place long enough to react.  By comparison, the hottest that the center of a TNT explosion can get is several thousand degrees celcius.

Unfortunately, the pressures and temperatures involved are much, much higher than can be contained with any material known.  I mean, normally you need to park a star on it to keep the reaction contained and active.  There have been some tricks attempted to get around these difficulties.

“Muonic hydrogen” allows the hydrogen to get closer together before the repulsive forces start.  When a proton (positive charge) has an electron (negative charge) around it, the overall charge outside of the electron shell is zero.  This is called “shielding”.  But, if you bring the protons closer together than the size of their electron shells, their charges are no longer shielded from each other, and they repel.  Muons are like a heavy, unstable version of electrons.  One effect of their larger mass is that they form a much smaller shell around the proton, allowing the protons to get much closer together before they start to repel.  Because they can “sneak up on each other”, it takes less energy to bring the protons together.  Unfortunately, muons only exist for a few millions of a second.  In that time, they need to be created, slowed down (the creation event is pretty energetic), paired to a proton to form muonic hydrogen, and then slammed into another similarly prepared muonic hydrogen atom.

The negative charges in orbit around a proton "shield" the proton's positive charges from each other. By orbiting tighter around the proton, muons allow the protons to stay shielded from each other to much shorter distances.

“Magnetic containment” gets around the whole “no material can contain the reaction problem” by getting rid of the material (magnetic fields aren’t made of “stuff”).  This seems to be the best hope for cold fusion.

ITER in France uses magnetic fields to contain a fusion reaction. That glow isn't the reaction. The reaction actually happens in a ring that runs through the middle of the room.

The only “efficient” fusion ever attained on Earth has been in the middle of hydrogen bombs, where the fusion reaction is contained by being sandwiched between several fission explosions (several atomic bombs).

With great effort, and tremendous amounts of energy (for magnets, and heating, and whatnot), you can get a little bit of fusion.  However, the heat energy generated by that fusion doesn’t come close to offsetting the energy that went into getting the fusion to work in the first place.

In dark contrast, you can easily run drills and oil refineries dozens of times over on the energy from the oil they produce.

Posted in -- By the Physicist, Particle Physics, Physics | 6 Comments

Q: Why does light choose the “path of least time”?

Posted on August 5, 2011 by The Physicist

Physicist: Light travels at different speeds in different materials.  When you shine a beam of light from one material into another (like from air to water) it bends in such a way that the path it takes from one point to another requires the least possible time.

Taking a straight line means traveling through a lot of the “slow material”. Traveling through lots of “fast material” to make the path through the slow material as short as possible means taking a longer path overall (and taking more time). The path of “least time” is in between.

This should come across as deeply spooky.  A particle that somehow “scouts the future” and then picks the fastest path to get where it’s going seems impossible.

And to be fair: it is.  The crux of the problem is (as with damn near everything) wave/particle-ness.  Particles can’t magically know what the shortest path will be, but waves find it “accidentally” every time.

First, check out the path that the “principle of least time” carves out.  What follows is math, which some people dig.  If you skip over the block of calculations, you won’t really miss anything.

The time, T, that it takes to traverse a path is D/V, where D is the length of the path and V is the speed.

T = \frac{D_1}{V_1}+\frac{D_2}{V_2} = \frac{1}{V_1}\sqrt{a^2+x^2}+\frac{1}{V_2}\sqrt{b^2+(c-x)^2}

The picture for the derivation below.  In the top material the wave speed is “V1” and in the bottom “V2”. With a little calculus, by sliding x back and forth you can find the “minimum-time path”.

By changing x you change the path, and the amount of time T it takes to move along that path.  Calculus wonks may know that to find an “extremum” (either a maximum or a minimum), all you have to do is find the derivative, set it to zero, and solve.  With any luck, those same wonks will be forgiving if I just declare that the following derivation finds the minimum time (and not a maximum or something) instead of proving that it’s a minimum.

\begin{array}{ll}0=\frac{dT}{dx}\\\Leftrightarrow 0=\frac{d}{dx}\left[\frac{1}{V_1}\sqrt{a^2+x^2}+\frac{1}{V_2}\sqrt{b^2+(c-x)^2}\right]\\\Rightarrow 0=\frac{1}{V_1}\frac{1}{2\sqrt{a^2+x^2}}(2x)+\frac{1}{V_2}\frac{1}{2\sqrt{b^2+(c-x)^2}}(-2(c-x))\\\Leftrightarrow 0=\frac{1}{V_1}\frac{x}{\sqrt{a^2+x^2}}-\frac{1}{V_2}\frac{c-x}{\sqrt{b^2+(c-x)^2}}\\\Leftrightarrow \frac{1}{V_2}\frac{c-x}{\sqrt{b^2+(c-x)^2}}=\frac{1}{V_1}\frac{x}{\sqrt{a^2+x^2}}\\\Leftrightarrow \frac{1}{V_2}\frac{c-x}{D_2}=\frac{1}{V_1}\frac{x}{D_1}\\\Leftrightarrow \frac{1}{V_2}\sin{(\theta_2)}=\frac{1}{V_1}\sin{(\theta_1)}\end{array}

The angles for the last step above.  This is also “Snell’s law”.

The exact value of x isn’t particularly useful.  What is useful are those angles.  The statement “\frac{1}{V_2}\sin{(\theta_2)}=\frac{1}{V_1}\sin{(\theta_1)}” is Snell’s law.

Snell’s law should look familiar to anyone who’s used to talking about waves going from one material to another.  It describes, for example, the bending of light as it crosses between air and water.

So, the law of “least propagation time” is nothing more than a different, and far more difficult, way of stating “Snell’s law”.  And again, if you’re talking about a particle, it’s hard not to think that the particle is testing each path in advance, and then taking the quickest one.

However, you can derive the same result by thinking about how waves propagate.  Waves (light, sound, water, whatever) propagate perpendicular to their wave crests.  Take a second: picture an ocean wave.

As one side of the crest enters a different material it changes speed.  When different parts of the wave are traveling at different speeds the wave front as a whole changes direction.

Top: a section of the wave starts to hit the boundary between two materials.  Middle and Bottom: In the second material the wave moves slower.  Since one side of the wave is moving faster than the other the wave front “swings around” into a new direction.

A good (but not quite accurate) way to picture the situation is to think of a car where the wheels on one side spin faster than the wheels on the other.  Naturally, the car’ll turn to the side.

Left: the important angles, and where they show up in the triangles.  Right: the angles and lengths involved in the math below.

You can be a bit more exact about this.  The diagrams above describe a piece of the wave crest from the moment when one side hits the boundary, to the time the other side hits.  Call that time “T” (why not?).

The distance that the top end of the piece-of-crest travels is D1 = V1T, and the distance the bottom tip travels is D2 = V2T.  Now, using the definition of sine: \sin{(\theta_1)}=\frac{D_1}{L} and \sin{(\theta_2)}=\frac{D_2}{L}.

Combining these you get:

\begin{array}{ll}\frac{1}{D_1}\sin{(\theta_1)}=\frac{1}{L} = \frac{1}{D_2}\sin{(\theta_2)}\\\Rightarrow\frac{1}{V_1T}\sin{(\theta_1)}=\frac{1}{V_2T}\sin{(\theta_2)}\\\Rightarrow\frac{1}{V_1}\sin{(\theta_1)}=\frac{1}{V_2}\sin{(\theta_2)}\end{array}

Holy crap!  Snell’s law again!  Having the same result means that, in terms of behavior, the two approaches are indistinguishable.  So, instead of a spooky particle scouting every path looking for the quickest one, you have a wave that’s just doing it’s thing.

The principle of least time is a cool idea, and actually makes the math behind a lot of more complicated situations easier, but at it’s root is waviness.

Posted in -- By the Physicist, Equations, Geometry, Logic, Physics | 27 Comments

Q: Does light experience time?

Posted on July 31, 2011 by The Physicist

The original question was: Given that light is moving at light speed, and time slows down as a massive object approaches the speed of light, does light travel through time?  Does the whole time slowing down thing just not apply to massless particles, and if not why not?  If light doesn’t travel through time, how does anything make sense, since clearly light moves but movement is dependent on time?

Physicist: Nope!

There are some things that behave differently when investigated from an “approaching light speed” way of thinking and the “being at light speed” way of thinking.  In this case there’s no difference.  When something travels at the speed of light it really doesn’t experience any time.

On the flip side of that coin, it also doesn’t experience any distance.  The time and location of its emission and the time and location of its absorption are the same from a photon’s perspective.

This may not make sense, and it’s a little mind bending, but consider this:

Movement isn’t dependent on the time experienced by the moving thing, it’s dependent on your time.  If you see someone pass by, you can say (for example) “that person is moving at 100 kph, because during one of my hours they’ve traveled 100 of my km”.  That may seem a little over-exact, but the time and distance between things changes for observers that are moving differently, so you have to be especially careful.

If, however, you were to ask the person who passed by “how fast are you moving?” they’d say that they’re not moving at all.  They’d say that during one of their hours they traveled zero of their km.  These different measurement systems / perspectives are called “reference frames”.

Here on Earth we feel like there’s such a thing as “non-relative movement”, since we all agree (very naturally) on the same reference frame: the (local) surface of the Earth.  That is, you probably frequently refer to yourself as moving, while you rarely think of the Earth as moving.  You’d have to be pretty full of yourself to drive down the street and claim that you’re stationary and that the rest of the world is moving past you.  But at the same time: you’d be right.

Smug drivers: technically correct.

The point is: everything always thinks of itself as stationary (you don’t move with respect to yourself), and movement is a property assigned to other things based on each observer’s reference frame.  So light may not experience either time or distance itself, but to move, all it needs to do is get from one point in your spacetime to another point in your spacetime.

Answer Gravy: As a needless side-note: when physicists talk about the path of an object through spacetime they usually “parametrize” it using that object’s on-board (or “proper”) time.  That is, you give them a time on the on-board clock, and they’ll tell you where the object is at that time.

Using on-board time is convenient for a number of subtle reasons.  It even makes one of the derivations of E=MC2 run a lot smoother!

But a photon can’t have an on-board-clock, so physicists instead use an “affine parameter”, which is fancy-speak for “screw it, we’ll just use my clock”.

Posted in -- By the Physicist, Philosophical, Physics, Relativity | 120 Comments