Physicist: With very few exceptions, yes. What we normally call “random” is not truly random, but only appears so. The randomness is a reflection of our ignorance about the thing being observed, rather than something inherent to it.
For example: If you know everything about a craps table, and everything about the dice being thrown, and everything about the air around the table, then you will be able to predict the outcome.

Not actually random.
If, on the other hand, you try to predict something like the moment that a radioactive atom will radioact, then you’ll find yourself at the corner of Poo Creek and No. Einstein and many others believed that the randomness of things like radioactive decay, photons going through polarizers, and other bizarre quantum effects could be explained and predicted if only we knew the “hidden variables” involved. Not surprisingly, this became known as “hidden variable theory”, and it turns out to be wrong.
If outcomes can be determined (by hidden variables or whatever), then any experiment will have a result. More importantly, any experiment will have a result whether or not you choose to do that experiment, because the result is written into the hidden variables before the experiment is even done. Like the dice, if you know all the variables in advance, then you don’t need to do the experiment (roll the dice, turn on the accelerator, etc.). The idea that every experiment has an outcome, regardless of whether or not you choose to do that experiment is called “the reality assumption”, and it should make a lot of sense. If you flip a coin, but don’t look at it, then it’ll land either heads or tails (this is an unobserved result) and it doesn’t make any difference if you look at it or not. In this case the hidden variable is “heads” or “tails”, and it’s only hidden because you haven’t looked at it.
It took a while, but hidden variable theory was eventually disproved by John Bell, who showed that there are lots of experiments that cannot have unmeasured results. Thus the results cannot be determined ahead of time, so there are no hidden variables, and the results are truly random. That is, if it is physically and mathematically impossible to predict the results, then the results are truly, fundamentally random.
What follows is answer gravy: a description of one of the experiments that demonstrates Bell’s inequality and shows that the reality assumption is false. If you’re already satisfied that true randomness exists, then there’s no reason to read on. Here’s the experiment:
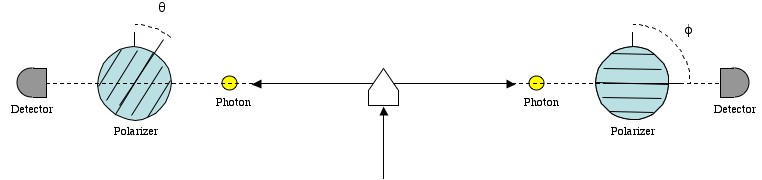
The set up: A photon is fired at a down-converter, which converts it into two entangled photons. These photons then go through polarizers that are set at two different angles. Finally, photo-detectors measure whether a photon passes through their polarizer or not.
1) Generate a pair of entangled photons (you can do this with a down converter, which splits one photon into an entangled pair of photons).
2) Fire them at two polarizers.
3) Randomly change the angle of the polarizers after the photons are emitted. This prevents information about one measurement to affect the other, since that would require that the information travels faster than light.
4) Measure both photons (do they go through the polarizers (1) or not (0)?) and record the results.
The amazing thing about entangled photons is that they always give the same result when you measure them at the same angle. Entangled particles are in fact in a single state shared between the two particles. So by making a measurement with the polarizers at different angles we can measure what one photon would do at two different angles.
It has been experimentally verified that if the polarizers are set at angles and
, then the chance that the measurements are the same is:
. This is only true for entangled photons. If they are not entangled, then
, since the results are random. Now, notice that if
and
, then
. This is because:
We can do two experiments at 0°, 22.5°, 45°, 67.5°, and 90°. The reality assumption says that the results of all of these experiments exist, but unfortunately we can only do two at a time. So C(0°, 22.5°) = C(22.5°, 45°) = C(45°, 67.5°) = C(67.5°, 90°) = cos2(22.5°) = 0.85. Now based only on this, and the reality assumption, we know that if we were to do all of these experiments (instead of only two) then:
C(0°, 22.5°) = 0.85
C(0°, 45°) ≥ C(0°, 22.5°) + C(22.5°, 45°) -1 = 0.70
C(0°, 67.5°) ≥ C(0°, 45°) + C(45°, 67.5°) -1 = 0.55
C(0°, 90°) ≥ C(0°, 67.5°) + C(67.5°, 90°) – 1 = 0.40
That is, if we could hypothetically do all of the experiments at the same time we would find that the measurement at 0° and the measurement at 90° are the same at least 40% of the time. However, we find that C(0°, 90°) = cos2(90°) = 0 (they never give the same result).
Therefore, the result of an experiment only exists if the experiment is actually done.
Therefore, you can’t predict the result of the experiment before it’s done.
Therefore, true randomness exists.
As an aside, it turns out that the absolute randomness comes from the fact that every result of every interaction is expressed in parallel universes (you can’t predict two or more mutually exclusive, yet simultaneous results). “Parallel universes” are not nearly as exciting as they sound. Things are defined to be in different universes if they can’t coexist or interact. For example: in the double slit experiment a single photon goes through two slits. These two versions of the same photon exist in different universes from their own points of view (since they are mutually exclusive), but they are in the same universe from our perspective (since we can’t tell which slit they went through, and probably don’t care). Don’t worry about it too much all at once. You gotta pace your swearing.
As another aside, Bell’s Inequality only proves that the reality assumption and locality (nothing can travel faster then light) can’t both be true. However, locality (and relativity) work perfectly, and there are almost no physicists who are willing to give it up.







Your gravy is lumpy. Don’t use so much flour.
The answer did not include examples of chaos (nonlinear dynamics) which, while technically deterministic, provide ‘random’ results regardless of how well initial conditions are known.
Still, nice answer, especially with regard to quantum theory.
I think the swearing at Bohm is uncalled for. Skeptics of a standard paradigm should exist and continually be looking for a break in the standard paradigm. Also, I believe Bohm did most of his very original and interesting work before Bell derived his inequalities. If being wrong is a crime then most theorists would be in the klink.
Thanks kindly, and fair enough. In a chaotic system the predictions do get better, the better your measurements are (reducible randomness). The irreducible component comes in when your measurements run up against Heisenberg uncertainty, or when the evolution of your system is (eventually) overwhelmed by quantum effects. But this is just another way of talking about the quantum randomness in the post.
I mostly think that Bohm is an ass based on his stubbornness and his personal creepiness. He’s only a criminal according to McCarthy.
I think a more useful way to talk about randomness is “uncertainty”. There are different kinds of uncertainty, and thus different kinds of randomness. Uncertainty about air currents and temperature and the exact velocity of coins and dice are what make those things random. Under controlled conditions, that uncertainty can be eliminated, and so those things aren’t *always* random. A lot of binary quantum events (like polarizers) are more about indexical uncertainty (from the perspective of MWI)–the uncertainty we have about what part of the universe we’re in. So “uncertainty” doesn’t work to explain quantum randomness in the Copenhagen interpretation.
I think this is a pretty good way to explain it to a general audience.
Pingback: Q: What is the meaning of the term “random”? Can thinking affect the future? « Ask a Mathematician / Ask a Physicist
Uncertainty and randomness are not the same thing. Probablistic events have uncertainty associated with them e.g.: No one can predict exactly when a specific radioactive nuclide will decay (uncertainty) but the decay of these nuclides is a strictly governed probablistic function not a random one. There’s exactly a 50% chance it will decay within it’s half-life period for example.
A random event can’t be assigned any probability. A truly random event wouldn’t have any greater or lesser chance of occurring by any parameter (time, conditions, interactions etc).
I don’t know of any natural process that’s truly random (unbounded and completely indeterminate).
Don’t expect to find one!
It’s not possible to create a function (probability distribution or otherwise) that is uniform over all numbers. If the definition of random is: “absolutely no tendency to happen more at any time or place”, then nothing can be random.
Well, boy I feel dumb here:
“It’s not possible to create a function…that is uniform over all numbers”
but isn’t f(x) = 5 uniform over all values of x — all numbers? I agree for probability distributions because uniform probabilities in a distribution would approach 0 as the number of outcomes approached infinity, but *any* function? Am I not understanding the term “uniform” ?
That’s my bad. You’re exactly right. Your understanding of “uniform” is perfect.
I’ve been spending too much time only considering L1 functions.
Hi, I think this demonstration has multiple issues.
1. To quote Wikipedia, “Experimental results have demonstrated that effects due to entanglement travel at least thousands of times faster than the speed of light.” This conflicts with the presumption made in step 3.
2. The impossible chance of 40% only exists when you do impossible experiments.
3. To prove to cause a random effect in the real world, one must affect deterministic objects and change their ways randomly. Otherwise the world is perfectly deterministic with some state-unknown particles. And when these particles come in contact with the world, all results and therefore the world are deterministic, just like what happens in the experiment.
1. I was a little surprised to read that in Wikipedia. It’s at best misleading and at worst wrong. Entanglement is a fancy form of correlation, so if you’d like to say that the fact that two coins are already in the same state is an “effect that travels” then it definitely travels infinitely fast.
2. The 40% thing is just a tool to emphasize that the correlation found in entanglement experiments is classically impossible. It can’t happen if the particles are in a particular state.
3. The crux of Bell’s theorem is that a probability distribution for a thing being in a single (even unknown), state can’t exist. That is, you can show that it doesn’t make sense to ask “what is the probability that this particle is in state 1?” for a particle in an entangled pair. There’s a better proof of that here.
You could make the argument that the universe is deterministic, but only from a fairly weird point of view.
What I meant in 3 is: If one small universe only consists of entangled photons and fixed polarizers, in this case you “know everything about” the universe, and you can predict the chances. So there’s no randomness (i.e. unpredictability) in the universe when you “know everything about” it. No need to introduce multiverse.
The statistics of entangled particles and the idea of “one universe” (a universe with one state) are inconsistent. The “multiverse” is just a way of talking about a universe with many states (both large and small scale). The language here is a little weak: we need more words than just “universe”.
Even if you know everything about a state, the best you can do is find the probability of measuring it in a particular (different) state. For example, if I know that a photon is definitely diagonally polarized, then I can say that the chance of it being measured in a horizontal state is 50%. But, unfortunately, there’s no way to bump that to 0% or 100%.
I didn’t read through the whole article just skimmed bits but I did pick up one part where the experiment is described.
3) Randomly change the angle of the polarizers after the photons are emitted.
But how do you randomly change the angle of the polarizers. In the future Scientists may be able to scan your brain and again take in all other atmospheric/physical variables and predict exactly where you will move the polarizer too. So it’s not truly random, only random if you believe that you are moving the polarizer to a random location. My argument may be a bit off here as I didn’t read that much into everything.
I don’t believe there is such a thing as true randomness. Only at this point in time it may appear as though some things are. If we had any true randomness then i think the universe would collapse from a knock-on-effect.
Any and every manner by which you determine how to randomly orient the polarizers works. This is an addressed concern.
That said, you have a point. It could be that, somehow, the universe is conspiring against us at every turn to make it seem as though things are random. That is, it may be that all of the results of the experiment that demonstrate the fundamental randomness of things may have been written in the stars (so to speak) at the beginning of time. However, predictions based on the quantum mechanics (which involves, among other things, fundamental randomness) seem to hold up.
Fate or not!
This blew my mind. Before reading this, I thought there was always a slight chance that one answer of another would come up. Damn, was I wrong!
I’m not a physicist, but reading this makes me question things:
1) The Reality Assumption implies choice, which has inherent randomness in it, which results in it being disproven. Choosing to do the test or not as well as choosing to look at the results or not. Why is the choice of the tester not included in this experiment? If the tester actually “chose” then there is randomness, regardless of the test outcome. Instead, wouldn’t the particles that make up the state of the tester and the experiment also part of the experiment? Deciding to run that test seems like it should be the experiment, not the experiment itself.
2) “However, predictions based on the quantum mechanics (which involves, among other things, fundamental randomness) seem to hold up.” – We are a part of the system and therefore interact with it. The randomness is caused by our measuring it and interacting with it, which may indeed be no random choice to do so. Wouldn’t it be safer to say “We do not have the capacity to measure and record all required variables without influencing them, and therefore must account for the apparent randomness our lack of information provides” ? The absence of data does not imply absence of data.
Pingback: Q: Is quantum randomness ever large enough to be noticed? | Ask a Mathematician / Ask a Physicist
After 20 years of studying the chaos theory I am deeply convinced that true randomness and stohasticity do not exist in our universe. Common sense dictates that in causal universe it is not possible. What appears to be random/stohastic is only a very very complex behaviour of a non-linear system. Classical example is coin flipping. It is not random at all – it is just a nonlinear system with large movements consisting of levers made of muscles, tendons and bones, i.e. a classical chaotic sytem, but deteministic.
Hey , i need to understand this experiment for a schoo project, could someone please tell me what
a
b
c
C
represent?
Thanks!
“That is, if it is physically and mathematically impossible to predict the results, then the results are truly, fundamentally random.”
The idea behind hidden variable theory is that everything is causal, even if we yet fail to understand how. Indeed, the march of time has shown that every single phenomenon we previously thought to be random was actually causal.
If something is mathematically impossible to predict, that doesn’t logically mean that it is not the result of causal interactions that we cannot yet know (which is what Einstein was trying to say in the first place).
For all its complexity, this equation by Bell fails simple logical analysis. What is the reality he is proposing exactly, that in certain situations, things suddenly occur with no relation to the rest of the universe? To me, all he seems to prove is that it is not possible to know everything, which really has nothing to do with whether everything that occurs is causal or not.
Here’s the one reason I am not inclined to believe that true randomness exists:
If true randomness existed at the quantum level, how could anything beyond the quantum level be deterministic? If things were truly random, we would never be able to predict anything. And yet, I can predict where a ball will be when it is thrown, I can predict the time it will take to bring an of a given size to temperature T given other information.
If the microuniverse isn’t deterministic, then the macrouniverse would be just as random.
Beautiful point!
But as unintuitive as it is, while individual things may be random, an aggregate of them can be pretty predictable (when taken together), which is exactly what the macrouniverse is. There’s even an old post that talks about this.
It seems to me that there is something wrong with this argument. When you say “the chance the measurements of the entagled photons are the same”, it seems equivalent to “the chance the two polarizers are less than 90 degrees apart”. You then calculate a positive value for C(0,90), which is clearly wrong, therefore some premise is wrong, therefore rejcet the reality assumption. I’d be more inclined to reject C(a,c) >= x+y-1, which doesn’t seem to follow from the argument you presented. Also, this argument doesn’t seem to require any experimental evidence, it’s just a mathematical derivation. What did I miss?
Having the polarizers less than 90° apart doesn’t mean that they’ll measure the same result. The experimental evidence is what lead to the fact that , which includes the fact that orthogonal polarizers always yield opposite results (
, which includes the fact that orthogonal polarizers always yield opposite results ( ). The
). The  is mathematically derivable (derived in the post in fact!).
is mathematically derivable (derived in the post in fact!).
There’s another post that talks about this experiment in a bit more detail here.
I’m still missing something. Suppose I have a square with area = 1, and I throw darts at it so they are uniformly distributed. Then for any two numbers a and b that are both between 0 and 90 (degrees), suppose I mark a sub-square whose sides have length cos(b-a). The sub-square has area cos^2(b-a). Define C(a,b) as the probability that a dart lands in this sub-square, so C(a,b) = cos^2(b-a). Doesn’t this satisfy all the premises of this argument? That is, we should have C(0,45) >= C(0,22.5) + C(22.5,45) -1, but in fact we have C(0,45) = 0.5 while C(0,22.5) + C(22.5,45) -1 = 0.7. That’s a contradiction. We didn’t have to experiment with entangled photons, or assume counterfactual definiteness … ?
What you’ve rediscovered is that C(a,b) = cos^2(b-a) is a classically impossible correlation. That is, there is no simple probability distribution on the two particles that supports it, in much the same way that there’s no probability distribution on the two coordinates for where the darts hit. And yet, when we actually do the experiments on entangled pairs this is exactly the correlation we always get.
That’s why counterfactual definiteness is dropped; entangled photons can’t be described in terms of being in a particular state, the way a dart must be in a particular state, even if that state is unknown!
It seems the proof of the randomness of the universe is in the quantum entaglement phenomenon, right? Because for other ordinary quantum phenomena the appearent randomness comes from the unertainty principle that precludes the exact knowledge of each variable in the mathematical model. Basically each ordinary particle is in a state that we can never measure precisely hence the description as a super position of states. Whereas for entagled particles there is no precise state as if the pair hops randomly from one possible state to another in an actual super position of states. Does this interpretation makes sense?
I thought entangled photons were polarised at 90 degrees to each other? Wouldn’t that mean C(0°, 90°) = 1?
The gravy experiment can be easily disproved with some logic. It is called a circular argument, and you stated that you had to change the angle of both polarizers to two RANDOM angles, and this proves randomness in the end.
An example: say I have a box. I can prove I have a box because I have an object and the object is the box. You are proving something with the same thing you are trying to prove, and that doesn’t work out, no matter how much math to stuff in there.
A typical example of randomness and stochasticity is coin flipping by hand and thumb.
But, this is, actually a non-linear system of levers (bones) and tendons and muscles with large (like pendulum) displacements and therefore it can behave chaotically. It is per se very sensitive to initial conditions. Hence, in my mind, it is actually a truly deterministic chaotic system. I am 100% convinced that true randomness cannot exist in a causal universe, as randomness inherently assume that the things are happening for no apparent reason!
If there is no randomness… then I don’t belive life can exist.. then we are just a complex system .. what is a will it i belive is a substantial example of randomness..
If will do not exist then life is just acomplex robot.
Another way of looking at it, is that indeed everything in the end is causal, but every possible causal line of events will happen on a grander scale, in a giant network. We just cannot know ahead which nodes in the network seem be to be experienced by us (our local universe/time line), because of the lack of hidden variables to tell us so (a concept which after 60 years still holds up).
Using the same logic, there are then instances of ourselves in other universes/time lines that also undergo all other causal nodes. From that vantage point, arguing about randomness seems a moot point, because, which one is the “real you”?
Any thoughts?
Could it not be said that what we label as random are merely phenomena for which we don’t yet understand all of the laws which govern their behavior? Therefore, they may be regarded as random from a practical standpoint insofar as our ability to predict behavior, but not truly random as their behavior is in fact governed by properties and laws which we have yet to discover.
To say that something is truly random would be to say that its behavior is not subject to physical law.
Excellent comment!
In a universe that we comprehend today, there can be no true randomness as it is not subject to physical law, i.e. things would happen for no reason. If there is a reason for an event then it is not truly random as the result can be predicted, as a consequence, all in accordance with physical laws. If it cannot be predicted then it is not subject to physical laws as we know them hence it cannot exist in our universe.
If you don’t believe that the best theory we have for how things exist at the quantum scale predicts true randomness than you have misunderstood the argument. If you can setup an experiment based on quantum probability such as the decay of a uranium atom then you cannot predict when that will happen with a certainty of 100% regardless of how much you know about the universe. The inability of 100% prediction does not come from a lack of instruments or accuracy. Just as the world is round and the sun shines, these are observations with a testable outcomes that fit the model. We cant measure or predict with any certainty because the certainty does not exist at any level.
If on the other hand we were to take a thousand or maybe a million particles, measure their decay and plot the times on a graph we will see that there is a ‘tendency’ for a lot of the times to occur close to a specific value. In a bell curve possibly. This still highlights the fact that you can predict where most of them will end up, but not all. And the not all is the key here because whilst the chances may be high of the time being in the middle of the curve, there is still no way that you can look at an individual particle and predict its decay with 100% accuracy. I will restate that: it is impossible to accurately predict the outcome and the impossibility is an inherent part of the universe and provides for a model that represents experimental outcome at a level where no other model can.
The outcome we see in a bounced basketball is because there are so many particles and most of them will be close to the centre of the bell curve in terms of measured position or velocity. This means that any particles dramatically removed from the centre of the curve are so few that there effect is practically unmeasurable. How ever this does not mean that there is absolutely no spooky quantumm stuff happening in your basketball. It is simply swamped by the probability curve.
okay, some of this was good and all , and thanks for that . but , true randomness does not exist except for in our minds . the term “random” is quintessentially a misnomer . how is that not completely obvious ?
Your “proof” is utterly specious. By confusing measurements with angles you invalidly apply to the correlation function a well-known mathematical inequality and unsurprisingly obtain a contradiction, then blame it on the assumption that more than two experiments can be performed. When Jim Nazium demonstrates that the inequality fails to hold, and hence the contradiction follows, even in the case of just two experiments (with angular differences of 22.5 and 45 degrees), you blame this on the correlation being “classically impossible,” yet there was no assumption of “classicality” and his objection holds in the non-classical case, so perhaps you’ve disproved the negation of the reality assumption instead.
Bell’s theorem depends on the assumption of free will.
You may find interesting to read these two posts by G. t’ Hooft, as well as his papers.
http://physics.stackexchange.com/questions/34217/why-do-people-categorically-dismiss-some-simple-quantum-models
http://physics.stackexchange.com/questions/30065/why-do-people-rule-out-local-hidden-variables
Pingback: Random observations | Peoria Pundit
I like the clarity of your explanation of the reality hypothesis. Unfortunately, your overall conclusion is misleading. You say that the test of Bell’s Theorem shows the reality hypothesis to be false and then, as a footnote, point out that locality and realism can’t both be true and that the majority choose locality. OK, let’s be forthright about it. I choose reality and a good argument can be made that relativity is compatible with both. Incidentally, the usual narrative is that Hidden Variables was demonstrated to be wrong by the experiment. In fact, Bohm’s non-local Hidden Variable theory, formulated long before the experiment was done, was vindicated right along with the usual interpretation of quantum theory.
A word about Bohm. All too many physicists have failed to examine the implications of quantum theory and Bohm grappled with it, first writing a book supporting the usual probabilistic interpretation, and then developing a mathematically consistent hidden variable theory when many argued it couldn’t be done. Like Einstein and others, he did not like the rank empiricism of quantum theory and for good reason. While almost the whole physics community considered EPR an empty philosophical question, it was literally the work of 3 guys, Einstein, Bohm and Bell, all fierce critics of the usual interpretation, that actually interrogated the issue – first raising the question and then coming up with a theorem, which lead to the understanding of entanglement we have now. Finally, big props for Bohm for refusing to testify before McCarthy. The creeps are those inside and outside the physics community who did.
Bouncing basket ball is an archetypal example of a DETERMINISTIC CHAOTIC SYSTEM!
I strongly support Jason’s excellent comment that true randomness can only exists in our minds and can be, as such, treated with abstract math (mathematical statistics) but this math DOES NOT EXPLAIN REALITY. This does not tell WHY!
To me, cosmos/universe is only an infinitely complex phenomenon, but deterministic. Complexity grows infinitely if not stopped by some external force but it always remain deterministic. After Big Bang, at which cosmos was a simple point, cosmos expanded (as we see now CHAOTICALLY) and is still expanding at an accelerated rate. Seemingly, nothing to stop its ever growing complexity and eventually, the birth of life (and intelligence) as its most complex form. But it seems that the dark energy would prevail, it is the force that will simplify the ever growing complexity by an eternal accelerated expansion and eventually lead us all into simple big freeze.
Things that come out of the blue for no apparent reason or cause (i.e. randomly) could not be subject to physical laws, but we see that cosmos was.
There can be many hidden physical variables that could affect a “random” experiment.
Doing the same experimente, some of us pay attention an know some conditions and ignore others.
My first impression is that we often know few compared to what we should to be 99% confidente of an outcome.
Probability calculus helps to test trials and define the chance to be certain of a statement.
I was looking for an authoritative but readable article on randomness to link to on my family-friendly blog. This one fits the bill – with one small but crucial exception: the profanity in paragraph 3. Would appreciate it if you would edit with a family-friendly synonym. Thanks.
Thank you!
Pingback: There Is No Such Thing as Random Chance | summa politica
Well let me ask another question. Is it possible to “prove” that a random number is “truly random”?
Bravo! Randomness would be a free lunch a there is no such thing! A happening for no apparent reason!
Cagin, is it possible to correctly predict the outcome of a quantum event with 100% accuracy?
If a number is truly random then that individual outcome should be impossible to “prove”. That is the nature of a “random” event. You cant disprove it and saying that you cannot prove it is random means you cant prove that it is non-random.
Slobodan, how is randomness a free lunch? And in fact the universe we currently see may in fact be some kind of gigantic free lunch!
Forgive me, I’m not a physicist, but I do have a problem with the concept of cause and effect, if every effect has a cause , then what conditions were in place to cause the big bang? , I’m just curious. That is all.