Physicist: Hells yeah.
Fastest fast: This is worth commenting on since you often hear “nothing can travel faster then light”, but the justification is almost always missing. The universe seems to be pretty happy thinking of the speed of light as being the same to everybody first (Maxwell’s Laws give you the speed of light, but Maxwell’s laws are the same to everybody so the speed of light is the same to everybody), and as a speed limit second. Since you always see light moving at the same speed, then no matter how much you speed up, it will always pass you by. So catching up to it isn’t an option, and everyone will always see you traveling slower than the speed of light.
Densest dense: The harder you compress something, the denser it becomes. Normally this is reflected in the distance between atoms shrinking. However, if the pressure is great enough, the atoms will find that it’s easier to have their electrons merge with their protons which then turn into neutrons (and also spit out neutrinos, but whatever). Without battling electron shells, the once mostly-empty atoms can be packed nucleus-to-nucleus. Pressures and densities this high only seem to show up in neutron stars (guess where the name comes from). By way of comparison, here are some densities (in kilograms per liter): Air = 0.0012, People = 1, the Sun = 1.4, Iron = 7.8, Gold = 19.3, Neutron Star = 500,000,000,000,000.
You can also cheat a little. If a neutron star has a mass of more than about 5 Suns it will collapse into a blackhole, which is technically more dense.
Coldest cold: You might have guessed: zero. Specifically 0°K = -273°C = -460°F. However, this is more of an “asymptotic limit” and can never quite be reached. An object with a temperature of absolute zero will have no atomic movement (heat) whatsoever, but that’s not possible. One way of thinking about it is in terms of the Heisenberg uncertainty principle which, in a paraphrased nutshell, states: “You can’t have both a perfectly certain position and a perfectly certain momentum”. Where 
So if you’ve got a substance and you have any idea where it is (
Despite the difficulties imposed by the uncertainty principle, we can still get things crazy cold. The world record for lowest temperature now stands at 0.0000000001°K = 0.1 nK.
Hottest hot: There are actually two limits here, depending on how you phrase the question. The first is the theoretical upper limit, which depends on which theory you’re working with, but is often quoted around 1030 °K. These limits have to do with “the graininess” of space, and how much energy can be forced into a particular region.
The second kind of limit is more practical. As a gas is heated its atoms move faster and faster. When they collide they bounce off of each other and often create photons (light), which generally just go on to push other atoms around. However, as the temperature approaches about 4 billion °C, the atoms of the gas will often have enough energy to create electron/positron pairs (“E=mc2“, where “E” is the kinetic energy of the gas atoms, and “m” is the total mass of the electron/positron pair). Normally these newly created particles will almost immediately find other electrons and positrons and annihilate, creating light. But sometimes they’ll create neutrinos instead of light. Neutrinos are “weakly interacting” (which is science speak for “goes through walls, no problem”), so the energy used to create them just flies into space, never to be seen again (or just about never). This has the effect that a gas with a temperature above around 4,000,000,000°C will cool off on its own (without seeming to radiate any energy). For comparison, the core temperature of the Sun is about 15.7 million °C.
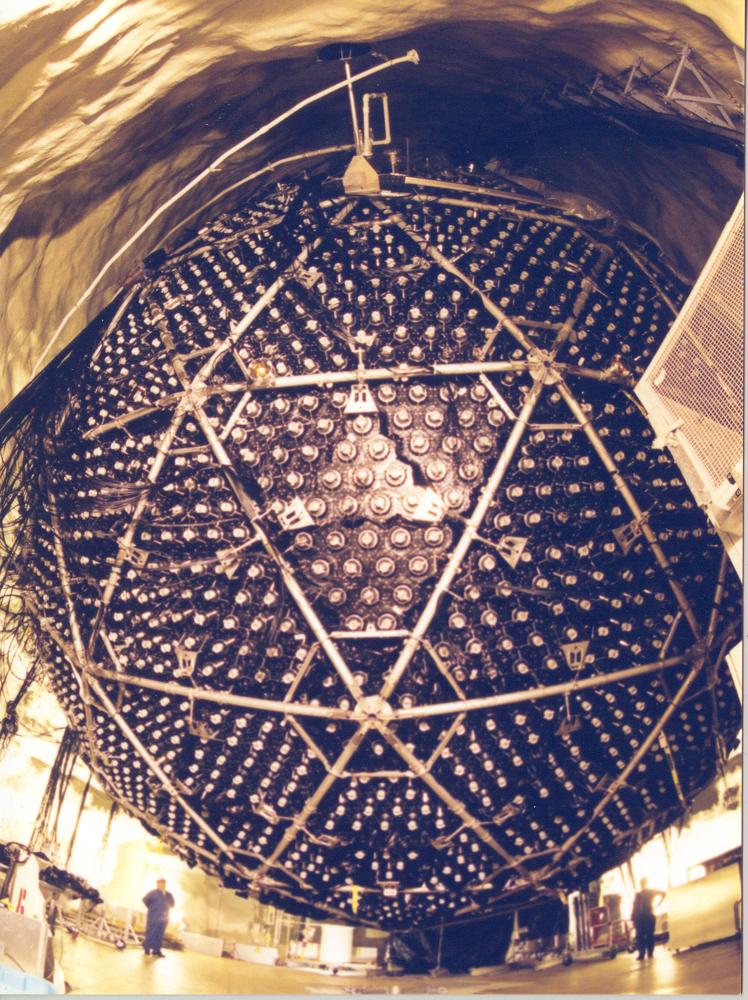
The Sudbury neutrino detector: 40 feet across, and among the more evil looking things ever built. Image stolen without remorse from "http://zuserver2.star.ucl.ac.uk/~idh/apod/ap990623.html"
This is sometimes important during stellar collapse. If a star needs to have a core temperature above the cut-off point to hold itself up, then it’s not going to hold itself up.
Smallest small: Again, for “uncertainty principle type reasons” it doesn’t make sense to talk about objects or events smaller than the Planck scale, which is about 10-35m. So far, nobody can think of anything in the universe, at any scale, that would really care, or be able to tell the difference between two points separated by 10-35m.
Emptiest empty: One version of the Heisenberg uncertainty principle can be written “
So even with a perfect vacuum, you’ll still have crap around. This crap is often called the “vacuum energy” or “zero point energy”.
One of the few examples of a device that can harness the vacuum energy of the universe to charge your chrystals or whatever. This illustration of "pyramid power" stolen from "http://www.merlinsrealm.com/pyramid-power.htm"
Sadly, harvesting the vacuum energy is physically impossible (it would violate the uncertainty principle). The vacuum energy amounts to about 10-13J/m3, or about “the energy a baseball has falling off a table per volume of Lake Superior“.
I love it. Concentric triangles (three of them) can store vacuum energy. Hilarious. What do you bet Bill Mahr has one of those?
It’s great that the answer to my question is so complete and informative, and also has a reference to my hometown. Thanks!
Is there a fastest acceleration?
Do the field equations have a maximum curvature? I suppose you could just take the speed of light and divide by one plank unit of time…
Cool Website!
if over very small scales particles can pop in and out of existence, does that mean at any given point in space-time, the probability of a bowl of petunias poping into and then out of existence is non-zero?
Yes…
It doesn’t follow immediately, but the probability isn’t zero. For all practical purposes though, it is zero. You could “run the universe” over and over a ridiculous number of times and never see that happen anywhere.
well, i guess really two bowls would appear. a bowl of petunias and a bowl of anti petunias.
Actually, a bowl of petunias and a very surprised looking whale should satisfy the balance….lol
what is the limit of strength of ideal metal/alloy or any theoretical material that could be achieved on earth?
In the section on”densest dense” you claimed that neutrinos get spit out.
In the book “The Character of Physical Law” Richard Feynman claims it spits out anti-neutrinos , which are clearly different. Who is right?
Hey,
I’m just casually browsing through your old posts so this might seem a bit pedantic, but for the sake of scientific rigor: You don’t use the degree-symbol when refering to temperature measured in Kelvin, so technically -273 °C are about 0 K.
Thanks though for the (almost) always enlightning posts (despite the sometimes really blatant overestimation of the many worlds theory, which in my humble opinion does not explain or solve but rather shift any problems the copenhagen interpretation raises, but alais, that’s how opinions on non-testable theories work 🙂
Best wishes and regards,
Gregor
“In the section on”densest dense” you claimed that neutrinos get spit out.
In the book “The Character of Physical Law” Richard Feynman claims it spits out anti-neutrinos , which are clearly different. Who is right?”
neutrinos according to this http://en.wikipedia.org/wiki/Electron_capture
proton + electron -> neutron + electron neutrino
you may be confusing antineutrinos from beta- decay:
neutron -> proton + electron + electron anti-neutrino
that aside, i am curious if there is a shortest short in duration – even wavefunction collapse and molecular interactions take time.
also, is it possible for there to be frequencies so low the wavelength would exceed the observable universe?
@Xerenarcy
It’s likely that Feynmann and I are talking about different things (electron capture definitely creates neutrinos).
“Shortest duration” is a little tricky. We’re making attosecond laser pulses (an attosecond is to a second as a second is to the age of the universe), but that’s still not technically the shortest possible duration possible. The uncertainty principle gives us limits, but only in pairs. So there’s no limit on time, but there are limits on certain combinations of time and energy (that’s a little hand-wavy).
It’s more useful to say that a frequency so low that the wavelength is greater than the size of the universe is undetectable, rather than to say that it can’t exist at all.
@Physicist
i know this is rather tedious but what about near-field detection? a naive read of near-field physics seems to suggest a half-wavelength antenna is sufficient. in other words, an antenna spanning the entire universe could pick up near-field signals of twice the wavelength, could it not?
with regard to shortest time, intuitively plank time comes to mind (10^-43 s approx from memory) – time it takes for light to cross one plank length (fastest speed, shortest distance, therefore shortest time). by the same argument as longest wavelength being meaningless since we can’t detect it, a time shorter than plank time doesn’t come to mind.
Pingback: هل توجد حدود فيزيائية أخرى في الكون بخلاف سرعة الضوء؟
I’m a Mathematician and I have big problems understanding a world with limits.
Let’s assume I pointed with my hand in some way: how can it be that there’s a limit for the line made out of my arm. I know there might be somewhere where there’s nothing more, but spatially and theoretically, it feels like it should be infinite.
The same for the shortest length. What is it like in the half blanck length? I mean as long as I can imagine a length that’s shorter than the blanck length, who cares then about what there is between!?
Pingback: هل توجد حدود فيزيائية أخرى في الكون بخلاف سرعة الضوء؟ – Eslam Maher
This is a good read I love the different articles I hope among the email list
If something were cooled to absolute zero, wouldn’t it just vanish? You would have no method to determine its existence.