The original question was: I have a question about the twin paradox. Is it true that faster aging of the twin who stayed at home happens only when the other twin’s spaceship is accelerating/deceleration (btw, does it matter whether he is accelerating or decelerating?)? Consequently, do they age at the same rate when the spaceship moves inertially?
Physicist: The very short answer is: geometry works different in spacetime than it does in just space.
The twin paradox is a result from special relativity that states that if one person, Alice, remains “stationary” and another person, Bob, takes any kind of round trip, then the stationary Alice will experience more time. The twin paradox isn’t a paradox at all, it’s just strange and off-putting (like twins).
In relativity (that is to say: “in reality”) there’s no difference between being stationary and having smooth (non-accelerating) movement. On the surface of it, the only difference between Alice and Bob is that, in order to return home, Bob has to accelerate (turn around) at some point. So is acceleration the secret to the twin paradox? Nope.
In all of the pictures that follow the “time direction” is up, and one of the (three) space directions is left/right.
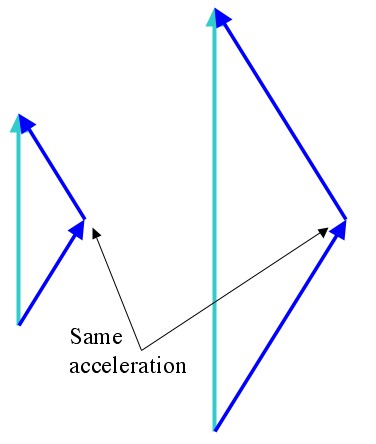
Out and back: In both situations Bob (blue) experiences the same acceleration, while Alice (aqua) sits around on Earth. The only difference is that the second situation involves twice the distance, and twice the difference in experienced time. Acceleration is not what’s important.
The trick is: spacetime doesn’t obey the “triangle inequality”. As a result, the bendier a path is, the shorter it is (that shouldn’t make any sense, so please read on).
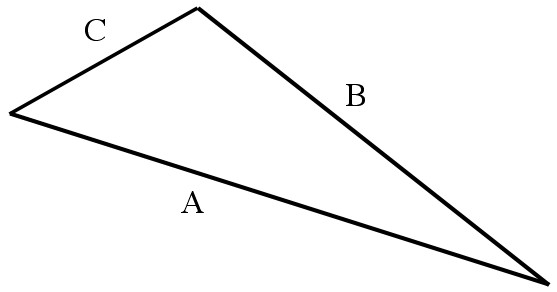
The triangle inequality says that (in space) the sum of any two sides of a triangle is greater than or equal to the third side. In spacetime the inequality can be reversed. One side is often longer than the other two: a round-about route is shorter than the direct route. In this case, A+C<B.
The equation for distance that we’re used to is: (this is just the Pythagorean theorem). But you find that when you start involving time and movement, this isn’t a particularly good measure of the distance between two points. Specifically, it’s different for different observers because of length contraction.
It so happens that the effects of length contraction and time dilation cancel each other perfectly, so that we can use a new (better) measure for spacetime distance, called the “Interval” or “spacetime interval” or “Lorentz interval”:
(as often as not the sign on the right hand side is reversed, not to worry)
The advantage to the Interval is that, no matter what, the Interval between any two points in spacetime (two locations and times) is always the same, despite relativistic weirdness. Here’s another bonus! The Interval of a path is the same as the amount of time experienced on that path!
No one every really feels like their own position is changing, so:
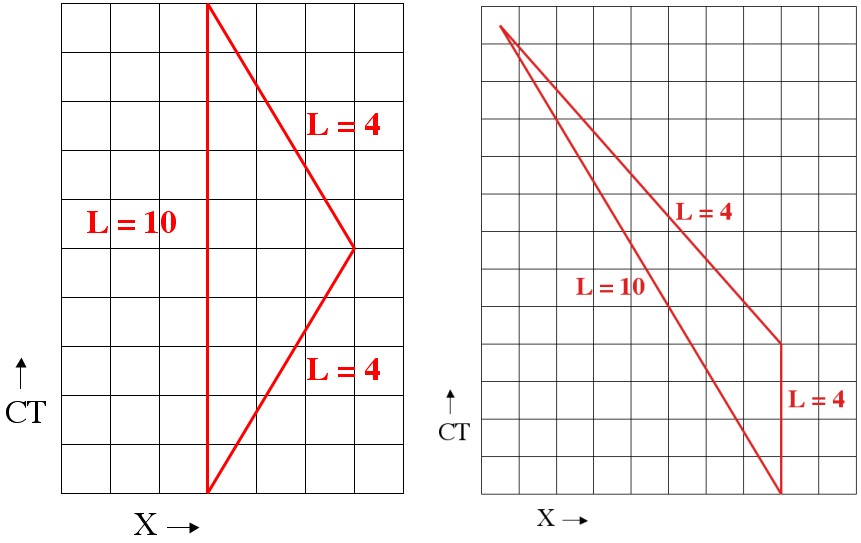
Now all that’s left is to draw a picture and do a little calculating. Here’s an example situation from Alice’s perspective, and then Bob’s (initial) perspective. The difference between Alice and Bob’s velocity is 0.6C (60% of light speed).







Pingback: Q: According to relativity, two moving observers always see the other moving through time slower. Isn’t that a contradiction? Doesn’t one have to be faster? « Ask a Mathematician / Ask a Physicist
I still don’t quite follow why the situation isn’t symmetrical.
Why can we say that Alice is sitting still (moving only through time) and Bob is taking a round trip, but we can’t say that Bob is sitting still and Alice is moving? Is it because Bob changes velocity? But it’s not the acceleration that’s important, it’s just that he’s had two different velocities?
Regardless of your perspective, Alice is always moving in a straight line, and Bob is always moving on a crooked path. As a result of the “messed up triangle inequality” all crooked paths are shorter than straight paths (through spacetime). That’s the essential difference.
Another (clearer) way to say that is Bob has two velocities.
Bob has two velocities, that if we say Alice is sitting still on earth, while Bob is moving away.
But from Bob’s perspective, he is sitting still (on the rocket), while Alice is moving away from him with a speed of 0.6c.
Pingback: Q: How can wormholes be used for time travel? | Ask a Mathematician / Ask a Physicist
Rani, I think that if you accelerate, you can’t treat your frame of reference as inertial. We cannot treat Bob as still and Alice on Earth as accelerating and decelerating, because there is a force (the rocket jet) affecting Bob’s frame of reference. I stand to be corrected but i think that at constant velocities you can apply either frame of reference, but not when one body experiences any kind of acceleration.
@Anthony Rose
That sounds exactly right! I tried to cover that idea with the last picture in the post, but it’s not terribly clear.
Pingback: Q: Is it possible to experience different rates of time? If time were to speed up, slow down, or stop, what would you experience? | Ask a Mathematician / Ask a Physicist
If Bob leaves Earth and travels to some point in space, he will have aged slower than Alice who stayed on Earth, but if Bob stays at that point and Alice leaves Earth and joins Bob, will they both have aged the same amount of time?
Yup. Weirdly, it’s impossible to objectively say which has aged more or less until they’re brought back together.
Are you aware of any other credible explanations for the Twin Paradox?
I don’t believe the Twin Paradox is solved by determining which twin accelerated (non-inertial frame) or which twin changed inertial reference frames. I can provide a thought experiment where the twin on Earth (who neither accelerates or changes inertial frames) ages slower than the twin in a spaceship (who does accelerate and/or change inertial frames). No tricks.
These two explanations appear to be ubiquitous. I am only looking for “mainstream worthy” credible explanations, not quack explanations such as “special relativity is not real” or some variety of Mach’s principle.
My understanding of the twin paradox is that the uniform accelerating motion of the traveiling twin plays a big role in why the principles of special relativity and this motion cause time for the traveling twin to pass slower than before this motion but, beause this accelerating motion it not the motion of the stay-at-home twin, it causes no slowing of his time.
My questions:
1) Is the above understtanding correct?
2) Does the relativity principle of special relativity function through this uniform motion because the motion keeps slipping from one uniform moving frame to another and thus, this motion cfan be considered uniform as demanded by this principle?
3) Would the relativity prinicple of special relativity function through this accelerating motion if it were rough, erratic, and swerving from side to side?
Thanks for your help
John
Einstein claims that the laws of physics apply equally to all observers in any inertial field (at rest or moving at a constant speed). He also claims that there is no preferential inertial field. That is Alice can say she is stationary and Bob is moving and Bob can say he is stationary and Alice is moving. In this situation they are both correct and you can not arbitrarily pick one view and say this is the correct view.
From an example above:
“Why can we say that Alice is sitting still (moving only through time) and Bob is taking a round trip, but we can’t say that Bob is sitting still and Alice is moving? ”
Sure you can, Einstein stated it. Either Alice or Bob can claim they are sitting still and the other is moving. Otherwise you have a preferential inertial field and Einstein says you can’t have that.
I think the issue here is Reality vs Relativity. Everyone knows that the rocket ship is what takes off and travels around and returns. But according to Einstein either observer can claim they are sitting still so as weird as it sounds Bob in the rocket ship can claim he is sitting still and the earth is moving away traveling at close to the speed of light and then returns. Although this sounds ridiculous, it is within the postulates of special relativity.
I believe too many people only take one observers view and then claim things like time dilation, length contraction, time running slow etc. They fail to also consider the view from the other observer who also claims that he is sitting still and it is the other that is moving.
The bottom line is that both Alice and Bob claim they are sitting still and the other is moving. When they reunite at the end of the trip they will both sway the other has aged less. So basically they are both exactly the same as before the trip started.
The theory of relativity only means something if taken and observed from one observers view. You have to use both views.
Concering the twins, Alice and Bob, upon being reuinted aftter the motion between them, you wrote: “The bottom line is that both Alice and Bob claim they are sitting still and the other is moving. When they reunite at the end of the trip they will both say the other has aged less. So basically they are both exactly the same as before the trip started..” However, acccording to Einstein in chapter 4 of ‘On the Electrodynamics of Moving Bodies’, when the traveling twin (clock A) returns to the stay-at-home twin (clock B), the twins no longer have the same age (the clocks are no longer synchronied) but the traveling twin is younger than the stay-at-home twin (the traveling clock lags behind the stay-at-home clock). It seems to me that you are disagreeing with Einstein. John
Actually I am not disagreeing with Einstein. From a preferential inertial field view, if “Twin A traveling and Twin B stays at home at home” then Einsteins prediction would hold.
However Einstein also claims that there is no preferential inertial field. That means either A or B can claim to be stationary and the other traveling.
traveling twin (clock A) returns to the stay-at-home twin (clock B)
traveling twin (clock B) returns to the stay-at-home twin (clock A)
Both scenarios are equally valid. Which one would choose? Either one you choose is a preferential inertial field view.
Imagine both twins waking up in outer space. There are no stars, planets or anything else around. What each twin sees is the other passing by. Which one is stationary and which one is moving? How would you pick one and then try to prove that you are correct?
What people don’t seem to understand is that both views exist at the same time, whatever happens to one happens to the other as well. The only way Einsteins theory works is if you pick a preferential view: “A is stationary and B is traveling” or “B is stationary and A is traveling”. But Einstein also said you can’t choose just one because that is preferential.
If you look at all the examples of time dilation, twin paradox etc. they all are from one view only, the stationary observer. This makes the theory work. I’d like to see someone explain the the theory from both observers view and then see their results.
You wrote: “If you look at all the examples of time dilation, twin paradox etc. they all are from one view only, the stationary observer. This makes the theory work. I’d like to see someone explain the the theory from both observers view and then see their results.” However, acccording to Einstein in chapter 4 of ‘On the Electrodynamics of Moving Bodies’, when the traveling twin (clock A) returns to the stay-at-home twin (clock B), the travelling clock lags behind the stationary clock relative to the observers with the stationary clock and those those who travelled with the moving clock – or in terms of twins – the travelling twin was younger than the stationary twin relative to the stationary twin and observers who remained with the twin and relative to the travelling twin and those who travelled with him. In other words, when the travelling clock and the stationary clock are placed side by side after the motion between them, the traveling clock lags behind the stationary clock before all viewers of the two clocks. Some these viewers cannot see clock A lag behind clock B and some see clock B lag behind clock A but all see the same thing: in the words of Eiinstein: “Clock A (the travelling clock) lags behind clock B.
twins no longer have the same age (the clocks are no longer synchronied) but the traveling twin is younger than the stay-at-home twin (the traveling clock lags behind the stay-at-home clock). It seems to me that you are disagreeing with Einstein. John
Once again you are looking at the problem from a preferred view, the twin at home. And yes you are right. Look at the problem from the traveling twin position also.
Traveling Twin: I am stationary in the rocket. It is the earth that moves away at close to the speed of light. When the earth returns to me from traveling I compare my clock with the twin at home (on the earth). The twin on earth would experience the time dilation and hence age slower.
Trying to view this problem from this position is hard to do. Of course the earth could never do this, but Einsteins theory permits us to say it does because there is no preferred reference frame. What you are doing is looking at only the results from one of the twins reference frame. Doesn’t the other twin have the right to claim that it was him that was stationary instead of being the traveling one?
Forget the rocket and earth example for a moment. Imagine both twins being in outer space looking at each other. Pick one of the twins, A or B, and do the mind experiment, ie. twin travels away from the other and returns later. You get the results you mentioned, twins no longer have the same age (the clocks are no longer synchronized) but the traveling twin is younger than the stay-at-home twin (the traveling clock lags behind the stay-at-home clock).
Now redo the same experiment using the other twin as the traveling twin. When this twin returns home you again have the same results as above. Compare the results from both twins trips. How can you say which one is older or which one experienced time dilation. How can one twin be any different from the other? They both traveled the exact same path, distance, time, speed etc. So which one is older?
The confusion for the relativity theory is that Einstein claims, in his first postulate:
– The laws of physics work equally well for any inertial reference frame
– There is no preferred inertial reference frame.
Einstein wrote the theory. I just find it very interesting that people don’t understand what he is actually saying and the implications it causes.
A simple explanation of the first postulate could be: The laws of physics work equally well in any inertial reference frame and you can’t arbitrarily pick the inertial reference field you like and disregard the other.
So think about your comment above. Are you arbitrarily picking the twin at home?
@Walter
Sorry about the long response time.
The fact you bring up, that there is no preferred frame, is true. The fact that from each of their perspectives the other experiences less time is also true (although understanding how that works is a little tricky).
Addressing why one twin experiences more or less time than the other, despite neither of their frames being “preferred”, is what the post above is about. The difference ultimately comes down to the shape of the path they take through space-time. The twin with the more circuitous, longer path always experiences less time. That distance is defined using this notion for special relativity, and using this notion more generally (when you take into account gravity).
The twin on Earth is assumed to take a “straight line” from their mutual starting time and place to their mutual ending time and place. The “traveling” twin is assumed to take a curved or bent path. The straight path between the two meeting points experiences less time (counterintuitively).
While relativity does assume an equivalence of velocities (no preferred frame), it does not assume an equivalence of accelerations (when someone steps on the gas or the brakes, you’ll always know). The bending of the traveling twin’s path cannot be ignored, because (unlike velocity) it’s a real and objectively measurable quantity. As well, the time difference of the two paths can be objectively measured (and has been!).
Hope that helps!
You wrote: “The bending of the traveling twin’s path cannot be ignored.” However, acccording to Einstein in chapter 4 of ‘On the Electrodynamics of Moving Bodies,’ the traveling twin A (clock A) does not start his travels at the side of the stay-at-home twin, B, but apart from him. Then, according to Einstein, twin A (clock A synchronized with clock B) travels from his distant position to the side of twin B and that indicates that his journey was on a straight, non-bending path. When twin A reaches B, his accelerating, uniform, decelerating motion causes him to be younger than the stay at home twin relative to all observers.
According to Einstein, we have twins at rest with each other with the same age. Twin A goes into motion relative to twin B and B remains stationary. When twin A is at the side of twin B after this motion, he is younger than B relative to all observers. How can this be the case if, as you claim, the motion between the twins is the motion of A relative to B and this motion causes A to age slower than B, and if this motion is the motion of B relative to A and this motion causes B to age slower than A relative to all observers?
The issue here is that B’s reference frame is used to define the starting time for both twins. From B’s perspective A’s clock will read a lower value when they meet because A was moving.
Twin A will agree that when they meet his clock reads a lower value (this comparison, at the same place and time, is objective). However, A explains this as being caused by B’s clock starting with a higher value. They can’t start at the same time because, without simultaneity, they can’t agree on what the “same time” is.
I thought about using an argument like this, but tilted “now planes” and whatnot seemed like it would be a lot more difficult to convey or understand than the “longer path = shorter time” argument.
Wow, this is getting interesting.
You say “The difference ultimately comes down to the shape of the path they take through space-time. ” and “The twin on Earth is assumed to take a “straight line” from their mutual starting time and place to their mutual ending time and place. The “traveling” twin is assumed to take a curved or bent path. The straight path between the two meeting points experiences less time (counterintuitively).”
How can you assume this? By your logic then you could assume anything you want to make the theory work?
Use my example of the two twins in space, side by side looking at each other, both of their clocks are synchronized. Nothing else is around. They close their eyes and when they open their eyes they both see the other twin as traveling. In reality only one twin is moving the other is stationary, but they can’t tell which is which because the motion looks the same from each perspective. After they return from identical trips both twins claim the other is younger and had the slower clock. Is not the net result then zero? Each twin claimed they were stationary and the other was traveling, and their routes were exactly the same in speed, length, turns etc.
I try to look at this problem from the simplest view possible to try and understand it.
From my simple example of the two twins in orbit same path etc. can you show me why that won’t work?
BTW you guys are good, I enjoy reading your answers.
You wrote: “However, A explains this as being caused by B’s clock starting with a higher value.” You seem to be saying that when the journey of twin A begins while twin B remains stationary, twin B is already older than A and, hence, when A returns, twin B is still older that A.
The Twin Paradox is one of the most well known and debated paradoxes associated with Relativity theory. Opponents challenge Relativity theory on the
grounds that the Twin Paradox reveals an underlying flaw in the theory. Such opponents feel that the existence of a paradox, in and of itself, is sufficient to disqualify the theory. Supporters explain the paradox by introducing the concept of acceleration into the theory, thus limiting the interpretation to the twin that was undergoing the force of acceleration. (Frederick J. Hutchison)
This is my point exactly. When ever you claim things like different legs of travel, acceleration, different path, different starting clocks you change the original parameters to suit your own agenda. In my example of the two twins in space, they both see and experience the exact same path. Upon return they are exactly both the same age etc. even though they both observed the other twin as being in a slower time frame.
Einsteins theory only works from a preferential view. And then the result is basically meaningless. So what if the twin traveling through space came back to earth and was 10 years younger than his twin. Do you actually think that he would live the rest of his life on earth being 10 years younger than his twin, and that life would continue as before he left? That would be the result Einstein would like you to believe. But don’t you think having a brother all of a sudden 10 years younger than you would be sort of weird and maybe challenge some laws of science?
Think of it, there could be an opportunity here to make a fortune here. Give people a ride on the “Youth Space Ship” and after a 1 year trip they can be 10 years younger.
I’m really amazed at that scientists haven’t figured this out yet.
Walter, I agree that physicists have some splainen to do! Although I don’t agree that the thought experiment you are giving will prove why Einstein’s theory of relativity is invalid. If You have 2 people floating in space, if one moves away and the other doesn’t you could determine which one is staying in their inertial frame via an accelerometer they could be holding, ok? One stays in their inertial frame, the other moves away (accelerates), moves away at near the speed of light, causing their time to slow down (according the Special Theory of Relativity) with respect to the other twin which is staying in their inertial frame. And the moving away twin will have to make a total deceleration at the end of his/her trip and then reaccelerate in the opposite direction to get back to their twin (or some variation of this via a curved path). So that’s the excuse the believers in this aspect of the SToR will tell you. But I want to present to you a thought experiment I came up with which is much harder to counter, I think. Here it is (I wrote this more than a year ago):
Ok, let’s say we are standing on a huge inertial frame, a flat 2-dimensional space (just like people living on this planet used to think of the ground they were standing on). Off in the distance ahead of us are 2 train tracks, parallel to each other and separated from each other by a few meters. They are both going off to the east and the west from us, the viewer (using east & west directions assumes we are looking north). And our line-of-sight to them is perpendicular to the straight-lines of these tracks, which go off to the right and left for as far as we want. Let’s say if we go way up above this scene, the main points of interest are at the vertices of a huge equilateral triangle, but with the base of the triangle at the top. There is a train on one end of the tracks to the left, sitting there ready to go towards the right. There is a train on the right side of the tracks at that vertex of the triangle, pointed to the left ready to go. There are clocks at all three vertices of this triangle, as well as people: A clock at the train on the left (call this train A), a clock at the train on the right (call this train B). And we the viewer at the 3rd vertex of the triangle also have a clock. All these clocks are connected to this vast inertial frame we a standing on, and they are all still with respect to the inertial frame and with respect with each other. So they are all synchronized (am I allowed to do that, to synchronize clocks at various points of an inertial frame?). They all show the same time and they are all ticking at the same rate.
Now, people at train A get on their train and start going at a predetermined time (say 10 o’clock AM). Likewise, people at train B get on their train (train B) and start their train engine moving towards train A, also at 10 o’clock. They both are going to increase their speed from a dead stop at exactly the same rate. They will go faster and faster. They will both move a faster and faster rate, which will start to approach the speed of light. But they will both be moving with respect to their inertial frame at the same rate, only they will be going in opposite directions and on separate tracks. Finally they have their speed up to something like 1/3 of the speed of light (with respect to the inertial frame this is taking place on) and that will be their cruising speed.
Now, according to the special theory of relativity, people in train A will see the clock in train B as going at a slower pace then their own clock, because train B is going at a high speed in relation to train A. Like wise, the people in train B will see the clock in train A as ticking at a slower pace than their own clock. For example, when the clock in train A gets to show 11 AM, they might see the clock in train B as showing 10:50 AM. So they are both barreling along towards each other. Finally they are both approaching the midpoint between where they started from (this will be directly between the left vertex and right vertex of this equilateral triangle, and it will be directly “above” the bottom vertex where us the viewers are watching this from). They put on their brakes at the same time and slow down. Slower and slower and finally come to a stop right next to each other. The people in train A will have been watching the clock in train B tick along slower than their own clock, so once train A has stopped at let’s say 12 o’clock their time, they expect the clock on train B to be showing something like 11:30 AM. Likewise, the people on train B have been watching the clock on train A, seeing it ticking at a slower rate than their own clock, so when they stop and get off train B at a point when their own clock says 12 o’clock, they expect the clock in train A to show something like 11:30 AM. Now from the vantage point of us the viewers of these 2 trains from the lower vertex of this huge equilateral triangle, we who are sitting on this huge inertial frame that everything is taking place on, we would have seen both the clock on train A and the clock on train B as ticking at the exact same rate, although they would be ticking slightly slower than our own clock, since they are both moving with respect to us and even some significant fraction of the speed of light. But now everyone is stopped and standing on this one huge inertial frame of reference again. Now, how will the clocks on train A and on train B compare? To us the viewers of these trains, they should both show the same time. To train A occupants, train B’s clock should be retarded in relation to their own. To the train B occupants, train A’s clock should be retarded in relation to their own. But only one reality will manifest itself once the occupants of train A and train B meet at the middle, correct? What will they see when they view each other’s clocks?
The above thought experiment, to me, shows that Einstein’s Special Theory of Relativity has a definite problem, at least in regards to this time dilation business. There is a paradox, and I don’t see how it can be resolved. If a theory leads to an unresolvable paradox, then how can that theory stand? Of course, I shouldn’t say it definitely IS unresolvable. Perhaps I just haven’t thought of the solution. But what I love about physicists is the way they talk about this stuff like it is a fact, like its all settled. They can’t say “we believe this is the way it is”, they’ve got to say “this is the way it is” as if they are gods or something.
Hi Phaedrus, thank you for the very simple and interesting explanation.
I think you finally understood my original question, although you expanded it with the third observer, good job.
My whole point was that in any thought experiment only one side of it is shown (ie, The train passes the observer standing on the platform, who sees the clock on the train running slower, therefore time is running slower on the train.)
No one ever seems to look at the same thought experiment from the observer on the trains view, who would of course see exactly the same thing.
I would guess the reason for this is people can’t visualize a “stationary train and a moving train station/platform experiment”. So they add acceleration, turns and so on to the “stationary side” to show it was different from the real moving object (the train).
Therefore from a non preferential view thought experiment, when they both get back together the net result will be zero. No one traveled faster or slower than the other. Like you expressed in your answer above.
Also if the theory was true look at some unexpected consequences that could occur.
Say in the Twin Paradox, the traveling twin returns home and is 50 years younger than his twin brother on earth. Does he live the rest of his life on earth with a 50 year age difference to his brother? Think of the problems that this raises in how life works on earth, , biology etc.
the twin paradox is a nonparadox if you only involve special relativity, which deals with velocities.
true, both twins would argue that, while in constant motion, the other’s clock is what is running slow and not theirs. the difference is, unfortunately as stated, the path taken.
the oversimplified answer is:
twin A, when B accelerates away, does not notice anything different about the rest of the universe.
twin B, when it accelerates, notices the entire universe accelerating with twin A.
that is an asymmetrical experience, resulting B aging independently of the rest of the universe as seen by A, and A as seen by B, ages identically to wherever A happens to be (that is, stationary). simple.
Fist I want to say this is very exciting discussion. Brought new lights to my mind.
Just a thought, but no one seems to take SPACE into account here. If you spin in emty space (take the rest of matter in the universe away), would you feel a force pulling your hand outward eventhough you are spinning relative to nothing? You would feel a force pulling your hand outward, because you are spinning relative to space.
Can’t we say that SPACE is STATIONARY.
– If we say A is moving, A is moving relative to Space.
– If we say B is stationary, B is stationary relative to Space.
In this situation the 3rd observer is Space itself, and Space knows who is moving and who is not moving.
I think we are all missing something very important, time may not even exist.
I am not going to address The Twins paradox because without knowing if time exist there is no point at all.
Think about in more simple terms, the way physicist decided to quantify something (Time) they have no real proof of it existence at all, is prone to errors.
All it was proven in all the time dilation experiments is that, interval measuring on machines created by humans are susceptible to quantification errors and or interference.
The symmetry breaking comes from the acceleration. Time does not really pass slower in different inertial frames, only in accelerating ones. Clocks really run slower in higher gravity/accelerating frames. You can make the acceleration time as small as you like, but then the acceleration need to be higher and that just speeds up the effect. From the observer at home time for the moving observer appears to be running slow. From the moving observers point of view time also appears to be running slow in the other frame, until the turnaround is experienced. During the turnaround time in the world frame appears to speed up, catch up and overtake. The moving observer will make up some time on the way back, but not enough and will still be younger.
In every explanation of the twin paradox the assumption is that one twin is stationary and one twin goes through the acceleration, constant speed, deceleration cycle. Hence resulting with the traveling twin being younger. In any experiment like this, one observer does not have to be stationary, but only in an inertial reference field, ie constant speed or stationary. If you ran this thought experiment with both twins traveling exactly the same in opposite directions then returning they would both be the same age upon returning. However no one wants to explain what happens in this case because it proves the twin paradox is meaningless.
If you go back to Einstein’s thought experiment with the light clock on a train passing a stationary observer, the stationary observer sees the light clock traveling a longer distance (/\/\/\) and because Einstein claimed that the speed of light was constant it had to be time that slowed down (time dilation). Once again no one seems to question the apparent sawtooth pattern of the light pulse ( /\/\/\). Try visualizing the light clock positioned in a 45 degree angle like this /. Then visualize the results of the experiment. The observer would not see the same pattern but some combination of short and long light pulses because the light pulse would be traveling partially forward in the line of travel then backwards to the line of travel at the 45 degree angle. Time doesn’t slow down except in the logic used to try and explain what Einstien wanted to believe.
Einstein was a genius, no question there, but his theories are just that, theories. They have never been proven correct no matter what the claims are. Just because an experiment has results consistent with the theory doesn’t prove the theory.
It’s only a week I discovered this discussion (getting recently intrigued by the Twin Paradox) and I found it tremendously interesting and helpful in guiding my understanding of it.
My overall impression is that much of the confusion arises because explaining the ‘viewpoints’ of Alice and Bob from their respective frame(s) of reference is not the same as explaining the ‘symmetrical’ situation and that causes the frustrated responses.
Let me state the following :
The explanation for Alice stationary and Bob travelling – using Alice’s frame of reference – is straightforward and invokes (and needs) the time dilation effect only.
The explanation for Alice stationary and Bob travelling – using Bob’s frame(s) of reference – is more complicated and introduces the concept of changing simultaneity (which follows from Bob changing velocity – his crooked path). And when the ‘time gap’ effect is added to the time dilation effect things add up nicely and there is no contradiction. I see also that time dilation is not direction dependent but simultaneity is.
Making Alice the traveler (and using the rocket) and Bob stationary ‘on ground’ looks symmetrical but is of course the identical situation with a name switch.
The true ‘symmetrical’ situation that should be evaluated (and that is insisted on during the discussion) is Bob being stationary (not changing inertial frames) and Alice changing velocities (and changing inertial frames) WHILE BOB USES HIS ROCKET.
Someone remarks that asymmetry can be spotted by observing the Universe (“is it ALL moving relative to the observer or not”) and that seems correct to me, but forget about there being an earth and stars to hold on.
For Alice ‘to fall away’ from Bob and for Bob staying put at the same spot although firing his rocket it is convenient to imagine a gravitational field pulling Alice away but compensated for by Bob by the thrust of his rocket. After all, in GR acceleration is identical to gravitational pull.
Alice will loose ‘gravitational potential’ and her clock will slow down accordingly.
To ‘come back’ the gravitational field must be inverted symmetrical to Bob and therefore Bob must turn his rocket. Because Alice is ‘falling again’ (towards Bob) her clock will also be slow during the ‘inbound leg’ of her journey.
This ‘inversion’ of the gravitational field however inverts the gravitational potential difference between Alice and Bob, and this seems to me to be suspiciously equivalent to the ‘time gap’ fenomenon resulting from changing simultaneity in the SR way of explaining.
I’m quite sure (but I am no mathematician man and not able to do it) that the computation of the occurring time dilations plus the effect of the ‘potential switch’ will add up nicely to prove that Bob aged less (and in the same amount as before) than Alice, hence no contradiction and no ‘paradox’.
I see no way to avoid using GR in the explanation, but on the other hand we are only talking of ‘uniform gravitation’ (an idealized form) which does not curb (but only contracts) space.
I will try to explain, how I see it, as simple as possible with a simple example, using only special relativity.
Let’s assume that the traveling brother goes from earth to a planet X and back to earth and that the values are taken so that the time dilatation factor is 1/2. Then we have:
1) brother from earth looks at his clock/calendar and sees 32 years and thinks that his brother’s clock/calendar should show 16 years. Here we also have ship’s length dilation but is not important for this case.
2) brother from ship looks at his clock/calendar and sees 16 years and (thinking that he was at rest) believes that his brother’s clock/calendar should show 8 years.
Here the space dilation would need to be taken in consideration to explain exactly why the ship brother says 16 years and not 32. As opposed to the first brother he will see the whole system earth-planet shrinking on the moving direction but moving with the same speed as in case 1 – this would account for why in this case he sees 16 years; in case 1 first brother sees the ship shrinking and going with the same speed but the distance traveled would be longer.(complete calculations can be caried out, but I think the point is obvious).
Now to conclude: Both brothers would only be able to see their own watches which actually tells the correct answer, for both of them, but they can not fully trust what they “think” the other brother experiences. That is the whole point of relativity: you don’t know if you are moving or not. You can tell what time is on your clock and how much you aged but you don’t know if you are moving or if the other brother is moving.
They could send signals which might arrive back and forth after some time during the travel and they could see and calculate the actual time on the other side, but in that case they will have some information which will tell them which one of them is moving and which si not, and they can calculate the other’s clock using reverse formulas.
So the actual key is this: the reality will tell what really happened. They will not know until they meet and compare the clocks. Case 2 can be true also if the son in the ship actually stayed still and the whole system earth-planet really moved near the speed of light around him, back and forth :).
For this case only special relativity is needed to explain what is happening. And the fact that you don’t know(until you meet your brother), is the whole point of relativity.
The other points made in previous points are also true: if you know you are the one who’s moving or staying still you can easily calculate what the other clock is actually showing.
So indications such as accelearation, changing path, or the fact that you are on a ship clearly will give you a clue of the truth :).
I want to make a correction. This passage from the previous answer is wrong:
“They could send signals which might arrive back and forth after some time during the travel and they could see and calculate the actual time on the other side, but in that case they will have some information which will tell them which one of them is moving and which si not, and they can calculate the other’s clock using reverse formulas.”
Actually this is not possible assuming they don’t know which one of them is moving or not. Sending signals will only provide knowledge of the actual relative speed(if it is not known), but not which one is moving. Or it could present information to the receiver that everything works according to plan, but he would need to know who is moving and who is not.
So relativity is indeed great: inertial frame of references can not know if they are moving relative to another unless they have some other “fixed” observer, or some knowledge of who is actually moving, or if they accelerate, or if the other is accelerating … .
Pingback: Q: Since the Earth is spinning and orbiting and whatnot, are we experiencing time wrong because of time dilation? | Ask a Mathematician / Ask a Physicist
Let’s strip the twin paradox case down to the simplest features. Suppose Alice is sitting on a space station located in interstellar space, not orbiting anything, and not in the influence of any significant gravitational field. That’s about as close to a notion of standing absolutely still as one can get. Now suppose Bob passes by in a starship, which is then travelling at some large but constant velocity. If they communicate by radio during the fly-by, they can compare clocks, and it will seem to each of them that the other person’s clock is running slow, because both are genuine inertial frames of reference.
So this interaction proceeds, and soon enough Bob’s ship pulls out of radio range of Alice. Some years later, Bob returns to the neighborhood of Alice’s station, and they again compare clocks. Intuitively, the result should be the same as before.
Conventional wisdom says no, and explains that in order to get back to Alice, Bob’s flight path must have curved, because if he continued in a straight line, he would go on forever without ending up back where he started. Because velocity is a vector, a curved path (even travelled at constant speed) does not hold velocity constant, so Bob did not maintain an inertial frame of reference, and relativity no longer endorses the conclusion that their perspectives are equally valid.
The critical step in that argument is that Bob’s path was curved. The reasoning above is correct under our usual assumption that the geometry of the universe is Euclidean. More advanced physical theories (general relativity, string theory, etc.) feature non-Euclidean geometries, but usually those in which the reasoning still holds. However, there are well-known geometries in which the reasoning is invalid. The simplest is spherical geometry, which is standardly understood as a 2D geometry where the `plane’ is actually the surface of a sphere. If you continue in a straight line alone the surface of a sphere, you will end up back where you started. So if the universe where Alice and Bob are living is actually the 3D surface of a hypersphere, we have Alice and Bob still potentially being in inertial frames of reference on their second encounter, which makes the twin paradox really paradoxical.
Of course, the usual response to that paradox is that fortunately we don’t live in such a universe. However, we don’t have any really good evidence of that, except for the unacceptability of the result. It’s also worth noting that a standard SF device for FTL travel is to move the ship from the real universe to some parallel universe whose properties are more conducive to travel. Perhaps the hyperdrive universe might have some geometry that allows the twin paradox to be fully exploited.
Hi All,
I have not read all the posts. But I do not really agree with the view here.
For simplicity, let us just say that Alice is at New York and Bob is flying from New York to London at a straight line at high speed. In NY, they can synchronize the clock and we can assume that Alice is at the constant speed already and no acceleration is needed. Just a little radio signal would be enough to synchronize the clock. Also the clock in London and NY are also synchronized. Here again for simplicity, we can assume that the three clocks are all set to 0 at the start in NY. When Alice gets to London, through a little radio without decelerating, she can compare the clock with the clock in London. I say that the reading on the clock in London is larger. In other word, the time passed on earth is longer. This should resolve the twin paradox once and for all, right?
How do I get this conclusion? Well, first we notice that from NY to London, whether on the ship or on earth, if I shoot a beam of light straight in the direction of London, it will travel side by side and reach the same distance using the time passed on earth. In other word, the speed of the light on flying ship or the earth is the same seeing from earth. With this fact, at the start in NY, we can shoot two beams of light, one on earth and the other on the flying ship from the same position or on a line perpendicular to the flying direction. Then when the ship arrives at London, we can calculate the time passed on earth and ship using the distance light traveled. Note viewing from earth, the end point of both light beams is the same. But the starting point of the light is not depending on whether you calculate on earth or ship because ship is now in London. So the time passed on the ship is shorter. Doesn’t this explanation resolve the paradox completely?
Walter Roberts,
You say:
Forget the rocket and earth example for a moment. Imagine both twins being in outer space looking at each other. Pick one of the twins, A or B, and do the mind experiment, ie. twin travels away from the other and returns later. You get the results you mentioned, twins no longer have the same age (the clocks are no longer synchronized) but the traveling twin is younger than the stay-at-home twin (the traveling clock lags behind the stay-at-home clock).
Now redo the same experiment using the other twin as the traveling twin. When this twin returns home you again have the same results as above. Compare the results from both twins trips. How can you say which one is older or which one experienced time dilation. How can one twin be any different from the other? They both traveled the exact same path, distance, time, speed etc. So which one is older?
The source of your misunderstanding is the fact that which of the twins experienced the acceleration is objective from either of their frames of reference. Let’s ignore the fluff and take your scenario of two twins, A and B, floating next to each other in space, and twin A gets a rocket and uses it to fly away from twin B and back. In this case, they will both agree from either reference point that twin A was the one who accelerated and twin B did not. Even if the rocket were invisible, twin A would feel the force of the acceleration on his body and twin B would not. That is why twin A would end up being younger when they are eventually reunited. If it were the case that they both had rockets and used them simultaneously to fly away from their center point and back, then the twins would end up the same age. Even though the relative position of each to the other would be the same throughout these two scenarios, and in the first scenario each would see the other “moving away and back” from his frame of reference, which of the twins actually experiences the acceleration is objective from either frame of reference because only one would have a force applied to his body.
@Jack
This is the misunderstanding I had for awhile. You’re absolutely correct; it’s the ACCELERATION which determines who experiences the d dilation effect. In fact, this is the first stepping stone to relating dilation to gravity: gravity is equivalent to constant acceleration. Thus there’s a direct link between higher gravitational fields creating dilated time lines – or as the armchair physicist would point out, “that’s why time passed slowly in Interstellar, when they were near the black hole.”
Carrying this further, one can imagine several crazy systems of acceleration, even with static speed traveling in a circle (hence why velocity incorporates direction, thus acceleration, meaning a change in velocity, can include static speed with constantly changing direction), where time dilation would still occur.
This is where Walter trips up.
Time is relative to your movement through space. That is what the sides of the triangle represent. The faster your move through space the shorter distance you move through time. The only thing that changes is how you perceive the changes in your movement through time.
See this is the problem that you all forgetting. Specificslly to Walter, they tested the effects of time dialation in the real world. They tested this in the Hafele–Keating experiment. They took two cranium atomic clocks and put them “at rest” on the surface of the earth and then flew one plane going in the direction of the rotation of the earth and one opposite. If your thought experiment were true then all clocks would have returned with the same time. This was not the case. The clock traveling at a greater velocity, with the rotation of the earth, as predicted was slower than the one at rest. Similarly the one traveling opposite was slower than those at rest. This is real world Confirmation of Einsteins theory. Yes during travel all observations are relative to their observers reference frames. But after travel of either body when they both return to the same reference frame they are then able to make objective observations about the state of any positions in time because they are both in the same frame of reference. That’a your problem. You assume that all observers stay in separate frames of reference when in actuality they return to the same frame of reference after travel and are then able to reconcile any differences caused by their separate reference points and separate paths taken through space time. When at rest space and time are in equilibrium. When not at rest travel space causes time to distort. When back at rest space and time return to equilibrium. Thus the clocks on the ground don’t change whereas the clocks on the planes do. The reason we can record these results in real time is that the disparity created is small enough as to not be preceptable to us without using specialized equipment, not because it isn’t fast enough. So.the experiment is valid. At high enough speeds we would be measuring this change in years and not nanoseconds however so the difference would be more than apparent. I hope that clears things up.
I refer to my comment of 23 august last year when I tried to describe what the ‘real symmetric situation’ should be.
I think it also makes clear that ‘feeling the force’ is NO proof of acceleration : Alice is accelerating in free fall by gravitational pull and does NOT feel any force (as long as tidal forces don’t come into play, but my example proposes ‘uniform’ gravitation) and Bob DOES FEEL the force of his rocket but stays in fact immobile and therefore is not accelerating.
Objectively in this situation Alice is accelerating (and also loosing potential in the gravitational field) and therefore her clock slows down, both during the ‘falling out’ (outbound journey) as during the ‘falling in’ (inbound journey). At first sight this would mean that Bob aged MORE than Alice. Note however that the ‘turning point’ here involves a ‘gravitational inversion’ (a gravitational potential switch) – which cannot happen in the real world I suppose – but if it could I reckon that this factor changes the calculation in such a way that in turns out that it is nevertheless Bob who aged LESS than Alice. I think this can be interpreted as the equivalent of the ‘time gap effect’ created by the change in simultaneity in the original situation.
Can mathematician/physician man give some helping comment here ?
And Jack : One example of your ‘crazy systems of acceleration’ are the satellites doing turns around the earth : their clocks slow down because of their speed relative to us BUT their clocks also speed up because of their altitude in the gravitational field of the earth (position with higher potential). The two effects must be added and the net result is that they tick quicker.
Correction for the satellites : the speeding effect only takes precedence if the satellite is in a high enough orbit (I had GPS-satellites in mind).
Since the universe is speeding up, it stands to reason that we’re traveling along the space/time continuum faster than our ancient ancestors did. According to relativity, time should be passing slower on Earth now, relative to the rate it passed eons ago. If we could look back in time and compare our daily activities side-by-side with our Neanderthal cousins would we see them whipping around in super-fastmotion compared to us today?
Hi Craig,
I am not so sure about universe expansion. In GR, we should only get whether light goes forever or not, not space here and there expands. By the way, space expansion sounds like anti-gravity to me. We have no evidence whatsoever in our labs about the existence of such a force or process. By GR, we should only get something like blackhole stuff, not a space expansion cosmology. It is possible to imagine that due to light radiation, matter gets relocated in the universe… But then what do I know as a hobbyist?
Can anybody help me to define the “clock time” or “time” or “rate of happening something”.Or can anybody replace this T i think every explanation of time is again somehow bounded with time for an example dilation of time is depended on velocity and time
If relativity is right, though we do not have absolute time and space, for each reference frame, we can define the time the same way and it should mean the same thing to each frame. Obviously one second in one frame is certainly not equal to one second in another frame even though it is defined in exactly the same way. This alone sounds weird to me too.
By the way, star trek 5 year mission if it is taken as earth time, then they could go much beyond our closest star. If it is the spaceship time, then many years would have passed on earth. Either way, it could not be true by relativity.
Also by SR, any thing going in anyway faster than light would mean a way to send message from future to past. This would have a logical problem. So it seems there is no way to go faster than light.
I didn’t see any mention of Doppler shift here, so I’ll give my 2 cents worth based on a book I read by PJE Peebles.
Assume Alpha Centari is exactly 4 light years away, and one twin is
traveling
there at 4/5 speed of light. (Using a 3,4,5 triangle I avoid
irrational numbers in my computations.
Traveling at 4/5 the speed of light, from the point of view of the
stay at home twin, the trip will take 10 years, 5 years there, 5 years
back.
Time for the traveller T’ = T( sqrt( 1- (v^2/c^2))) = 3/5 T
Likewise, the distance for the traveler, D’ = 3/5 D
The traveler on the spaceship sees himself traveling a distance of
4*3/5 = 2 2/5 light years in a time of 3 years, and likewise the 2 2/5
light years back
in a time of 3 years, so the traveler will see the trip as lasting 6
years.
Say the twins have super telescopes and can see each other throughout
the trip.
As long as they are traveling apart, the twins will see each other as
aging at 1/3 speed. As long as they are traveling towards each other,
the twins will see each other as aging at triple speed.
The difference is, the traveling twin will see the stay at home twin
as aging at 1/3 speed for the 3 years to Alpha Centauri, for a total
of 1 year,
and at triple speed for the 3 year trip back to earth = 3*3=9.
The traveling twin will see the stay at home age 1 year during the
trip out, and 9 years during the trip back, for a total of 10 years.
The stay at home twin will see the travel age at 1/3 speed for 9
years, the 5 years it takes the traveler to get to Alpha Centauri,
plus the 4 years it takes the light to get back to earth. Since the
total trip will take 10 years, the stay at home twin will see the
traveler age at triple speed during the one year he observes the
traveler coming back to earth. The earth observer sees the traveler
age at 1/3 speed for 9 years, for a total of 3 years, and at triple
speed for 1 year, for another 3 years, giving 6 years for the round
trip.
Both observers see each other aging at the same slow rate while moving
apart, they see each other aging at the same fast rate while moving
together. The difference lies in one observer deliberately changes
the relative motion of his rocket from moving away from earth to
moving towards earth, and the other observer remaining passive, and
not seeing the change until the light from the
traveler reaches earth. If the earth could be accelerated like a
rocket ship, and the earthbound observer decided to change his frame
so the rocket appeared to be moving towards him at 4/5 lightspeed
rather that away at 4/5 lightspeed, while the rocket remained in
motion past Alpha Centauri, then it would have been the earth twin who
appeared to age less.
Of course you could have some intermeditate situation where BOTH
observers decide to change their relative motion before they see the
other observer change his motion.
This so called Doppler effect description while it is correct, it describes and involves some other aspects of twin journey. As we can see, many posts here are about understanding the essentials of time dilation in twin paradox. Some are not convinced that it is real. In fact, there are some well known arguments against it. I have to say there are quite a few papers written on the topics in scientific journals. Some of them are as recent as about 15 years back or later. It is amazing.
So I have to say, this description does not help much those who have doubts about relativity to see time dilation in twin paradox. Similarly I find the space time diagram argument, direct Lorentz argument and some math argument are not convincing enough.
What I do not understand is that, in Google, we can still find many websites presenting arguments against time dilation. It says something like if you select twin A as reference frame, twin B’s clock is slower, and if you select twin B as reference frame, then twin A’s clock is slower , hence a contradiction. Then there is H Dingle’s clock rate argument… Do you guys see these?
“What I do not understand is that, in Google, we can still find many websites presenting arguments against time dilation. It says something like if you select twin A as reference frame, twin B’s clock is slower, and if you select twin B as reference frame, then twin A’s clock is slower , hence a contradiction.”
People are used to dealing with an earth based reference frame, in which the speed of light is almost instantaneous, and they apply their personal experience to travel for years at a significant fraction of the speed of light. If something is traveling at a significant fraction of the speed of light, you cannot apply normal experience here on earth because you won’t see the object “now” any more than you see Alpha Centauri or
Rigel, or even Jupiter or Mars “now”.