Physicist: Everything in special relativity, no matter how weird, eventually boils down to the speed of light being the same to everyone. It’s just not immediately obvious how. The very short answer is: if v and w are pointing in the same direction, then their sum, V, is 
A fanatical adherence to this principle (that the speed of light is the same to everyone) allows relativistic effects to change the distance and time between events, slow the passage of (other) people’s time, even (under the right conditions) rearrange the order of events. However, what it can’t change is that an event happens, and (oddly enough) it can’t change the ordering of speeds in any particular direction.
Say you take one direction, and you have a variety of things traveling in that direction going fast, faster, and fastest. While different perspectives may disagree on exactly how fast each one is going, they will all agree on the same fast-faster-fastest ordering. And light is always the same, and always the fastest.
Imagine pitching great Walter Johnson throws a baseball at 0.3c (30% of light speed, or about 200 million mph). Clearly Walt (being stationary) is moving slower than the ball. In turn, the ball is clearly moving slower than a passing beam of light.
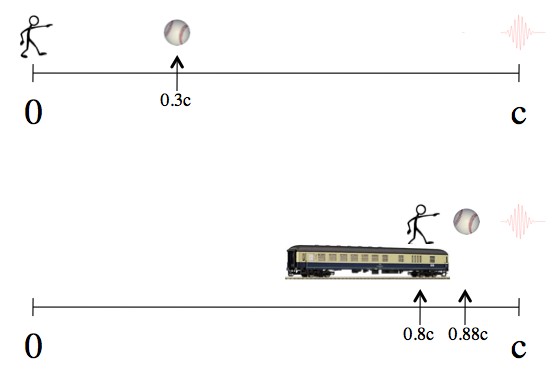
Top: for the pitcher's point of view he's sitting still (zero velocity), the ball is moving at 0.3c, and light is moving at c. Bottom: the same situation, but moving to the right at 0.8c. This is how you "add" 0.3c and 0.8c.
Now imagine that, unbeknownst to Walt, the entire baseball field was actually on some kind of super train tearing through the countryside at 0.8c. “Intuitively”, if the usual addition of velocities held up, the ball should be traveling at 1.1c (0.8c+0.3c). But relativity, being neither kind nor reasonable, elects instead to be stubborn about the whole “speed of light thing”.
Even on the train, Mr. Johnson is certainly still traveling to the right slower than the ball, which is still traveling slower than the light. Moving to a different frame doesn’t change the order, it just scrunches all the velocities up.
If light obeyed the normal addition of velocities, then the speed of the light beam that pitching great Walter Johnson saw should now be 1.8c as seen by someone watching from beside the train tracks (the c Walter saw, plus the 0.8c of the train moving the whole shebang), and there should be no scrunching. But it isn’t 1.8c! That’s where the fact that the speed of light is the same in all reference frames comes up.
In Walter’s frame (top) the light is (naturally) traveling at light speed, and the ball is traveling slower than that. In the frame where the whole thing is passing by on a train (bottom), the same light is still moving at c, and the ball (although traveling at a different speed) is still slower.
The classic question “what happens if I’m already on a beam of light and…” sadly doesn’t make sense from a physicsy standpoint. No material can travel that fast. In fact, nothing that experiences time can move that fast. You’re always restricted by the fact that, from your own point of view, you’re sitting still and light is traveling at light speed (try it!).
If you’d like to find out why in the example above the ball ended up traveling at 0.88c instead of something else, or what would happen if Walter “The Big Train” Johnson had thrown the ball sideways off of the train, you’ll find it milling about in the answer gravy.
By the way, here’s the same example as seen from the baseball’s point of view:
On the off chance that the question would come up, and just to emphasize the symmetry of it: this is the same example from the baseball's point of view.
Answer gravy: When you go from one frame to another (changing from moving at one speed to moving at another) all you’re really doing is rearranging coordinates. This rearrangement is called a “Lorentz boost” or a “Lorentz transformation“, and it’s surprisingly similar to a rotation (which is also just a rearrangement of coordinates). Adding velocities amounts to applying a series of Lorentz boosts.
When doing a “coordinate transform” the weapon of choice is matrix multiplication. It’s easy stuff. If you can add and multiply you can do matrix multiplication. Although I won’t lay out the technicalities here, I’ll try to be gruesomely slow algebraically.
Also, when dealing with 4-velocities (velocities that have 3 space components and one time component) it happens to be useful to not consider the time dimension, “t”, by itself, but rather “ct”. You can think of this as natural units, or whatever. It just tends to make everything work out beautifully.
A general 4-velocity looks like this: 
An object at rest has a velocity of 
The matrix of the Lorentz transformation to a frame moving at velocity v in the x-direction is given by: 
Matrix aficionados may notice that this looks almost like a rotation in the time and x directions. Just a note, those 1’s mean that the y and z components are unaffected.
So when the pitcher throws the stationary ball he “boosts” it into a new frame (by making it move). Mathematically you can do this with matrix multiplication:
The top 
So, the baseball is moving with ordinary velocity v (like you’d hope) and 4-velocity 
So, what’s the normal velocity after adding the speeds v and w? What is the final velocity “V”? Algebra!:
Holy crap! The equation we normally think is right, 
So, in the example from (way) above the speeds were v=0.3c and w=0.8c. So
Seems like a long trip for 
Finally, if you choose to add velocities that aren’t in the same direction, you just have to rotate one of the Lorentz matrices. For example, while all of the examples so far have been in the x direction, a boost in the y direction would be given by:
If Walter Johnson had decided to throw the ball in the y direction on the train, and everything else stayed the same, you’d figure out the end 4-velocity of the ball by doing the following boosts:
It seems a little spooky that when the velocities aren’t parallel, velocity addition is no longer commutative (notice that the alphas and betas aren’t on equal footing), and the order in which you do the boosts becomes important. Of course, it may be spooky that that isn’t always the case.
“A fanatical adherence to this principle (that the speed of light is the same to everyone) allows relativistic effects to change the distance and time between events, slow the passage of (other) people’s time, even rearrange the order of events.”
Wait, rearrange the order of events? I thought that was impossible even with relativity, because it would violate the principle of causality. I know that if you’ve got two events A and B, you could have A and B simultaneous in one reference frame and A following B in another, but I was under the impression that if there exists one reference frame in which A follows B, then in all reference frames the time of A is >= the time of B.
I should have been more precise and less showy.
“Spacelike separated” events A and B can happen at the same time, A then B, or B then A. However, causality is maintained since they’re too far away in space, and too close together in time to send any kind of signal to the other.
I’ll put a disclaimer in the post.
Recommendation for further reading: The Geometry of Spacetime by Callahan. Only requires understanding of basic linear algebra and calculus.
So put simply, the ball will travel in time ?
Not quite that simply. Everything (with mass) travels in time, it’s just a matter of how exactly.
Since time is a function of light speed, would you experience time if you could travel at light speed, and if you could, then would the thrown ball effectively travel into the future?
To quote Douglas Adams, “this is of course, impossible” 🙂
Nopers! If you could travel at light speed you’d find that time wouldn’t pass at all. Any journey, no matter how long (distance-wise), would be instantaneous from your perspective.
Keeping with the “ordering of speeds” idea of the post; since the baseball needs to travel between your speed (the speed of light) and the speed of light, the only speed it has the option of going is also the speed of light.
Of course, not being able to move at the speed of light, this is a non-issue.
What you seem to be saying is that the faster you travel, the slower you are able to move, so that, at light speed, you would not be able to move the ball in the direction of travel.
This seems to imply that were you able to travel faster than light, you would travel into the past.
Hi, there has recently been an experiment at Cern with results indicating faster than light travel occured. I am interested on your thoughts on this. I studied physics at degree level some years ago and will never forget one event. Our lecturer stated that the faster speed possible is c. He then went on to’proove’ this with 4 pages of mathematics with the answer being zero. I queried that i did not follow WHY c is the max speed. Lo and behold he went through the maths again. He could not explain in physical terms why.
I believe science is guilty of over indulgence in theories without experimental backup and use of assumptions. We must use observation as the key and then try to explain what we see with theories. Otherwise it all gets ‘fantastical’.
I know there is currently a variable speed of light theory gaining ground. And why not ! Makes more sense than forcing a constant c then having to deal with bending space time, worm holes, parallel universes etc…. If we are honest with ourselves we know we do not really fully understand relativity (special but especially general) until we can EXPLAIN it to the lay person.
One little thought experiment if I may. Imagine you have a piece of string the length of 299km I.e. the length that light would travel in 1 second. I am at one end and you at the other. Ignoring the fact that it would be heavy and assuming it is rigid, if I pull it you should notice it moved almost instantly. So hasn’t information just travelled faster than c ?
You’re absolutely right! Science in general is an observational practice, and almost all practicing scientists take that very seriously (not including mathematicians, of course).
We’ve done a couple of posts on this stuff:
Q: Why is the speed of light the fastest speed? Why is light so special?
Q: CERN’s faster than light neutrino thing: WTF?
I’ve never heard that the constantness of C requires worm holes and parallel universes. Do you have a reference for that?
The perfectly rigid rope or pole thought experiment is a classic. But it turns out, it doesn’t work. The information that passes from one atom to another down the length of an object that says “move” moves at no faster than light speed.
You say that the maximum attainable speed permissible by the relativity is the light speed. What about the “inflation”? We have enough evidence that it occurred soon after the “big bang”. Inflation expanded the universe at a rate faster than the speed of light. How?
“Inflating faster than the speed of light” is a phrase that shows up in a lot of science documentaries, that really shouldn’t. The inflation refers to space itself, whereas “speed” refers to movement through space. In fact, the rate of inflation is described in terms of “speed per distance”, as opposed to just “speed”.
You can think of inflation as the expansion of a balloon, and movement through space as things moving across the surface of that balloon. If the balloon expands quickly, that doesn’t mean that dots drawn on the surface are moving fast. In fact, it doesn’t say anything about the speed of things on the surface.
This is the BEST explanation of this topic I have ever seen! Thank you!
If two photons are moving in opposite directions, what is their relative velocity?
IS it c or 2c?
how can it be 2c when any speed cannot exceed the speed of light?
Depends on your perspective.
In between the two photons you’d say that their relative velocities are 2C.
However, neither of them sees the other moving faster than light (or in fact at all), and you of course see them both moving at exactly light speed.
The important thing is that nothing is moving faster than light with respect to anything else.
I had asked a physicist a very similar question and got a beautiful answer. He was nice enough to take the time to make charts and everything. I had asked “if a spaceship leaves space station A toward space station B at 0.6c and another spaceship leaves B toward A also at 0.6 then I sit at poin C in the middle to witness this 1.2c collision what would happen? The actual question was would each spaceship see the other spaceship as moving at 1.2c toward them? The answer was no. I forget the exact speed from the math but it basically turned out where each ship would see me traveling at 0.6c toward them and the other ship at some number > 0.6c and < 1.0c.
One of the ways I break it down in my lay mind is that to the Newtonian relativity we experience intuitively, space and time are constants that all frames agree on. They are rigid. Well once the speed of light was realized to be rigid… Then everything else has to flex. So it's most intuitive to preface any discussion of relativistic speeds as saying "on a scale of 0 to c how fast were things going" and that's where you will get two reference frames that agree … Would draw a scale that looks similar in order and proportion but the actual numbers in the middle would not be the same. They would correlate though in relation to everything else. And the idea of breaking the speed limit by simply going faster does the same weird things when you try to exceed an infinite limit in any system. Merely going faster than c is like merely going colder than absolute 0. As you approach the limit and your curve on the graph gets closer its like you can't get there you just zoom in on the gap more.
It's like if you spend twice as much energy you only go a fraction faster or a fraction colder. 0.99 becomes 0.999 becomes 0.9999 or 0.01 becomes 0.001 becomes 0.0001. It's like the way extents work.
If light can’t move at different speeds relative to a perspective, how do you account for red shift? The baseball thought process is archaic and repeated so many times as fundamental, it hasn’t been challenged in modern times. Of course! a baseball being thrown is practically zero compared to the speed of light, therefore has no bearing in calculations or reality for that matter. What physical experiments prove this?… It’s easy to write equations based on illusions, and to consider them truth when you are out of the realm of perception.
That’s a good point about redshift. I never would have thought of that. If your speed relative to a photon never changes, then why does it appear to redshift when you move away from its direction of travel?
The baseball thing, however, is relevant no matter how old the analogy gets. Many experiments have proven that time slows down as speed increases. This can easily be translated into terms of throwing a baseball, or shooting a gun. Speed is distance over time. If time is slower, but distance hasn’t changed, then the speed must be slower.
I’m significantly younger and less educated than most people commenting on this article and read an answer by the physicist saying that if “you” could travel at light speed you wouldn’t experience time but everything would happen instantly. If that were true why does it take light “time” to travel? It supposedly takes about 7-8 minutes for light to travel to Earth from the Sun. So if I were riding a light beam just emitted from the sun I wouldn’t experience the 7-8 minutes I would just end up on Earth almost simultaneously as I left the sun? I’m not sure I quite understand how that works. Sorry if I’m a little below the average IQ of the average user on this site but it just struck me as interesting. Thanks
@Caleb Salem
That’s exactly right. It takes light about 8 minutes to travel to the Earth according to our clocks, but the light itself experiences neither the time nor the distance.
Another lay here. The way Im inferring matters: if, let’s say, we stood at the fore of the train (on top of the moving object, obviously) and there was no wind resistance (it’s impossible, but for arguments sake,) if we failed to throw the ball equal to or greater than the speed of the train, it would wind up at either where we stood (“equal to”) or behind us (failure to throw the ball “greater than”). Now about the light beam deal: since there are no postulates (again, all we have in a world of scientific research, as u alluded to, are observable practices) that evince the speed of light can be superseded by anything) the ball, as a reference relative to the object moving through space, should wind up several hundred thousand miles behind. True?
The reason light has a constant speed, why the speed of a mass isn’t added to the speed of light originating from that mass, and why relativity has nothing to do with it is simple.
Light originates from mass, and indeed, is just a different form of mass. When mass reaches the point that it changes from mass to a photon of light, it does the change instantaneously. That means, that no matter the velocity of the mass, the instantaneous creation of a light photon means that the mass is stationary in space at that instant. Thus, light originates from a stationary point, and as light originates at speed C, it will always have a speed of C.
Each individual photon in a beam of light originates from a stationary point, and as light originates as individual photons, and not a continuous wave, then the photons originate from different stationery spots along the direction of travel.
There’s nothing mysterious about it. There is certainly nothing that prohibits anything going faster than light, as light behaves by traditional physics, and doesn’t need relativity to explain its properties.