The original question was: How does reflection work (what happens on the reflective surface), why is the angle of incidence equal to the angle of reflection and can this be viewed both from particle and wave point of view?
Physicist: In a way, reflection can’t really be described as particle phenomena at all. A solid object can bounce, but that’s just a flexing and un-flexing of the object. An individual particle can’t bounce the same way, but due to its wave nature it can reflect.
Essentially, a reflective surface enforces some kind of restriction on the wave, usually that the wave must have zero amplitude at the surface.
For example, to derive the existence of echos (off of a rock wall or something) you declare (reasonably) that the perpendicular velocity of the air at the surface must be zero. That is; air can flow along rock, but it can’t flow into or out of it.
For another example; it turns out that the electric field in conductive metals is zero (if it’s not, then the charges in the metal move to make it zero). In a way mathematically almost identical to echos you can then describe light (an electromagnetic wave) reflecting
off of shiny metals.
It usually makes most people a little uncomfortable, but the best way to enforce the “zero boundary condition” (like in both examples above) is to:
1) pretend the surface isn’t there and then
2) describe the situation in terms of another wave coming through where the surface was that’s exactly the same as the incoming wave, but negative.
The result is that the new wave coming back at you cancels out the original wave at the location where there should be the reflecting surface. So, the boundary condition is satisfied: the wave has zero value at the surface (whether that’s zero air movement, or whatever).
The negative wave that comes out doesn’t sound or look any different, and the original wave disappears (past the surface). What we perceive in waves is frequency (“pitch” for sound, “color” for light), but the difference between the old and new waves is “phase”, which we don’t sense.
The important thing to keep in mind is that only what happens on the near side of the surface is real, everything on the other side is just a mathematical artifact, and (once the math is done) should be ignored.
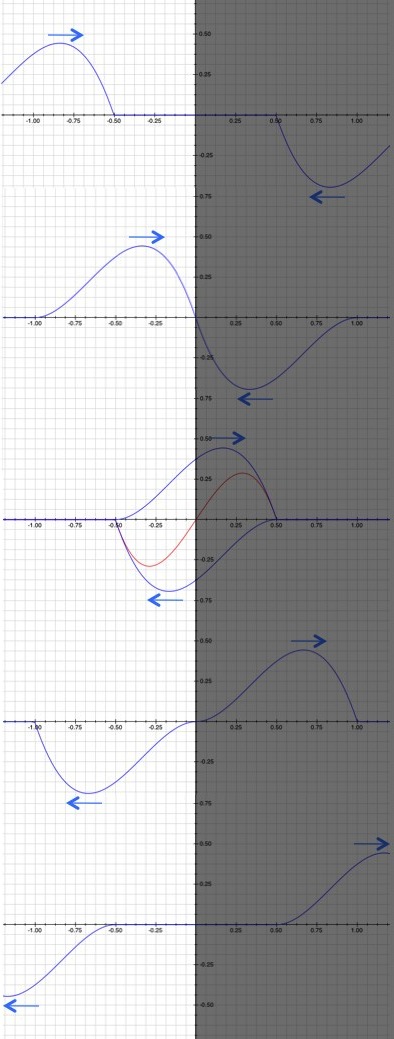
Mathematically, as long as you restrict your attention to the left side, the following cases are exactly the same: 1) a wave reflecting off of a non-permeable surface and 2) no surface, but two waves, one positive, one negative, passing each other such that they exactly cancel. The red curve is the sum of the two waves.
Notice that this only applies to the component of the wave that’s perpendicular to the surface. The part of the wave that runs parallel to the surface is unaffected. That gives you exactly what you need for the angle of incidence and the angle of reflection to be equal.
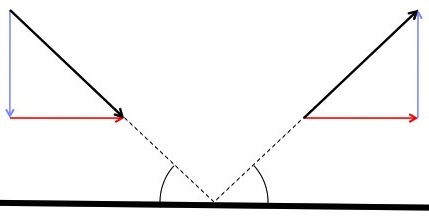
Only the part of the wave perpendicular to the surface (blue) gets flipped. The part of the wave running parallel to the surface (red) is unaffected. Draw a picture, notice the similar triangles, and boom!: the angle of incidence equals the angle of reflection.
This has nothing to do with the question, but it’s interesting. The “ignore the surface and pretend there’s something balancing on the other side” technique is useful all over the place. For example, when something flies (birds, planes, etc.) it does so by pushing air downward, one way or another.

Pelicans, and many other birds, fly low over water to take advantage of the ground effect, which can be imagined in terms of the influence of the "reflected pelican" pushing air up. Also, there are fish involved.
Again, since air can’t move through a solid surface, you can model the fluid dynamics (“fluid dynamics” includes “air dynamics”) of something flying low over a surface with an identical “flying reflection”. So while a bird pushes air downward, its “reflection” is pushing air up, with the net effect that no air moves through the surface. The upward push of air manifests as the “ground effect” which is experienced by pilots and birds as a cushion of air that helps hold you up when you get close enough to the ground.
Even as a passenger, you can usually feel the ground effect just as your plane is about to touch down.







This seems to me more like a useful way to describe it and do math on it than a true explanation of the phenomenon.
I wonder what happens on the atomic scale. What if a hydrogen atom reflected off of a uranium surface. It would still reflect, right? I would think, that the hydrogen atom would experience the surface atoms as giant bumps rather than a smooth surface, and therefor bounce in an almost random direction. How come that the angle of incidence and the angle of the reflexion is still equal?
Still a very interesting post, though 🙂
Fair enough.
The point (such as it was) was: at a surface a wave suffers some restrictions, the results of which can be predicted/modeled mathematically. The math predicts that the component of the wave perpendicular to the surface will be reversed. The rest of the post is just a useful trick.
On an atomic scale, because of the waviness of particles, you get interference patterns from the particle interacting with many of the atoms in the surface. You can actually take advantage of that fact for things like “crystallography“.
For charged particles on the other hand, they are generally pushed around by the charges in the surface, and “reflection” doesn’t enter the picture. Specifically, the angle of incidence and the angle of reflection are generally not the same.