Physicist: There are a couple of different contexts in which the word “dimension” comes up. In the case of fractals the number of dimensions has to do (this is a little hand-wavy) with the way points in the fractal are distributed. For example, if you have points distributed at random in space you’d say you have a three-dimensional set of points, and if they’re all arranged on a flat sheet you’d say you have two-dimensional set of points. Way back in the day mathematicians figured that a good way to determine the “dimensionality” of a set is to pick a point in the set, and then put progressively larger and larger spheres of radius R around it. If the number of points contained in the sphere is proportional to Rd, then the set is d-dimensional.
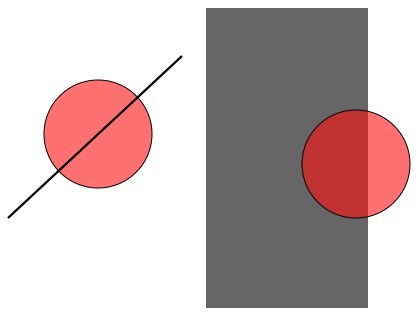
(Left) as the sphere grows, the number of points from the line that it contains increases like R, so the line is one-dimensional. (Right) as the sphere increases in size the number of points from the rectangle that it contains increases like R2, so the square is two-dimensional.
However, there’s a problem with this technique. You can have a set that’s really d-dimensional, but on a large scale it appears to be a different dimension. For example, a piece of paper is basically 2-D, but if you crumple it up into a ball it seems 3-D on a large enough scale. A hairball or bundle of cables seems 3-D (by the “sphere test”), but they’re really 1-D (Ideally at least. Every physical object is always 3-D).
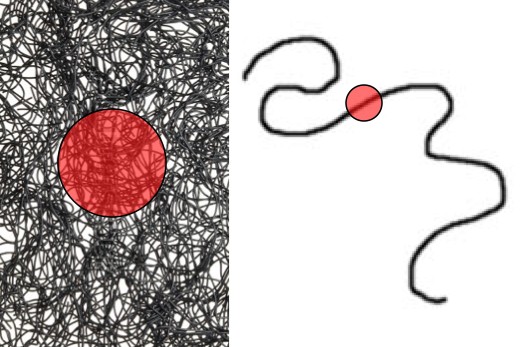
A “crumpled up” set seems like it has a higher dimension than it really does. You can get around this by using smaller and smaller spheres. Eventually you’ll get the correct dimension.
This whole “look at the number of points inside of tiny spheres and see how that number scales with size” thing works great for every half-way reasonable set. However, fractal sets can be “infinitely crumpled”, so no matter how small a sphere you use, you still get a dimension larger than you might expect.
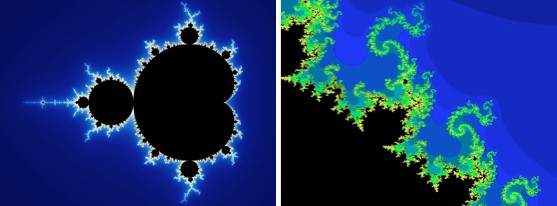
The edge of the Mandelbrot set “should” be one-dimensional since it’s just a line. However, it’s infinitely twisty, and no matter how much you zoom in it stays just as messed up.
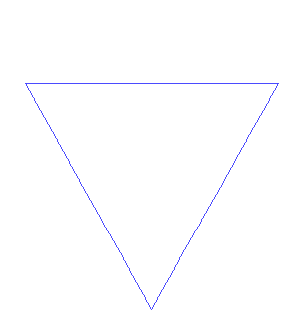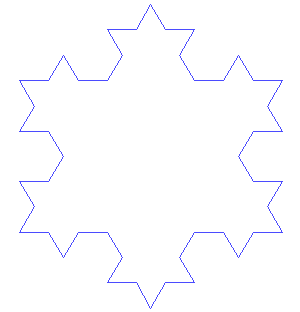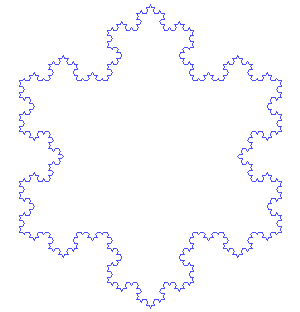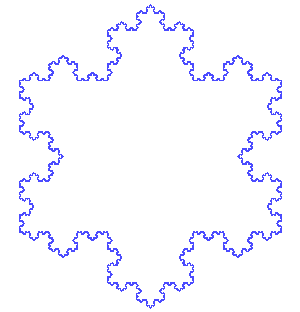
When the “sphere trick” is applied to tangled messes it doesn’t necessarily have to give you integer numbers until the spheres are small enough. With fractals there is no “small enough” (that should totally be a terrible movie tag line), and you find that they have a dimension that’s often a fraction. The dimension of the Mandelbrot’s boundary (picture above) is 2, which is the highest it can be, but there are more interesting (but less pretty) fractals out there with genuinely fractional dimensions, like the “Koch snowflake” which has a dimension of approximately 1.262.
That all said, when somebody (looking at you, all mathematicians) talks about fractional dimensions, they’re really talking about a weird, abstract, and not at all physical notion of dimension. There’s no such thing as “2.5 dimensional universe”. When we talk about the “dimension of space” we’re talking about the number of completely different directions that are available, not the whole “sphere thing”. The dimensions of space are either there or not, so while you could have 4 dimensions, you couldn’t have 3.5.







Please reply by commenting: How exactly can the Koch snowflake be 1.262 dimensional? If you drew it on paper, you could draw each stage of the fractal, and each stage would be two-dimensional!
Could you please reply so that an average high-school student can understand it, please?
Think of it this way: a fully two dimensional object would fill an infinite plane, and as far as we know, no such planes exist in reality.
Any simple geometric shape formed by a line (circle, square, triangle) fills a small part of an entire plane, so its dimension cannot be 2, it must be less.
We inherited whole number dimensions, lines, sphere’s, etc. from the ancients, who recognized this is a convenient conceptualization for calculating, but as far as we know, no such shapes actually exist – they are simply convenient representations we use for measuring and predicting.
The physicists claims: When we talk about the “dimension of space” we’re talking about the number of completely different directions that are available, not the whole “sphere thing”. The dimensions of space are either there or not, so while you could have 4 dimensions, you couldn’t have 3.5.
While it is true that this is what physicists mean when they talk about dimensions, I am unaware of any evidence that such conceptualizations have been tested, much less verified.
[The following may not be suitable for philosophy undergrads or physics post-docs.]
There are also good reasons to treat the common attribution of fundamental reality to physics concepts with skepticism. From the perspective of project management, such assumptions are a primary source of risk, and more than a century of inability to solve basic mysteries with GR suggest some assumptions upon which the model is based are the root causes. From a history and philosophy of science perspective, replacement of object concepts by process concepts is a litmus test of fundamentally transformative models, but this was not really ever completed with either QM or GR. Also: failure to document assumptions violates best practices for risk planning and control.
All in all, if fundamental physics were a government or business project, it would be considered a disastrous boondoggle, unable to even clearly define basics like time, mass, charge, energy, etc.
Cantor set has dimension lower than 1, because some locations are “forbidden”. Matter and energy also haves forbidden locations, which depend recursively on the allowed locations, so they have not integer dimensions.
The point is that it cannot be claimed, without proof, that “Every physical object is always 3-D”.
I have the same questions as the aforementioned three people.
Have a look at the video at http://www.youtube.com/watch?v=js9nIgHA5FU which explains the idea at a high school level!
Great explanation. using all of these dimensions metaphore, if might consider time being the axis upon which a higher dimensional entity is seen as differnt realities observable in 3D real world.
but what is real? only the now.
Is future probability part of this fractal? or could it be, a fifth dimension of this growing universe.
and if so, and we like to count our consciousness in, are we not ,by thinking it, manifesting it .
Are you certain that the three dimensions you experience in 3D are really integer dimensions? How can you tell due to your mind being within that frame of reference? What was Einstein talking about with spacetime. Is it indeed a Minkowski 3D space and 1D time?
Those are pretty bold assertions!
@me,
There are no forbidden locations.
If a vector set can be defined as superpositions of n orthogonal unit vectors, these set can be represented on a n dimensional space. If we talk about an axis called fractional dimension which is also orthogonal to other dimensions, what doer make it ‘fractional’?
Since I read Williams’ book on Chaos back in 2009, I understand the 1/2 dimension thing when it comes to vision. Humans have 2.5-D vision. We cannot see behind us, so towards and away are only half a dimension. I recently came across fractional (fractal) dimensions. I get it. What I don’t know is what to do with it. I have a dataset. I can chop the dataset up into areas having different densities that require different numbers of covers, so I end up with different fractional dimensions.
I haven’t read about statistical topology yet, but I’m wondering about how to put fractional dimensions to work. Any hints?
Also, I’m looking at a process that changes from hyperbolic to Euclidean to spherical, and wonder what a bit looks like in those geometries. In Euclidean, it would be a cube.
@Buck Field:
“Think of it this way: a fully two dimensional object would fill an infinite plane, and as far as we know, no such planes exist in reality.”
Yes, but disks of finite radii are classified by mathematicians as 2-dimensional, even though they only cover a finite area in the infinite plane. Also, an infinite plane has no need to exist in reality. Aperigons exist in geometry.
“Any simple geometric shape formed by a line (circle, square, triangle) fills a small part of an entire plane, so its dimension cannot be 2, it must be less.”
Yes, but it can be more than 1 since it cannot simple drawn with a line either.
“We inherited whole number dimensions, lines, sphere’s, etc. from the ancients, who recognized this is a convenient conceptualization for calculating, but as far as we know, no such shapes actually exist – they are simply convenient representations we use for measuring and predicting.”
By this definition, spheres aren’t 3-dimensional.
“The physicists claims: When we talk about the “dimension of space” we’re talking about the number of completely different directions that are available, not the whole “sphere thing”. The dimensions of space are either there or not, so while you could have 4 dimensions, you couldn’t have 3.5.
While it is true that this is what physicists mean when they talk about dimensions, I am unaware of any evidence that such conceptualizations have been tested, much less verified.”
Relativity.
“[The following may not be suitable for philosophy undergrads or physics post-docs.]
There are also good reasons to treat the common attribution of fundamental reality to physics concepts with skepticism. From the perspective of project management, such assumptions are a primary source of risk, and more than a century of inability to solve basic mysteries with GR suggest some assumptions upon which the model is based are the root causes. From a history and philosophy of science perspective, replacement of object concepts by process concepts is a litmus test of fundamentally transformative models, but this was not really ever completed with either QM or GR. Also: failure to document assumptions violates best practices for risk planning and control.
All in all, if fundamental physics were a government or business project, it would be considered a disastrous boondoggle, unable to even clearly define basics like time, mass, charge, energy, etc.”
Sure enough, we can choose to not attribute “fundamental” notions of reality to the physical world, but why would we?
@itay:
“Great explanation. using all of these dimensions metaphore, if might consider time being the axis upon which a higher dimensional entity is seen as differnt realities observable in 3D real world.
but what is real? only the now.
Is future probability part of this fractal? or could it be, a fifth dimension of this growing universe.
and if so, and we like to count our consciousness in, are we not ,by thinking it, manifesting it .”
Itay, these notions you’re presenting are not ideas of dimension at all. Your usage of the term “dimension” is incorrect. They have nothing to do with multiverses or realms of existence.
@Alper Said:
“If a vector set can be defined as superpositions of n orthogonal unit vectors, these set can be represented on a n dimensional space. If we talk about an axis called fractional dimension which is also orthogonal to other dimensions, what doer make it ‘fractional’?”
It means it only partially accounts for vectors in this new direction, I presume.
The easiest way to understand fractional dimensions is with the number line.
We can have a zero dimensional point (say the point “1”). No argument there. Then we could fill it with all the integers, positive and negative and have an infinity of points. This line is not really zero dimensional because we need one number to describe each point, but there again its not truly one dimensional either. I could then add all the rational numbers, still infinity but in some sense closer together, but there is still that infinity of irrationals (an even bigger infinity in fact) that we could fit in. Clearly any real line would be completely indistinguishable from the line of rationals so its hard to claim its zero dimensional, but its provably not continuous. If you include the irrationals then you will have (I think) a one dimensional object (I’m pretty sure) but that’s a deal of points.