Q: Why don’t “cheats” ever work on the uncertainty principle? What’s uncertain in the uncertainty principle?

Physicist: The Uncertainty Principle is often stated as “the position and momentum of a particle cannot be simultaneously and perfectly measured”.  Mathematically, it’s written as , which means that the product of the uncertainties in the position, x, and momentum, p, is greater than some (tiny) constant.  But the Uncertainty Principle, rather than being a statement about the techniques we use to make measurements, is about the nature of the particles themselves (this actually applies to everything, not just particles).

The way the uncertainty principle is generally stated it sounds as though our inability to measure the position and momentum simultaneously is a failing of imagination on our parts.  So “cheats” are often conceived to get around the Uncertainty Principle.  The cheat that gave rise to this post is:

An attempt to out-smart the uncertainty principle.

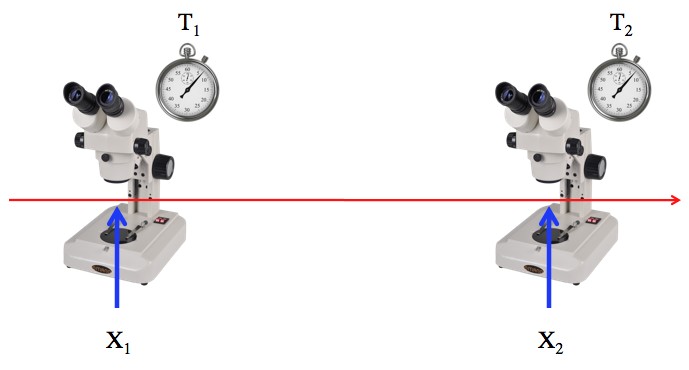
Say you have two instruments that can very accurately measure the location of a particle and the time when that particle was at that location, and a particle passes by each of them.  Both of them are strictly measuring the position and not momentum, so there shouldn’t be an “uncertainty issue”.  If you take the two (very exact) positions and times, then you can construct the speed of the particle, , and thus the momentum, .  Now, even if the first measurement screws up the momentum by accidentally giving the particle a kick during measurement, we still have a mechanism that can tell us the position and momentum immediately after the first measurement with arbitrary precision (which is no good according to the Uncertainty Principle).

However, in quantum mechanics, having something in a definite state does not mean that the results of your measurements will be certain, and this is where the real uncertainty shows up.  In this case you’ll find that if you prepare a string of in-every-way-identical particles and send them past the two detectors one at a time, then the measurements will not measure the same time difference.  Instead they’ll measure a range of different values (each individual measurement will fall within this range), and the size of this range is the uncertainty of the momentum (technically, the standard deviation of this range).

So, the power to do a single accurate measurement doesn’t have much to do with the Uncertainty Principle and doesn’t cause any problems.  But being able to repeat that set of perfect position and momentum measurements, secure in the knowledge (“certain” even) that each trial will have the same result, does violate the Uncertainty Principle.

Answering “why?” there’s no way to perfectly measure momentum and position is a bit more subtle.  Basically, the state of being in a particular position is composed of lots of momentum states, so when the momentum of a given “position state” is measured, you’ll catch any of the large number of momentum states, each of which gives you a different (uncertain) result.  More on that in the answer gravy below.

Answer gravy: This is the same essential point, but with more technical jargon.

The problem with position and momentum is that they are “conjugate variables” which means that their “measurement bases” are related in an obnoxious, Uncertainty making, kind of way.  Conjugate variables are hard to describe, so this sentence is the last time they’ll be mentioned.

This example is to demonstrate that there exists pairs of measurements that must always have uncertainties.

Light can be polarized at any angle.  A “polarizing beam splitter” is a piece of material that causes photons that are polarized in the one way to take one path, and perpendicularly polarized photons to take another path.  Some crystals are surprisingly good at this.

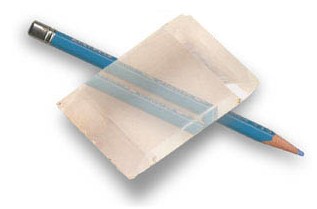
A calcite crystal sorts light based on its polarization.  Photons polarized “with the grain” go one way, and photons polarized “against the grain” (90° different) go another.  Photons that are polarized somewhere in between have a probability of going either way.

These crystals have molecules that tend to line up in a particular direction, and that gives them weird, direction-dependent, electrical properties which causes different polarizations to interact with them differently.  If a photon is polarized in the same direction that the crystal’s molecules are aligned (“with the grain”), then it will definitely take the first path.  If the photon is polarized perpendicular to the grain, then it will definitely take the second path.  In general, if it’s polarized at some angle θ to the grain, then the probability of it taking the first path is cos²(θ) and the probability of it taking the second path is 1-cos²(θ).  In particular, if it’s polarized at 45° then it has an even chance of going down either path.

Let’s say we’ve got a polarizing beam splitter arranged with the grain pointing straight up (0°).  This beam splitter can perfectly sort photons that are polarized at 0° or 90°.  We’ll call using this beam splitter an “A type” measurement.

Another polarizing beam splitter rotated so that the grain is tilted by 45° can perfectly sort photons polarized at 45° and 135° (or “45° and -45°” if you prefer).  We’ll call using this beam splitter a “B type” measurement.  The difference between the A and B type measurements is they have different “measurement bases”.

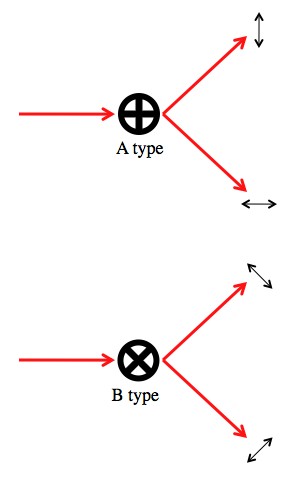
An “A type” measurement sends vertical and horizontal photons down different paths, but randomly assigns a path to diagonally polarized photons. The B type measurement does the opposite.

Now, lets say you create a set up to do an A type measurement, followed by a B type measurement.

This set up should do both types of measurements on a photon coming from the left by looking at what path it exits through.

But here’s the problem: the vertical polarization state, , is an equal combination of the diagonal states, and , and each of those diagonal states are an equal combination of the vertical and horizontal states, and .  This means that a vertically polarized photon will have a definite result from the A type measurement, but a completely random measurement from the B type measurement (either path with a 50/50 chance).  Similarly, a photon that has a definite result from the B type measurement will have a completely random result from the A type measurement.

So, if you repeat this experiment with a huge number of identically prepared photons, you’ll never be certain which of the four paths it will exit through.  They’ll always exit through at least two of the paths.  This is literally an uncertainty principle (though not one of the big ones, like position/momentum).

However, the source of this “path uncertainty principle” and the position/momentum Uncertainty Principle stem from the same source.  Here you can’t be sure of the measurement, because the states that give you a definite result for one measurement is made of a combination of states that give uncertain results for the other measurement.  E.g., the vertical state is exactly the same as a combination of the diagonal states, so it’ll give a definite result for the A type measurement, but since it’s some of both of the diagonal states it will yield either of the possible results from the B type measurement.

Position measurements and momentum measurements are similar.  The state of being at a particular position, a “position state”, is the same as a huge combination of momentum states that have different momenta (momentums?).  In exactly the same way, a particular momentum state is exactly the same as a particular combination of position states (this is not obvious, so don’t stress).  So, if you’ve set things up so that you have a definite, “certain”, result for one, then you’ve set things up so that you don’t have a definite result for the other, just like with the whole “A type / B type photon thingy”.  Unfortunately, there’s just no way around that.

The pencil picture is from here.