Physicist: As much of a trope as “Other Quantum Worlds” has become in sci-fi, there are reasons to think that they may be a real thing; including “other yous”. Here’s the idea.
Superposition is a real thing
One of the most fundamental aspects of quantum mechanics is “superposition“. Something is in a superposition when it’s in multiple states/places simultaneously. You can show, without too much effort, that a wide variety of things can be in a superposition. The cardinal example is a photon going through two slits before impacting a screen: the double slit experiment.
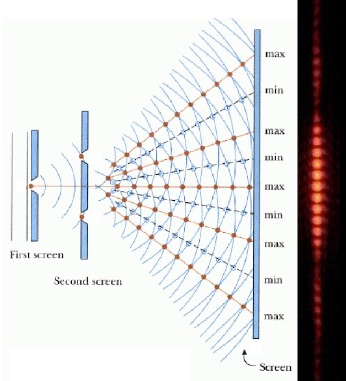
The infamous Double Slit experiment demonstrates a single photon going through two (or more) slits simultaneously. The “beats” of light are caused by photons interfering like waves between the two slits. This still works if you release one photon at a time; even individually, they’ll only hit the bright regions.
Instead of the photons going straight through and creating a single bright spot behind every slit (classical) we instead see a wave interference pattern (quantum). This only makes sense if: 1) the photons of light act like waves and 2) they’re going through both slits.
It’s completely natural to suspect that the objects involved in experiments like this are really in only one state, but have behaviors too complex for us to understand. Rather surprisingly, we can pretty effectively rule that out.
There is no scale at which quantum effects stop working
To date, every experiment capable of detecting the difference between quantum and classical results has always demonstrated that the underlying behavior is strictly quantum. To be fair, quantum phenomena is as delicate as delicate can be. For example, in the double slit experiment any interaction that could indicate to the outside world (the “environment”), even in principle, which slit the particle went through will destroy the quantumness of the experiment (the interference fringes go away). When you have to worry about the disruptive influence of individual stray particles, you don’t expect to see quantum effects on the scale of people and planets.
That said, the double slit experiment has been done and works for every kind of particle and even molecules with hundreds of atoms. Quantum states can be maintained for minutes or hours, so superposition doesn’t “wear out” on its own. Needles large enough to be seen with the naked eye have been put into superpositions of vibrational modes and this year China launched the first quantum communication satellite which is being used as a relay to establish quantum effects over scales of hundreds or thousands of miles. So far there is no indication of a natural scale where objects are big enough, far enough apart, or old enough that quantum mechanics simply cease to apply. The only limits seem to be in our engineering abilities. It’s completely infeasible to do experimental quantum physics with something as substantive as a person (or really anything close).
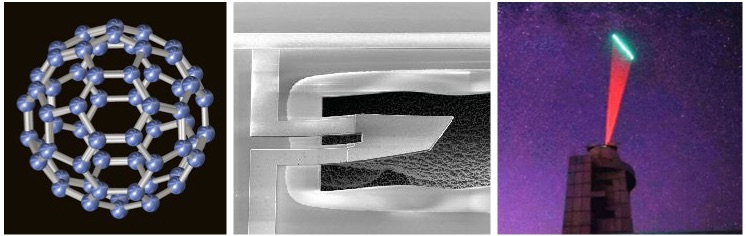
Left: Buckminsterfullerene (and even much larger molecules) interfere in double-slit experiments. Middle: A needle that was put into a superposition of literally both vibrating and not vibrating at all. Right: Time lapse of a laser being used to establish quantum entanglement with carefully isolated atoms inside of a satellite in orbit.
If the quantum laws did simply ceased to apply at some scale, then those laws would be bizarre and unique; the first of their kind. Every physical law applies at all scales, it’s just a question of how relevant each is. For example, on large scales gravity is practically the only force worth worrying about, but on the atomic scale it can be efficiently ignored (usually).
Io sticks to (orbits) Jupiter because of gravitational forces and styrofoam sticks to cats because of electrical forces. Both apply on all scales, but on smaller scales (evidently cat scale and below) electrical forces tend to dominate.
So here comes the point: if the quantum laws really do apply at all scales, then we should expect that exactly like everything else, people (no big deal) should ultimately follow quantum laws and exhibit quantum behavior. Including superposition. But that begs a very natural question: what does it feel like to be in multiple states? Why don’t we notice?
The quantum laws don’t contradict the world we see
When you first hear about the heliocentric theory (the Earth is in motion around the Sun instead of the other way around), the first question that naturally comes to mind is “Why don’t I feel the Earth moving?“. But in trying to answer that you find yourself trying to climb out of the assumption that you should notice anything. A more enlightening question is “What do the laws of gravitation and motion say that we should experience?“. In the case of Newton’s laws and the Earth, we find that because the surface of the Earth, the air above it, and the people on it all travel together, we shouldn’t feel anything. Despite moving at ridiculous speeds there are only subtle tell-tail signs that we’re moving at all, like ocean tides or the precession of garishly large pendulums.
Quantum laws are in a similar situation. You may have noticed that you’ve never met other versions of yourself wandering around your house. You’re there, so shouldn’t at least some other versions of you be as well? Why don’t we see them? A better question is “What do the quantum laws say that we should experience?“.
Why don’t we run into our other versions all the time, instead of absolutely never?
The Correspondence Principle is arguably one of the most important philosophical underpinnings in science. It says that whatever your theories are, they need to predict (or at the very least not contradict) ordinary physical laws and ordinary experience when applied to ordinary situations.
When you apply the laws of relativity to speeds much slower than light all of the length contractions and twin paradoxes become less and less important until the laws we’re accustomed to working with are more than sufficient. That is to say; while we can detect relativistic effects all the way down to walking speed, the effect is so small that you don’t need to worry about it when you’re walking.
Similarly, the quantum laws reproduce the classical laws when you assume that there are far more particles around than you can keep track of and that they’re not particularly correlated with one another (i.e., if you’re watching one air molecule there’s really no telling what the next will be doing). There are times when this assumption doesn’t hold, but those are exactly the cases that reveal that the quantum laws are there.
It turns out that simply applying the quantum laws to everything seems to resolve all the big paradoxes. That’s good on the one hand because physics works. But on the other hand, we’re forced into the suspicion that the our universe might be needlessly weird.
The big rift between “the quantum world” and “the classical world” is that large things, like you and literally everything that you can see, always seem to be in exactly one state. When we keep quantum systems carefully isolated (usually by making them very cold, very tiny, and very dark) we find that they exhibit superposition, but when we then interact with those quantum systems they “decohere” and are found to be in a smaller set of states. This is sometimes called “wave function collapse”, to evoke an image of a wide wave suddenly collapsing into a single tiny particle. The rule seems to be that interacting with things makes their behavior more classical.
But not always. “Wave function collapse” doesn’t happen when isolated quantum systems interact with each other, only when they interact with the environment (the outside world). Experimentally, when you allow a couple of systems that are both in a superposition of states to interact, then the result is two systems in a joint superposition of states (this is entanglement). If the rule were as simple as “when things interact they decohere” you’d expect to find both systems each in only one state after interacting. What we find instead is that in every testable case the superposition in maintained. Changed or entangled, sure, but the various states in a superposition never just disappear. When you interact with a system in a superposition you only see a particular state, not a superposition. So what’s going on when we, or anything in the environment, interacts with a quantum system? Where did the other states in the superposition go?
We have physical laws that describe the interactions between pairs of isolated quantum systems (A and B). When we treat the environment as another (albeit very big) quantum system we can continue to use those same laws. When we assume that the environment is not a quantum system, we have to make up new laws and special exceptions.
The rules we use to describe how pairs of isolated systems interact also do an excellent job describing the way isolated quantum systems interact with the outside environment. When isolated systems interact with each other they become entangled. When isolated systems interact with the environment they decohere. It turns out that these two effects, entanglement and decoherence, are two sides of the same coin. When we make the somewhat artificial choice to ask “What states of system B can some particular state in system A interact with?” we find that the result mirrors what we ourselves see when we interact with things and “collapse their wave functions” (see the Answer Gravy below for more on that). The phrase “wave function collapse” is like the word “sunrise”; it does a good job describing our personal experience, but does a terrible job describing the underlying dynamics. When you ask the natural question “What does it feel like to be in a many different states?” the frustrating answer seems to be “You tell me.“.
A thing can only be inferred to be in multiple states (such as by witnessing an interference pattern). If there’s any way to tell the difference between the individual states that make up a superposition, then (from your point of view) there is no superposition. Since you can see yourself, you can tell the difference between your state and another. You might be in an effectively infinite number of states, but you’d never know it. The fact that you can’t help but observe yourself means that you will never observe yourself somewhere that you’re not.
“Where are my other versions?” isn’t quite the right question
Where are those other versions of you? Assuming that they exist, they’re no place more mysterious than where you are now. In the double slit experiment different versions of the same object go through the different slits and you can literally point to exactly where each version is (in exactly the same way you can point at anything you can’t presently observe), so the physical position of each version isn’t a mystery.
The mathematical operations that describe quantum mechanical interactions and the passage of time are “linear”, which means that they treat the different states in a superposition separately. A linear operator does the same thing to every state individually, and then sums the results. There are a lot of examples of linear phenomena in nature, including waves (which are solutions to the wave equation). The Schrodinger equation, which describe how quantum wave functions behave, is also linear.
The wave equation is linear, so you can describe how each of these ways travels across the surface of the water by considering them each one at a time and adding up the results. They don’t interact with each other directly, but they do add up.
So, if there are other versions of you, they’re wandering around in very much the same way you are. But (as you may have noticed) you don’t interact with them, so saying they’re “in the same place you are” isn’t particularly useful. Which is frustrating in a chained-in-Plato’s-cave-kind-of-way.
The rain puddle picture is from here.
Answer Gravy: In quantum mechanics the affect of the passage of time and every interaction is described by a linear operator (even better, it’s a unitary operator). Linear operators treat everything they’re given separately, as though each piece was the only piece. In mathspeak, if 
Consider a quantum system that can be in either of two states, 
Let’s also say that the quantum scientists Alice and Bob can be described by the modest notation 
Define a linear “look” operation for Alice, 
and similarly for Bob
Applying these one at a time we see what happens when each looks at the quantum system; they end up seeing the same thing.
It’s subtle, but you’ll notice that the coefficient in front of this state is 1, meaning that it has a 100% of happening.
But what happens if the system is in a superposition of states, such as 
![\begin{array}{ll} &L_BL_A\left(|A(?)\rangle|B(?)\rangle\left(\frac{|\square\rangle+|\blacksquare\rangle}{\sqrt{2}}\right)\right) \\[2mm] =&\frac{1}{\sqrt{2}}L_BL_A\left(|A(?)\rangle|B(?)\rangle|\square\rangle\right)+\frac{1}{\sqrt{2}}L_BL_A\left(|A(?)\rangle|B(?)\rangle|\blacksquare\rangle\right) \\[2mm] =&\frac{1}{\sqrt{2}}L_B\left(|A(\square)\rangle|B(?)\rangle|\square\rangle\right)+\frac{1}{\sqrt{2}}L_B\left(|A(\blacksquare)\rangle|B(?)\rangle|\blacksquare\rangle\right) \\[2mm] =&\frac{1}{\sqrt{2}}|A(\square)\rangle|B(\square)\rangle|\square\rangle+\frac{1}{\sqrt{2}}|A(\blacksquare)\rangle|B(\blacksquare)\rangle|\blacksquare\rangle \\[2mm] \end{array}](http://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Bll%7D+%26L_BL_A%5Cleft%28%7CA%28%3F%29%5Crangle%7CB%28%3F%29%5Crangle%5Cleft%28%5Cfrac%7B%7C%5Csquare%5Crangle%2B%7C%5Cblacksquare%5Crangle%7D%7B%5Csqrt%7B2%7D%7D%5Cright%29%5Cright%29+%5C%5C%5B2mm%5D+%3D%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7DL_BL_A%5Cleft%28%7CA%28%3F%29%5Crangle%7CB%28%3F%29%5Crangle%7C%5Csquare%5Crangle%5Cright%29%2B%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7DL_BL_A%5Cleft%28%7CA%28%3F%29%5Crangle%7CB%28%3F%29%5Crangle%7C%5Cblacksquare%5Crangle%5Cright%29+%5C%5C%5B2mm%5D+%3D%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7DL_B%5Cleft%28%7CA%28%5Csquare%29%5Crangle%7CB%28%3F%29%5Crangle%7C%5Csquare%5Crangle%5Cright%29%2B%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7DL_B%5Cleft%28%7CA%28%5Cblacksquare%29%5Crangle%7CB%28%3F%29%5Crangle%7C%5Cblacksquare%5Crangle%5Cright%29+%5C%5C%5B2mm%5D+%3D%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%7CA%28%5Csquare%29%5Crangle%7CB%28%5Csquare%29%5Crangle%7C%5Csquare%5Crangle%2B%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%7CA%28%5Cblacksquare%29%5Crangle%7CB%28%5Cblacksquare%29%5Crangle%7C%5Cblacksquare%5Crangle+%5C%5C%5B2mm%5D+%5Cend%7Barray%7D&bg=ffffff&fg=000&s=0 "\begin{array}{ll} &L_BL_A\left(|A(?)\rangle|B(?)\rangle\left(\frac{|\square\rangle+|\blacksquare\rangle}{\sqrt{2}}\right)\right) \\[2mm] =&\frac{1}{\sqrt{2}}L_BL_A\left(|A(?)\rangle|B(?)\rangle|\square\rangle\right)+\frac{1}{\sqrt{2}}L_BL_A\left(|A(?)\rangle|B(?)\rangle|\blacksquare\rangle\right) \\[2mm] =&\frac{1}{\sqrt{2}}L_B\left(|A(\square)\rangle|B(?)\rangle|\square\rangle\right)+\frac{1}{\sqrt{2}}L_B\left(|A(\blacksquare)\rangle|B(?)\rangle|\blacksquare\rangle\right) \\[2mm] =&\frac{1}{\sqrt{2}}|A(\square)\rangle|B(\square)\rangle|\square\rangle+\frac{1}{\sqrt{2}}|A(\blacksquare)\rangle|B(\blacksquare)\rangle|\blacksquare\rangle \\[2mm] \end{array}")
From an extremely outside perspective, Alice and Bob have a probability of 
Anything else that happens can also be described by a linear operation (call it “F”) and therefore these two states can’t directly affect each other.
The states can both contribute to the same end result, but only for other systems/observers that haven’t interacted with either of Alice or Bob or their pet quantum system. We see this in the double slit experiment: the versions of the photons that go through each slit each contribute to the interference pattern on the screen, but neither version ever directly affects the other. “Contributing but not interacting” sounds more abstract than it is. If you shine two lights on the same object, the photons flying around all ignore each other, but each individually contributes to illuminating said object just fine.
The Alices and Bobs in the states 
Notice that Alice and Bob don’t see their own state being modified by that 
If you are determined to follow a particular state through the problem, ignoring the others, then you need to make this adjustment. The interaction operation starts to look more like a “projection operator” adjusted so that the resulting state is properly normalized.
The projection operator for the state 
An interaction with the system in the state 
Here comes the same example again, but in a more “wave function collapse sort of way”. We still start with the state 
![\begin{array}{ll} &M_\blacksquare\left(\frac{|\square\rangle+|\blacksquare\rangle}{\sqrt{2}}\right) \\[2mm] =& \frac{|\blacksquare\rangle\langle\blacksquare|\left(\frac{|\square\rangle+|\blacksquare\rangle}{\sqrt{2}}\right)}{\left|\langle\blacksquare|\left(\frac{|\square\rangle+|\blacksquare\rangle}{\sqrt{2}}\right)\right|} \\[2mm] =& \frac{|\blacksquare\rangle\left(\frac{\langle\blacksquare|\square\rangle+\langle\blacksquare|\blacksquare\rangle}{\sqrt{2}}\right)}{\left|\frac{\langle\blacksquare|\square\rangle+\langle\blacksquare|\blacksquare\rangle}{\sqrt{2}}\right|} \\[2mm] =& \frac{|\blacksquare\rangle\left(\frac{0+1}{\sqrt{2}}\right)}{\left|\frac{0+1}{\sqrt{2}}\right|} \\[2mm] =& \frac{|\blacksquare\rangle\frac{1}{\sqrt{2}}}{\frac{1}{\sqrt{2}}} \\[2mm] =& |\blacksquare\rangle \end{array}](http://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Bll%7D+%26M_%5Cblacksquare%5Cleft%28%5Cfrac%7B%7C%5Csquare%5Crangle%2B%7C%5Cblacksquare%5Crangle%7D%7B%5Csqrt%7B2%7D%7D%5Cright%29+%5C%5C%5B2mm%5D+%3D%26+%5Cfrac%7B%7C%5Cblacksquare%5Crangle%5Clangle%5Cblacksquare%7C%5Cleft%28%5Cfrac%7B%7C%5Csquare%5Crangle%2B%7C%5Cblacksquare%5Crangle%7D%7B%5Csqrt%7B2%7D%7D%5Cright%29%7D%7B%5Cleft%7C%5Clangle%5Cblacksquare%7C%5Cleft%28%5Cfrac%7B%7C%5Csquare%5Crangle%2B%7C%5Cblacksquare%5Crangle%7D%7B%5Csqrt%7B2%7D%7D%5Cright%29%5Cright%7C%7D+%5C%5C%5B2mm%5D+%3D%26+%5Cfrac%7B%7C%5Cblacksquare%5Crangle%5Cleft%28%5Cfrac%7B%5Clangle%5Cblacksquare%7C%5Csquare%5Crangle%2B%5Clangle%5Cblacksquare%7C%5Cblacksquare%5Crangle%7D%7B%5Csqrt%7B2%7D%7D%5Cright%29%7D%7B%5Cleft%7C%5Cfrac%7B%5Clangle%5Cblacksquare%7C%5Csquare%5Crangle%2B%5Clangle%5Cblacksquare%7C%5Cblacksquare%5Crangle%7D%7B%5Csqrt%7B2%7D%7D%5Cright%7C%7D+%5C%5C%5B2mm%5D+%3D%26+%5Cfrac%7B%7C%5Cblacksquare%5Crangle%5Cleft%28%5Cfrac%7B0%2B1%7D%7B%5Csqrt%7B2%7D%7D%5Cright%29%7D%7B%5Cleft%7C%5Cfrac%7B0%2B1%7D%7B%5Csqrt%7B2%7D%7D%5Cright%7C%7D+%5C%5C%5B2mm%5D+%3D%26+%5Cfrac%7B%7C%5Cblacksquare%5Crangle%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%7D%7B%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%7D+%5C%5C%5B2mm%5D+%3D%26+%7C%5Cblacksquare%5Crangle+%5Cend%7Barray%7D&bg=ffffff&fg=000&s=0 "\begin{array}{ll} &M_\blacksquare\left(\frac{|\square\rangle+|\blacksquare\rangle}{\sqrt{2}}\right) \\[2mm] =& \frac{|\blacksquare\rangle\langle\blacksquare|\left(\frac{|\square\rangle+|\blacksquare\rangle}{\sqrt{2}}\right)}{\left|\langle\blacksquare|\left(\frac{|\square\rangle+|\blacksquare\rangle}{\sqrt{2}}\right)\right|} \\[2mm] =& \frac{|\blacksquare\rangle\left(\frac{\langle\blacksquare|\square\rangle+\langle\blacksquare|\blacksquare\rangle}{\sqrt{2}}\right)}{\left|\frac{\langle\blacksquare|\square\rangle+\langle\blacksquare|\blacksquare\rangle}{\sqrt{2}}\right|} \\[2mm] =& \frac{|\blacksquare\rangle\left(\frac{0+1}{\sqrt{2}}\right)}{\left|\frac{0+1}{\sqrt{2}}\right|} \\[2mm] =& \frac{|\blacksquare\rangle\frac{1}{\sqrt{2}}}{\frac{1}{\sqrt{2}}} \\[2mm] =& |\blacksquare\rangle \end{array}")
That is to say, the superposition suddenly becomes only the measured state while the other states suddenly vanish (by some totally unknown means). After this collapse, the “look” operators function normally.
This “measurement operator” (which does all the collapsing) is definitively non-linear, which is a big red flag. We never see non-linear operations when we study isolated sets of quantum systems, no matter how they interact. The one and only time we see non-linear operations is when we include the environment and even then only when we assume that there’s something unique and special about the environment. When you assume that literally everything is a quantum system capable of being in superpositions of states the quantum laws become ontologically parsimonious (easy to write down). We lose our special position as the only version of us that exists, but we gain a system of physical laws that doesn’t involve lots of weird exceptions, extra rules, and paradoxes.
For someone who claims to be “The Physicist” to shamelessly flaunt this kind of unfalsifiable speculative fiction as if it was mainstream scientific consensus contributes nothing but froth to the frappuccino of YouTube pop science.
The short answer is ‘we don’t know’.
Physicists sometimes have trouble saying that, rather in the way that tough men sometimes have trouble saying ‘I love you’.
But in both cases, it’s still true.
I’m not sure I am following. Are you stating the many-world interpretation is FACTUALLY TRUE as contrasted with “still controversial or undecided”.
Besides, this deeply inside QM the words “as it appears to us”, “as perceived” should be liberally applied.
– greg
What if Myself of one of these universes decided to travel through space and had the technology or some how got lost in a worm hole or something and ended up in our current universe and interacted with me in this universe?
Why cat scale and below?
Electromagnetics can be larger than cats and hold up entire cars overcoming the entire earth’s gravity.. ?
@John Horsley
Then car scale and below, evidently. The important point is that on very large scales gravity is important and on very small scales it can be ignored, which gives the (false) impression that there are different laws in play at different scales.
“The exact same mathematics that predicts that other versions of us exist…”
There’s no mathematics that predicts that other versions of us exist, and very few physicists would say that there is. This is nonsense.
There’s just some mathematics that is very, very widely seen as uninterpreted. There are plenty of different interpretations for the mathematics, but many of us think they’re all unsatisfactory. You can’t just take your favourite interpretation and say that the mathematics predicts it.
Sad when even the science blogs you follow start to be post-truth.
The Parable of Schrodinger’s Cat and the Law :
https://goo.gl/5XDpkQ
The ‘other’ you’s if they exist in some sense are irrelevant to you.
Quantum Mechanics and quantum effects have resulted from lack of true understanding and interpreting of natural laws. They are figments of someones imagination, science fiction rather than science.
Rather than admitting that they don’t fully understand and account for all the variables and participants of the system, the so called scientists make up fictional and twisted realities that exist conveniently just beyond our physical perceptions, at scales too small or too large for direct observation.
It’s all nonsense and “fake” science in my opinion.
Tough crowd on this one. I just wanted to clarify that you are not explicitly talking about the many worlds interpretation of quantum mechanics but rather talking about the concept of superposition and how the ideas and evidence around it prevents separate but superimposed states from ever interacting with each other?
@Hasan
No kidding! And that’s exactly right.
The MWI involves some extra rules concerning that non-interaction that I’ve never seen evidence/need for.
Well I’d say that if you’re not talking about the many words interpretation, it should have been said in the article, as it’s very misleading.
But if you’re talking about superposition, and I hope I’ve understood correctly, it seems to me that another thing it might have been ‘a good idea to mention’, is that in that context the copies last less than 10^-17 seconds before the superposition decoheres (in this world), so those “other mes” only exist in the way you talk about, if you go back to…. the many worlds interpretation.
@David Martin
The approach taken in the article is basically “relational quantum mechanics” which is, in a nutshell, “apply the rules to everything” and “be very careful when you consider perspective”. Part of that is that anything/everything can be in a superposition, which you can read as “many worlds”, but “The Many Worlds Interpretation” (with Capitalization!) itself carries some extra ideas that I think may not be necessary.
Where does the “10^-17 seconds” come from?
The guy who asked the original question about ‘other mes’ was on Earth. Decoherence means that large scale objects like that guy lose the superposition by entanglements being created with their environment in a very short time. The larger the object, the quicker it happens, and objects far smaller than him lose the superposition in around 10^-17 seconds. RQM doesn’t contradict that, are you saying it does? Although it’s true that anything/everything can be in a superposition, it’s not for long.
@David Martin
It does. After 10^(-17)s only objects within about 3 nanometers (the distance light travels in that time) can know about a given event. Things outside of an event’s light cone are perfectly isolated from that event.
The entanglement you mentioned is a property shared between two systems which are both in a superposition of states, but correlated. So when you become entangled with something it doesn’t mean that some states have gone away, it means that some states can only see some other states. For example, when you check to see if a radioactive decay has occurred (with a geiger counter or something) you and the atom become entangled. Your collective superposition of states becomes “it decayed and you know that it decayed” along with “it didn’t decay and you know it didn’t”. Both versions see decoherence, they just disagree on the end result.
I get the impression that this post was not initially well-received. Here’s what I think I did wrong: I wrote this as though I’m in a place to make up your mind for you. I did try to make it “flashy” and in the process ended up making some pretty broad claims. “There are literally different versions of you…” may not have been the best start.
I’ve reorganized and rewritten it, included some relevant support and citations, and added an Answer Gravy with some of the math behind the physics. This is a very math-heavy subject and nothing’s perfect, so if you see something that’s wrong, needs clarifying, or is just too big a jump, let me know.
Yay! It’s back! I wondered where this post went for a while.
You’re always going to get people complaining about QM; it’s so inherently illogical that the average layman just refuses to accept it. The fact that it’s mostly only ‘relevant’ (using that term extremely broadly) at scales too small for most people to care about makes the matter worse.
Because apparently we just assume that the universe should be logical… For… Some reason?
I think the problem is how we teach it. We keep telling students “it’s strange, it can’t be understood, if you think you understand it you’re wrong, and so on. This poisons the fertile minds one of which will eventually say “QM is totally understandable”.
Consider this: you can explain/describe QM with Schrodinger’s equation, but that equation is TOTTALY classical and continuous. Einsteing said “Common sense is nothing but a set of prejudices we acquire in childhood and then add to them.
It’s not QM that’s strange, it’s CLASSICAL PHYSICS that is bizarre. QM makes perfect sense once you discard your prejudices and accept QM for what it is.
It’s akin to telling EVERYONE that the fact that heavy and light bodies fall at the same speed is counter-intuitive and hard to understand. No, it’s NOT. I can demonstrate that to anyone in a few paragraphs. All you have to do is drop your prejudices and use proper science instead.
Indeed, the primary issue with Quantum Mechanics though is that there are a lot of really strange questions that crop up to which the answer given typically ends up being ‘yes that is actually how it works.’
When the answer that really should be given is ‘Yes that is how it works, but that doesn’t mean what you think it means.’
This post is a pretty good example of that; yes, there are other ‘versions’ of you according to Quantum Mechanics, but that doesn’t mean what you think it means.
Suppose that I perform the double slit experiment with a detector. If the photon goes through the left slit, I will go to the sushi place on the west side of town for dinner. If the photon goes through the right slit, I will go to the pizza place on the east side of town.
The two resulting ‘mes’ occupy widely separated spaces. Each particle in my body has split into two particles. Doesn’t this violate conservation of energy?
@MattF
You’re right, if every event resolved itself by splitting the universe, or even individual particles, the conservation of energy would be torn to shreds. We’d barely have a reason to suspect that it’s a thing at all.
But being in a superposition of states does not mean that a thing is duplicated. In the double slit experiment a single particle goes through multiple slits, but it’s still a single particle. There’s a post here that tries to go into why energy is conserved.
The experiment you describe is a totally reasonable one that you can actually do. If you do, you’ll find there’s only one of you and energy is conserved (both versions can agree on that).
So, to be clear, you are saying that rather than both mes being made up of separate particles, we are made of the same particles in a superposition? By this point that seems like a meaningless distinction. A tiny particle having some uncertainty in position seems far different than it being in widely separated positions all with the same energy.
@MattF
It is deeply weird. But this isn’t just about small amounts of uncertainty, you can demonstrate interference on massive scales. There doesn’t seem to be any limit on how far apart different versions of a particle can be before they’re brought back together to interfere. The double slit is limited by the geometry of the set up to have the slits no more than a couple of wavelengths apart, but you can do analogous experiments with beam splitters and mirrors over many miles. LIGO for example.
Thanks for your responses. How does this all jive with gravity? Suppose in the early universe, a quantum event caused the sun to be in its current position, but the quantum event also resolved itself to cause the sun to be 1 light year away in another ‘branch’ of the wavefunction. Wouldn’t such extra stars cause spacetime to be bent in their location, thus causing a gravitational effect? Or is spacetime itself somehow splitting?
@MattF
I wonder about exactly the same thing myself. My guess (guess) is that spacetime can be in a superposition of different curvatures and shapes, but this isn’t an experiment that’s been done.
Everything else can be in superposition, why should space be exempt?
Hi babe, love your blog, just pointing a few typos in this post: (a) Answer gravy, paragraph three, the blank square should be black, and (b) ctrl+f “$” and you’ll see a coding error regarding the Bra-Ket notation for inner product.
Just passing by, love everything you write, and I appreciate so much what you do for the physics community and furthering public knowledge <3
There’s an erros in one of the equations in the paragraph that begins “The projection operator for the state”. It’s showing the code that should have been turned into an equation but wasn’t.
@José Abarzúa R. and @Alexander Gieg
Thanks!
There’s nothing special about either state, so in that particular paragraph it doesn’t matter. But it does read better with the change.
Yes there is another you.
It’s me.
And so is every posters/replier/reader/non-reader/:p.
We are all the same energy (and I believe it permeates through the entire universe, [ if you want to call it a soul]). The only information we can pass on though, is when we die, through our DNA.
If you are looking for someone that LOOKS like you and farts the same style ; I HAVE NO CLUE!
Atom wise – [note taking / general interest] so far reveals 2 days to 2 weeks to replace all your atoms in your body. So you are literally a different person from a month ago. I’m guessing it is closer to the 2 days model if you follow the American diet (eat, drink to excess).
From what I gather; 2/3 of atoms exit you via breathing, 1/3 urine and feces (a small extent is sweating).
Molecular / DNA wise
I’ll expand of this if there is interest. But I’m sure some of you have your own ideas. I will segway this in: YOU ARE what YOU ATE.
You were that chicken, you were that egg, that pig that became bacon, all those leaves in that leafy salad. etc.
You are not the energy that bounces around the universe; You are the energy of the universe. Our brains separate us but our DNA will pass on localized attained traits (example larger lungs) via reproduction.
Anecdotal – there are stories out there of donor receptiants taking on traits and sometimes the memories (ie: combination of locks, favorite school teachers, last moment of death events ) of the deceased donors. This is not really possible in the way I understand religion, but is possible if we were all the same ‘soul’ (for lack of a better description) energy.