Physicist: Nope! You definitely can’t.
Almost without exception, when you hear about quantum entanglement it’s described as some kind of communication or connection. Generally along the lines of “when you affect one entangled particle it instantly affects the other” or slightly more sophisticated “when you measure the state of one entangled particle, the other collapses to the same state”. But these statements cause more difficulties than a dark room full of loose rope. They’ve given rise to an endless parade of universally unsuccessful theories about using entanglement to communicate instantaneously between distant locations. But instantaneous communication has issues. In particular “instantaneous” is faster than c (the speed of light), which is the fastest speed. Ultimately, while entangled quantum systems do have plenty of seriously weird properties, being connected to each other is not one of them.
For the most part, entangled particles are like a pair of carefully boxed mail-order coins with the same (random) side up. If you and a friend shared a pair of these “coin boxes”, you’d find it difficult to tell whether or not your friend had seen their coin without asking.
In very short: if you do the same measurement on each of a pair of entangled particles, the two measurements will produce the same, random result. But that doesn’t involve any kind of signal bouncing around the universe. The distant entangled particle is like a lost wallet: there’s no way to tell if someone’s seen it until they call you.
Quick aside: Entanglement takes many forms, not just “always the same” (e.g., “always opposite” is an option). If you can think of a property of a particle or other quantum system, then you’re thinking of something that can be entangled: particle spin (up vs. down), photon polarization (vertical vs. horizontal), particle position (left path vs. right path), energy levels (excited vs. ground), even existence (is vs. isn’t). Entanglement is fun stuff.
Quantum systems can be in multiple states at the same time. For example, an electron can be both spin up and spin down. The question “which is it: up or down?” genuinely doesn’t make sense, because the electron’s state can be a combination of both. We call such combinations “superpositions”. Quantum superpositions are famously delicate. You can demonstrate that an electron is in multiple states, but you have to be clever about it, because if you ever directly measure whether the electron is spin up or down, you always find that it’s one or the other, but never both. Which single state you find is fundamentally random. This “effect” of measurement, where a superposition of several states is suddenly replaced by only one of those states, is called “decoherence” or “wave function collapse” or (when you’re in a hurry) “collapse”.
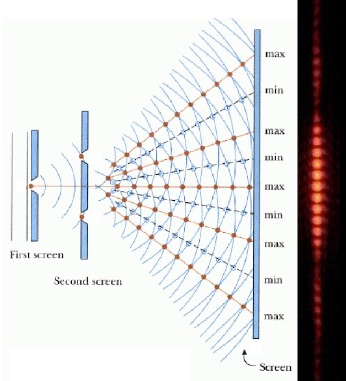
The oldest method to infer the superposition of states is the double slit experiment. Particles of any kind demonstrate that they can go through both slits (are so are in a superposition of both top path and bottom path) since the two paths/states interfere to create a pattern. But if you ever directly check, you only see particles going through one slit or the other. There are similar experiments for spin.
If a pair of electrons share a common state (for example by both being up and both being down, but never one up / one down), then those electrons are “entangled”. A pair of entangled particles are in a superposition of states together.
Entanglement isn’t something that happens accidentally. Or rather, it happens all the time (almost every interaction on every level generates entanglements), but useful entanglement is really hard to set up. It’s like the fact that practically everything makes sound, but the dulcet tones of a barbershop quartet takes work (and three friends). In order for two things to be entangled they need to have interacted with each other, so you can’t just cause two distant particles to become entangled. Generally speaking, you entangle two particles when they’re together, then move them apart.
When a single particle in a superposition of states is measured, it collapses to one of the states in its superposition. Entangled particles have the same rule; when you measure either of them you find that they’re in only one state. But since they’re in a shared state, a measurement on the other will yield the same result. A direct measurement is made, so the superposition (both up and both down) collapses and only a single state is left (either both up or both down). The fact that there are two particles involved instead of one doesn’t change things.
Einstein infamously quipped that entanglement is “spooky action at a distance” because, despite the fact that the result of each collapse is absolutely random, when it happens to a pair of entangled particles, they still manage to agree. That would seem to imply that they’re somehow telling each other how to behave (even if they would need to do so across any barrier or even faster than light).
Tricks that use entanglement to instantly send information generally involve collapsing the state of the distant particle (by measuring your own), and assuming that the person in charge of that particle will somehow notice. Unfortunately (and this is the answer), there’s no “new-particle-smell” for particles that are still in superpositions and there’s no big flash when that superposition collapses. If you measure an electron’s spin the result is simply “spin up” or “spin down”. That’s all you get. But that doesn’t tell you what the state was before, or if the original state was a superposition of several states, or even if the particle was entangled with something else.
If you only have access to one particle, then the two situations, 1) the other particle is inaccessible or 2) it has been quietly measured, are indistinguishable in every physical sense. Regardless of whatever else is involved, you can never notice the weirdness of entangled pairs until you directly compare them with each other.
The fact that you can’t tell the difference between a random state and an entangled state is super not obvious. In fact, the Fundamental Fysiks Group (who aren’t physicists so much as parapsychologists) once came up with a clever method for using entanglement for faster-than-light communication, that wasn’t clearly non-sense. Normally the way we determine that something is in multiple states is to prepare many identical versions and test them out, so the Fundamental Fysikists proposed copying one of the entangled particles and doing tests on the ensemble. In theory, Alice could communicate one bit of information by either looking (collapse) or not looking (no collapse) at her particle, and if Bob copied his particle many times and compared their behavior, he would be able to tell the difference. Technically, Bob might be able to tell the difference before Alice bothered to look, which is… suspicious (such is the nature of instantaneous communication in a universe where special relativity holds sway).
Faced with such daunting bizarritudes and paradoxes, professional physicists decided to take notice and “fix” the issue by carefully examining all of the assumptions that went into this cute trick (evidently, doing physics carefully is what separates physicists from fysiksists). It turns out that copying a quantum state is impossible and if it weren’t for the Fundamental Fysiks Group, we wouldn’t have had figured it out quite as soon as we did. The result of that figuring is the “no-cloning” or “better luck next time Fysikists” theorem.
Answer Gravy: A cute way to prove to yourself that superposition can’t be measured is to notice that “superposition-ness” is subjective. That is to say, a given quantum state can be a single state from one perspective and a superposition of several states from another. This is easiest to picture with polarized light.
A “measurement basis” is the set of states that your measurement apparatus can distinguish. For example, if you’re using a vertical polarizing filter, then you can distinguish between light that is vertically polarized (
But light can be polarized at any angle (there’s nothing special about verticalness). So what happens when diagonally polarized light hits a vertical filter? It turns out that diagonally polarized light, 
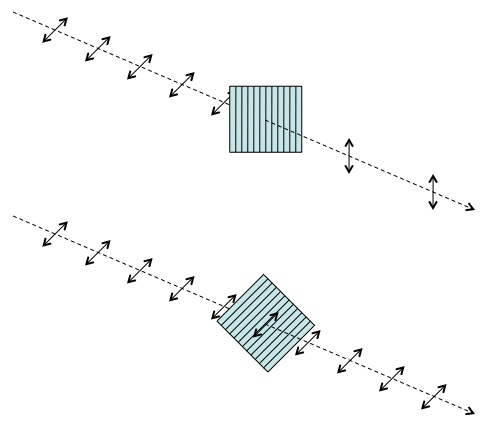
Top: When diagonally polarized light hits a vertical filter, its state randomly collapses to either vertical (it passes through) or horizontal (it stops). This is because diagonally polarized light is a superposition of both vertical and horizontal states. Bottom: On the other hand, it always passes through a similarly diagonal filter. For such a filter, the photons are in a single state. No collapse.
However! A diagonal filter measures photon polarization in the 
Brace yourself for the point. The same state,
Slightly Deeper Gravy: Entangled particles are nothing special. An entangled state is a regular, dull as dishwater, quantum state, it’s just that it’s shared between multiple particles. There actually is a “correct” basis in which you always get definite results from entangled particles, but you need all of the involved particles in one place to do such a measurement. Without all the pieces, you always get random results.
A diagonal state owned by Alice looks like this: 
Notice that the converse is also true. 
But for entangled states, if you only have access to one of the two particles, there is no “correct basis” where you always get a definite result.
A nice, tidy entangled state distributed between Alice and Bob is 
![\begin{array}{ll} &\frac{1}{\sqrt{2}}\left(|\rightarrow\rangle_a|\rightarrow\rangle_b+|\uparrow\rangle_a|\uparrow\rangle_b\right)\\[2mm] =&\frac{1}{\sqrt{2}}\left(\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_a-|\nwarrow\rangle_a\right)\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_b-|\nwarrow\rangle_b\right)+\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_a+|\nwarrow\rangle_a\right)\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_b+|\nwarrow\rangle_b\right)\right)\\[2mm] =&\frac{1}{2\sqrt{2}}\left(\left(|\nearrow\rangle_a-|\nwarrow\rangle_a\right)\left(|\nearrow\rangle_b-|\nwarrow\rangle_b\right)+\left(|\nearrow\rangle_a+|\nwarrow\rangle_a\right)\left(|\nearrow\rangle_b+|\nwarrow\rangle_b\right)\right)\\[2mm] =&\frac{1}{2\sqrt{2}}\left(\begin{array}{l}|\nearrow\rangle_a|\nearrow\rangle_b-|\nearrow\rangle_a|\nwarrow\rangle_b-|\nwarrow\rangle_a|\nearrow\rangle_b+|\nwarrow\rangle_a|\nwarrow\rangle_b\\+|\nearrow\rangle_a|\nearrow\rangle_b+|\nearrow\rangle_a|\nwarrow\rangle_b+|\nwarrow\rangle_a|\nearrow\rangle_b+|\nwarrow\rangle_a|\nwarrow\rangle_b\end{array}\right)\\[2mm] =&\frac{1}{2\sqrt{2}}\left(2|\nearrow\rangle_a|\nearrow\rangle_b+2|\nwarrow\rangle_a|\nwarrow\rangle_b\right)\\[2mm] =&\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_a|\nearrow\rangle_b+|\nwarrow\rangle_a|\nwarrow\rangle_b\right)\\[2mm] \end{array}](http://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Bll%7D++%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C%5Crightarrow%5Crangle_a%7C%5Crightarrow%5Crangle_b%2B%7C%5Cuparrow%5Crangle_a%7C%5Cuparrow%5Crangle_b%5Cright%29%5C%5C%5B2mm%5D++%3D%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C%5Cnearrow%5Crangle_a-%7C%5Cnwarrow%5Crangle_a%5Cright%29%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C%5Cnearrow%5Crangle_b-%7C%5Cnwarrow%5Crangle_b%5Cright%29%2B%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C%5Cnearrow%5Crangle_a%2B%7C%5Cnwarrow%5Crangle_a%5Cright%29%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C%5Cnearrow%5Crangle_b%2B%7C%5Cnwarrow%5Crangle_b%5Cright%29%5Cright%29%5C%5C%5B2mm%5D++%3D%26%5Cfrac%7B1%7D%7B2%5Csqrt%7B2%7D%7D%5Cleft%28%5Cleft%28%7C%5Cnearrow%5Crangle_a-%7C%5Cnwarrow%5Crangle_a%5Cright%29%5Cleft%28%7C%5Cnearrow%5Crangle_b-%7C%5Cnwarrow%5Crangle_b%5Cright%29%2B%5Cleft%28%7C%5Cnearrow%5Crangle_a%2B%7C%5Cnwarrow%5Crangle_a%5Cright%29%5Cleft%28%7C%5Cnearrow%5Crangle_b%2B%7C%5Cnwarrow%5Crangle_b%5Cright%29%5Cright%29%5C%5C%5B2mm%5D++%3D%26%5Cfrac%7B1%7D%7B2%5Csqrt%7B2%7D%7D%5Cleft%28%5Cbegin%7Barray%7D%7Bl%7D%7C%5Cnearrow%5Crangle_a%7C%5Cnearrow%5Crangle_b-%7C%5Cnearrow%5Crangle_a%7C%5Cnwarrow%5Crangle_b-%7C%5Cnwarrow%5Crangle_a%7C%5Cnearrow%5Crangle_b%2B%7C%5Cnwarrow%5Crangle_a%7C%5Cnwarrow%5Crangle_b%5C%5C%2B%7C%5Cnearrow%5Crangle_a%7C%5Cnearrow%5Crangle_b%2B%7C%5Cnearrow%5Crangle_a%7C%5Cnwarrow%5Crangle_b%2B%7C%5Cnwarrow%5Crangle_a%7C%5Cnearrow%5Crangle_b%2B%7C%5Cnwarrow%5Crangle_a%7C%5Cnwarrow%5Crangle_b%5Cend%7Barray%7D%5Cright%29%5C%5C%5B2mm%5D++%3D%26%5Cfrac%7B1%7D%7B2%5Csqrt%7B2%7D%7D%5Cleft%282%7C%5Cnearrow%5Crangle_a%7C%5Cnearrow%5Crangle_b%2B2%7C%5Cnwarrow%5Crangle_a%7C%5Cnwarrow%5Crangle_b%5Cright%29%5C%5C%5B2mm%5D++%3D%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C%5Cnearrow%5Crangle_a%7C%5Cnearrow%5Crangle_b%2B%7C%5Cnwarrow%5Crangle_a%7C%5Cnwarrow%5Crangle_b%5Cright%29%5C%5C%5B2mm%5D++%5Cend%7Barray%7D&bg=ffffff&fg=000&s=0 "\begin{array}{ll} &\frac{1}{\sqrt{2}}\left(|\rightarrow\rangle_a|\rightarrow\rangle_b+|\uparrow\rangle_a|\uparrow\rangle_b\right)\\[2mm] =&\frac{1}{\sqrt{2}}\left(\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_a-|\nwarrow\rangle_a\right)\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_b-|\nwarrow\rangle_b\right)+\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_a+|\nwarrow\rangle_a\right)\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_b+|\nwarrow\rangle_b\right)\right)\\[2mm] =&\frac{1}{2\sqrt{2}}\left(\left(|\nearrow\rangle_a-|\nwarrow\rangle_a\right)\left(|\nearrow\rangle_b-|\nwarrow\rangle_b\right)+\left(|\nearrow\rangle_a+|\nwarrow\rangle_a\right)\left(|\nearrow\rangle_b+|\nwarrow\rangle_b\right)\right)\\[2mm] =&\frac{1}{2\sqrt{2}}\left(\begin{array}{l}|\nearrow\rangle_a|\nearrow\rangle_b-|\nearrow\rangle_a|\nwarrow\rangle_b-|\nwarrow\rangle_a|\nearrow\rangle_b+|\nwarrow\rangle_a|\nwarrow\rangle_b\\+|\nearrow\rangle_a|\nearrow\rangle_b+|\nearrow\rangle_a|\nwarrow\rangle_b+|\nwarrow\rangle_a|\nearrow\rangle_b+|\nwarrow\rangle_a|\nwarrow\rangle_b\end{array}\right)\\[2mm] =&\frac{1}{2\sqrt{2}}\left(2|\nearrow\rangle_a|\nearrow\rangle_b+2|\nwarrow\rangle_a|\nwarrow\rangle_b\right)\\[2mm] =&\frac{1}{\sqrt{2}}\left(|\nearrow\rangle_a|\nearrow\rangle_b+|\nwarrow\rangle_a|\nwarrow\rangle_b\right)\\[2mm] \end{array}")
Holy crap! It’s essentially the same state in the diagonal basis! Once again, it’s perfectly random (50% chance of either result). More generally, as long as you only have one particle, the results are just as random in any basis. It turns out this is one of those universal truths of entangled states: it doesn’t matter how you measure them, if you’re only looking at one particle, you’re using the “wrong” basis.
On the other hand, if you have both particles, you can tell the difference between the four “maximally entangled states“. Each particle’s state is described by a qubit (a “quantum bit”). First, perform a controlled not gate (CNot) on the two entangled qubits, then apply a Hadamard gate (H) on the control qubit, then measure both.
Controlled Not does this:
Hadamard does this:
In the case of photon polarization, the Hadamard gate is a rotation that switches between the vertical/horizontal basis and the diagonal basis.
Here’s what happens to the entangled state this post keeps talking about:
![\begin{array}{rl}&\frac{1}{\sqrt{2}}\left(|0\rangle_a|0\rangle_b+|1\rangle_a|1\rangle_b\right)\\[2mm]\xrightarrow{CNot}&\frac{1}{\sqrt{2}}\left(|0\rangle_a|0\rangle_b+|1\rangle_a|0\rangle_b\right)\\[2mm]=&\frac{1}{\sqrt{2}}\left(|0\rangle_a+|1\rangle_a\right)|0\rangle_b\\[2mm]\xrightarrow{H}&|0\rangle_a|0\rangle_b\end{array}](https://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Brl%7D%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C0%5Crangle_a%7C0%5Crangle_b%2B%7C1%5Crangle_a%7C1%5Crangle_b%5Cright%29%5C%5C%5B2mm%5D%5Cxrightarrow%7BCNot%7D%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C0%5Crangle_a%7C0%5Crangle_b%2B%7C1%5Crangle_a%7C0%5Crangle_b%5Cright%29%5C%5C%5B2mm%5D%3D%26%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C0%5Crangle_a%2B%7C1%5Crangle_a%5Cright%29%7C0%5Crangle_b%5C%5C%5B2mm%5D%5Cxrightarrow%7BH%7D%26%7C0%5Crangle_a%7C0%5Crangle_b%5Cend%7Barray%7D&bg=ffffff&fg=000000&s=0 "\begin{array}{rl}&\frac{1}{\sqrt{2}}\left(|0\rangle_a|0\rangle_b+|1\rangle_a|1\rangle_b\right)\\[2mm]\xrightarrow{CNot}&\frac{1}{\sqrt{2}}\left(|0\rangle_a|0\rangle_b+|1\rangle_a|0\rangle_b\right)\\[2mm]=&\frac{1}{\sqrt{2}}\left(|0\rangle_a+|1\rangle_a\right)|0\rangle_b\\[2mm]\xrightarrow{H}&|0\rangle_a|0\rangle_b\end{array}")
There are four maximally entangled states for pairs of two-state systems. Doing the same operations to each yields:
![\begin{array}{rcl}\frac{1}{\sqrt{2}}\left(|0\rangle_a|0\rangle_b+|1\rangle_a|1\rangle_b\right)&\to&|0\rangle_a|0\rangle_b\\[2mm]\frac{1}{\sqrt{2}}\left(|0\rangle_a|0\rangle_b-|1\rangle_a|1\rangle_b\right)&\to&|1\rangle_a|0\rangle_b\\[2mm]\frac{1}{\sqrt{2}}\left(|0\rangle_a|1\rangle_b+|1\rangle_a|0\rangle_b\right)&\to&|0\rangle_a|1\rangle_b\\[2mm]\frac{1}{\sqrt{2}}\left(|0\rangle_a|1\rangle_b-|1\rangle_a|0\rangle_b\right)&\to&|1\rangle_a|1\rangle_b\end{array}](https://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Brcl%7D%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C0%5Crangle_a%7C0%5Crangle_b%2B%7C1%5Crangle_a%7C1%5Crangle_b%5Cright%29%26%5Cto%26%7C0%5Crangle_a%7C0%5Crangle_b%5C%5C%5B2mm%5D%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C0%5Crangle_a%7C0%5Crangle_b-%7C1%5Crangle_a%7C1%5Crangle_b%5Cright%29%26%5Cto%26%7C1%5Crangle_a%7C0%5Crangle_b%5C%5C%5B2mm%5D%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C0%5Crangle_a%7C1%5Crangle_b%2B%7C1%5Crangle_a%7C0%5Crangle_b%5Cright%29%26%5Cto%26%7C0%5Crangle_a%7C1%5Crangle_b%5C%5C%5B2mm%5D%5Cfrac%7B1%7D%7B%5Csqrt%7B2%7D%7D%5Cleft%28%7C0%5Crangle_a%7C1%5Crangle_b-%7C1%5Crangle_a%7C0%5Crangle_b%5Cright%29%26%5Cto%26%7C1%5Crangle_a%7C1%5Crangle_b%5Cend%7Barray%7D&bg=ffffff&fg=000000&s=0 "\begin{array}{rcl}\frac{1}{\sqrt{2}}\left(|0\rangle_a|0\rangle_b+|1\rangle_a|1\rangle_b\right)&\to&|0\rangle_a|0\rangle_b\\[2mm]\frac{1}{\sqrt{2}}\left(|0\rangle_a|0\rangle_b-|1\rangle_a|1\rangle_b\right)&\to&|1\rangle_a|0\rangle_b\\[2mm]\frac{1}{\sqrt{2}}\left(|0\rangle_a|1\rangle_b+|1\rangle_a|0\rangle_b\right)&\to&|0\rangle_a|1\rangle_b\\[2mm]\frac{1}{\sqrt{2}}\left(|0\rangle_a|1\rangle_b-|1\rangle_a|0\rangle_b\right)&\to&|1\rangle_a|1\rangle_b\end{array}")
These are different, definite states! So this is how to look at entangled two-state systems in the “correct” basis. But it wouldn’t be possible to do this without the CNot operation and that requires both particles to work.
The coin box pictures are from here.
Can someone explain why a dark room full of loose rope would cause problems?
@zapp
Can’t walk around in there without getting tangled up in rope.
I see, Macro entanglement. I think I could manage it without getting tangled though.
Dear Physicist, you say:
“A given quantum state can be a single state from one perspective and a superposition of several states from another.”
This is an interesting point – can you give a reference that can back that up? Thank you.
That is, for the idea that from one viewpoint there can have been an (apparent) collapse of the wave function, while from another, there has been no (apparent) collapse? This might not fit too well with objective collapse models.
@David
The important idea for this post was that the difference between a single state and a superposition is a matter of how the state is measured (the basis) exactly as much as it is a matter of the state itself.
That said, you’re right: collapse is also not objective. It’s easy to convince yourself that it is; after all, if you and someone else do the same measurement on the same thing, you’ll agree on the results. But that’s a perspective born from being part of the quantum system in question. If you manage to isolate a couple of systems and let them interact, you find that they don’t collapse, they entangle. If you ask “what does that entanglement look like from the inside?”, the answer is “it looks like collapse”. This post talks about that in more detail than you probably want.
I take your point that what one measures affects what one finds. But you say there’s also a subjective aspect of collapse. I just wondered if there’s any experimental evidence for that, or (more likely) reason to be sure this is so from within QM. I thought that a question like that was a matter of interpretation.
@David
Unfortunately, it is a matter of interpretation. We’d need to get a person to exhibit superposition (and make it cheap enough for anyone to try it) before we could really put this question to rest. I’m a big fan of “relational quantum physics“. When you compare the various interpretations you find that some of them involve a lot of new, absurd (but not necessarily baseless) ideas while others involve no new ideas, but force you to believe the universe is strange.
It’s a little like comparing the heliocentric theory vs. the geocentric theory before actually going into space. If you assume the Earth is standing still, you’re forced to make up lots of weird new laws to explain why the stars and especially the planets move in the weird ways they do. On the other hand, you could think of the Earth as just another planet and apply the laws we’ve got. One has lots of bizarre, unexplained laws and the other forces you to reconsider your (moving and spinning) place in the universe.
If you consider yourself and the world you perceive around you to be just another podunk quantum state, you find that the laws we have work, but they involve the world around us being just as weird as the quantum effects we see in the lab.
Hi, big fan here: there’s a couple of typos in the article:
1. Ctrl+f: “Unfortunately (and the this is the answer),”
2. Ctrl+f: “”Notice that the converse is also true…”, the formula that follows that has an uppercase A instead of a lowercase a.
Just proofreading, hope it helps! And again, I love your work promoting physics knowledge <3
@José Abarzúa R.
That’s fantastic! Thank you very much!
Fixed.
How many quantum leaps can an electron make in an hydrogen atom before leaving the atom?
Dear Physicist,
this is my first post here and first of all thank you very much for your great science blog!
I am not physicist, but I have one question according to the entanglement. Maybe you could help me?
“Still electron” does not react with a magnetic field. Spin causes that the electron becomes sensitive to the magnetic field. If it is up, the electron moves in one direction, if it is down then the electron moves in oposite direction. But if the electron is entangled, then it has no well defined spin and it does not react with the magnetic field.
If this is true then a question arises:
Suppose Alice and Bob have two entangled electrons. Alice places her electron in a center of a box with magnetic field and waits for Bob measurement and the collapse. After that, the Alice’s electron gets a spin and starts moving, say left or right.
Is it correct? If not, what is wrong in this thought experiment?
@Marco Bras
Thanks!
What you’re describing is a measurement; by putting an electron in a magnetic gradient you can determine its spin based on which direction it moves. So Alice is measuring the spin of her electron, just like Bob (who’s doing something similar), and the results of their measurements will be perfectly correlated as long as they’re measuring in the same direction. Although they won’t know that until they talk to each other and compare notes.
You’re right, entangled particles don’t have a particular spin, they’re in a superposition of spins. But like any superposition of multiple states, when you do a measurement the result you see is one of those states (“picked” randomly).
If you can only tell that two particles are entangled when you can measure them both together, or when persons who measured them separately can compare notes, how do we know they stay entangled when they move large distances from each other? How far away has this entanglement phenomenon been measured? Also, if Alice and Bob are in two different inertial frames, they might not be able to determine if their measurements of their particles were done at the same time (because of relativistic effects) – so even if they were able to compare notes, might they still be unable to decide if their particles were entangled or not? Finally, if Alice and Bob slowly move away from each other, making sure to keep their polarizing filters aligned with one another, does this mean they can maintain the same measurement basis, and that they can then know when the other has made a measurement?
Dear Physicist,
Regarding the analogy “For the most part, entangled particles are like a pair of carefully boxed mail-order coins with the same (random) side up.” (great analogy by the way) My question is, how are entangled particles fundamentally different than the coins? I mean the coins aren’t in a superposition of head|tail that collapses to either state when we open one of the boxes. They already have a state, we just won’t know which until we open a box.
In other words, are there experiments that show and explain how quantum entanglement is somehow different and not – similar to our coins – a pre-packaged matching of two quantum states that although random to start with got fixed in a random state as soon as the entanglement happened?
@Enzo
Absolutely! Entanglement is definitely very different from correlation (which is what the coins are working with), it’s just that correlation goes a long way toward building up good intuition.
The experiments that demonstrate the difference between regular coins and “quantum coins” (like the spin of an electron) are called “Bell tests”. They show that, unless the universe is conspiring on a truly… infeasible scale, the behavior of entangled particles cannot be explained by things being in a single (albeit complex) state.
If you’re doing the same measurement on both entangled particles, there’s no statistical difference between coins and entangled particles. Bell tests involve cute comparisons of different measurements on the two particles. The posts here and here talk about this stuff a bit more.
Hai, I’m not quite familiar with this idea but i have some questions regarding how this phenomenon become impossible for communication. If Alice and Bob have particles that are entagled, my doubt is if Alice changes any property of her particle,will Bob too observe this change in his particle? So if Alice notifies Bob that her particle is in say, +1 state, then can Bob look at his particle and see that his particle is in -1 state? If so, could we send lot of uncorrepted data from Alice to Bob instantly by first Alice collapsing her particle states, then sending just a notification by traditional data transfer and then Bob checks his particle state to calculate the original message?
@Diligent orater lee
If Alice measure her particle and gets a position, Bob won’t know unless he too measures his particle but he won’t be able to tell if she actually measured hers, until they meet to compare notes.
The act of measuring the particle destroys the entanglement, but you personally won’t know the entanglement is destroyed unless you talk to the other person who measured their particle (sub light speed communication) or measure your own particle(and definitely destroy the engagement). Hence your particles state can no longer be modified by the other person (and you wouldn’t even though if they did initially)
Hi,
Really helpful article – sorry if this is a nonsensical question but you mention that “
“There actually is a “correct” basis in which you always get definite results from entangled particles, but you need all of the involved particles in one place to do such a measurement.”
I would be really interested to understand that assuming the particles were physically co-located could you measure entangled particles with two different/physically independent instruments and receive the same result (e.g. spin up or down) even if that results of this measurement was entirely random?
This is by far the clearest explanation of quantum entanglement I’ve ever read. Thank you for this. I feel like I, at the very least, have a tiny grasp of this subject.
Can’t, won’t, impossible…bah…I say there is something we have yet to discover that will eventually allow FTL or FTL communications. Not by breaking laws of physics…but by discovering new ones or going “around” the limitations. Never say never. Just a few hundred years ago, most scientists thought human flight was impossible, not to mention space travel. Now it’s very ordinary.