Physicist: The Uncertainty Principle is a little subtle. Most folk are introduced to it as “the more precisely you measure the position of a particle, the less precisely you can measure its momentum, and vice versa”. That makes it sound like an engineering problem; something we can get around by, for example, trying harder.
Normally, if there’s a limit to how well you can measure something, you just need better equipment. But “there’s a limit to how well we can measure things” is not what the Uncertainty Principle is about.
But the Uncertainty Principle is a statement about what things are actually like in reality, and the weird limitations on how well we can do measurements is just a symptom. In a very fundamental, profound, and physical sense, everything is a little uncertain.
To be clear, you can measure the position and momentum of a single particle (or many particles or whatever quantum system you prefer) very precisely, and there’s nothing stopping you from getting very precise results from both measurements. The problem is that those precise measurements don’t really tell you much about the actual quantum state you’re looking at. You can prepare a series of identical quantum states and measure each of them in exactly the same way, but because quantum states are generally a combination of many different states together (what folk in the quantum biz call a “superposition of states”) you generally don’t get the same result over and over.
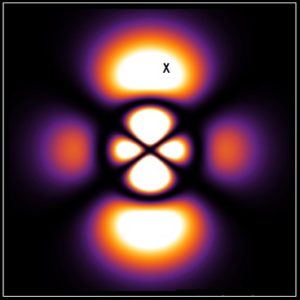
This is a map of where an electron in a particular quantum state (in the 4,2,0 orbital) is most likely to be found when measured. But the electron’s state is this whole thing. If you do a measurement and find the electron at the “x”, you’re still kinda missing the big picture.
An electron in an atomic orbital is in a state with one amount of energy, but many positions. The electron is kinda “smeared out” around the atom. So if you measure the energy you get a definite result, but if you measure the position you could get any result within a range of positions (a small range, what with atoms being small). You can picture this as being like a musical chord and either asking “what chord was played?” or “what note was played?”. For example, the C chord is composed of the C, E, and G notes. If you do a chord measurement (this is not an actual thing, but bear with me), you get a definite answer: C. If you do a note measurement (again, more quantum metaphor than mechanics), then you get one of three results: C, E, or G.
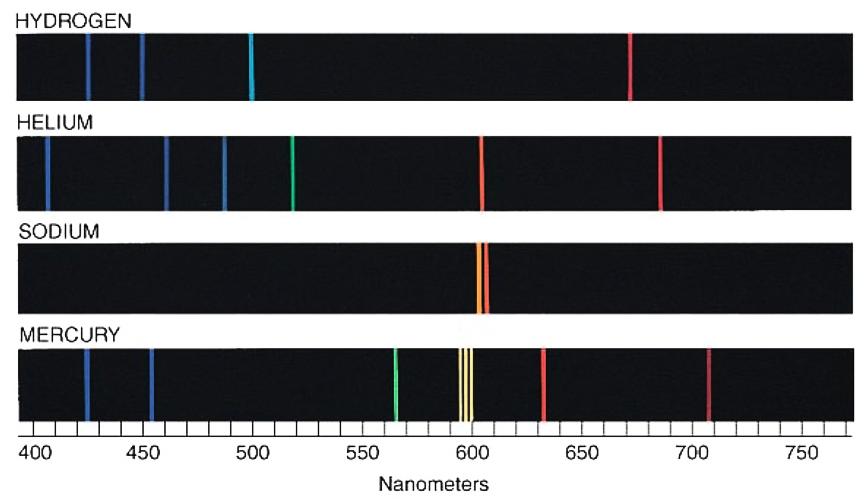
Each electron orbital is a very specific energy state (hence the discrete spectral lines produced when electrons jump between them), but at the same time each is a superposition of many positions, so the electrons don’t exist in any particular place.
The randomness of the Uncertainty Principle has the same root cause: a single quantum state being composed of many different states at the same time. Like the chord example, a position state is made up of a range of many momentum states. Unlike the chord example, the reverse is also true; a momentum state is composed of many position states. Unintuitively, the fewer position states something is in (the more specific the position) the more momentum states it is also in. Unfortunately, because of this fact (that position is describable in terms of momentum and vice versa) you can directly derive the uncertainty principle mathematically. In other words, assuming that every experiment ever designed to refute the basic physics didn’t all fail accidentally, the Uncertainty Principle is built into the universe and no cleverness or engineering will overcome it.
When you prepare many particles (or any other quantum system) in identical quantum states, measure them one after another, and write down the results, you’ll find that there’s some spread in where they show up as well as their momenta. You can prepare states with very little spread in their position or with very little spread in their momenta (so called “squeeze states”), so the Uncertainty Principle isn’t as simple as “everything is random and unpredictable”, it’s about pairs of measurements applied to “conjugate variables” (position and momentum are the classic example).
The Uncertainty Principle says that if you look at the spread (standard deviation) of those two measurements for many copies of any given state and multiply those spreads together, their product is always greater than some minimum amount. Explicitly, if 
Entanglement doesn’t do much to change the picture. With entangled pairs of particles, a measurement on one mirrors a measurement on the other. You can entangle any property (energy, polarization, delectability, hell even existence), including position and momentum. In a dangerously succinct nutshell, entanglement basically/sorta gives you two chances to make a measurement on a quantum state. Assume particles A and B are position-entangled. If you measure the position of A you’ll be able to say “ah, it’s over here” and if you measure the position of B you’ll be able to say “it sure is”. The two measurements, although otherwise random, agree.
But what you’d really like to see is a precise position measurement on A and a precise momentum measurement on B. It turns out: that’s fine. Once again, that spread of results shows up. If A shows up in some region, there is a corresponding set of momentum states that are compatible with that (if a certain “chord is played” there is a particular “set of notes” involved) and when you measure B, you’ll see one of those.
So entanglement does give you another chance to measure a quantum state with as much precision as you might desire, but… it doesn’t really change anything. The Uncertainty Principle doesn’t say “you can’t simultaneously measure position and momentum with nigh perfect precision!”, it says “it doesn’t matter if you do!”.
The giant microscope picture is from here.
I just wanted to say how much I enjoy all these quantum mechanics posts! Quantum mechanics is so hard to understand and so confusing and I find these are targeted at just the right level to be comprehendible, as well as challenging some of the ideas or conceptions that I have held before (case in point, I don’t think I quite understood the uncertainty principle :D).
@Arwen Nugteren
I’m flattered! Glad you’re enjoying the site!
> With entangled pairs of particles, a measurement on one mirrors a measurement on the other. You can entangle any property (energy, polarization, delectability, hell even existence)
Could you elaborate on what it means for detectability or existence to be entangled?
@Aaron1011
Delectibility was a weak joke (being delectable is probably a classical quantity).
There are a few ways to entangle existence, but an easy way is to get a process that produces pairs of particles through some fundamentally random process. The pair will both already be produced and not yet produced. Franson’s “A Bell Inequality in Position and Time” is a beautiful example where the entanglement is made explicit.
Always enjoy the articles.
Sean Corroll in his video https://youtu.be/iXRLDatmbgA says (paraphrasing).. ‘it’s not that we don’t know how to measure position and moment.., there is no such thing as position and moment‘.
Comment?
@Benjamin Hamilton
Glad you’re enjoying them!
It’s not quite fair to say that position and momentum don’t exist. I mean… clearly things exist in places. It’s just that definite, absolutely precise position isn’t a thing. But in practice, you’d never notice. The uncertainty principle is not why we lose our keys.
Why make it so complex. It is really very simple. You cannot know with absolute precision the position and the momentum of a particle, or indeed a bus, because to know the momentum you have to know the position twice and these positions must be different and, incidentally, the momentum calculated is the average momentum the particle has between whatever two positions you select.