Physicist: Like any plumbing fix: it depends on the pipe.
Evangelista Torricelli, the first to measure the weight of the air above us, once famously wrote that “Noi viviamo sommersi nel fondo d’un pelago d’aria.” (“We live submerged at the bottom of an ocean of air.”). That’s a really good way to think about the atmosphere. It’s held in place around the Earth in exactly the same way that the oceans are held where they are; air has weight and gravity holds it down. Gravity doesn’t suddenly go away above the atmosphere. In low Earth orbit (where most satellites can be found) gravity is almost as strong as it is on the surface. So if you pump air or CO2 or any kind of matter above the Kármán Line (the generally agreed upon, but arbitrary, boundary of space), it will still be subject to gravity and will fall. You’ll have yourself a CO2 fountain.
Pumping stuff to just above the atmosphere isn’t an effective way to get rid of it, although it might be pretty if it’s visible from the ground.
If you build your pipe straight up from one of the poles, then it’ll be fountain-like even when it’s millions of miles tall. More importantly, there are no materials that can support such a pipe (it’s important, when building a CO2 pump to the stars, to be realistic). However, a pipe built up from the equator would swing as the Earth turns, and that changes things a lot. Shorter pipes (a mere several thousand km tall) will still act like fountains, but eventually that swinging motion becomes important.
Space elevators operate on exactly this idea; combating gravity using the Earth’s rotation. So figuring out what happens to the CO2 flying out of the top of a very tall pipe boils down to figuring out what would happen if you stepped out of a space elevator at the same height. And since it is entirely possible (but difficult) to build space elevators, this plan could work. In fact, since that elevator would need to be made from carbon nanotubes (no other material is known to be strong enough), we’d be using carbon to get rid of carbon. That’s almost like being efficient!
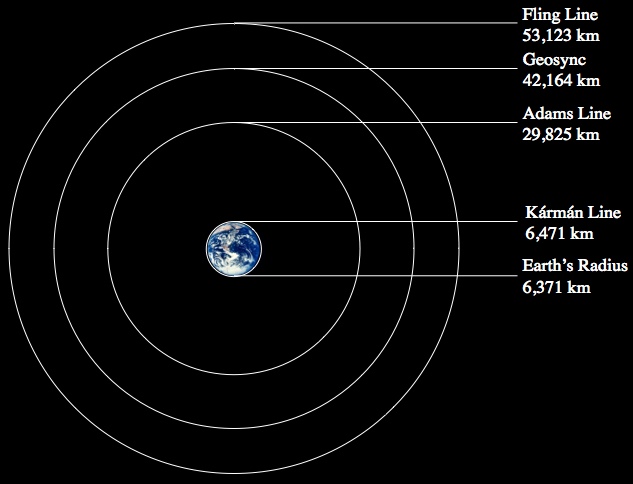
Douglas Adams once sagely observed that “There is an art to flying, or rather a knack. The knack lies in learning how to throw yourself at the ground and miss. … Clearly, it is this second part, the missing, that presents the difficulties.” Ordinarily this is difficult, but if the tower you throw yourself off is at least around 30,000 km from the center of the Earth, then by the time you get to the ground it won’t be there. Sweeping out a larger circle every day than a point on the ground means the platform you jump from will be moving sideways faster. Once it’s fast enough, when you throw yourself at the ground you’ll literally miss and find yourself in an orbit that skims just above the surface of the Earth at perigee (at the lowest) and returns up to the height of the platform at apogee (at the highest). Lacking an official name, I do hereby declare this to be the “Adams Line”.
What happens to the CO2 when you pump it into space depends on how high it is when it’s released.
Below the Adams Line, all of the CO2 pumped out of the pipe will fall back to Earth. Above the Adams line the released CO2 will find itself in orbit, and we’ll have given Earth a ring system. This wouldn’t be a permanent solution. All of the material in a ring system needs to “stay in its own lane” and orbit in a near-perfect circle (hence: “ring”) or things will run into each other, lose energy, drop into lower orbits and eventually return to Earth. A whole series of overlapping elliptical orbits swerving from the height of the pipe to as low as Earth’s surface would be a very messy, very unstable ring system.
Gases released above the Adams Line will orbit the Earth instead of falling back to the ground. However, gases released at different times will be on crossing (colliding) orbits, that would make Earth’s new rings very unstable.
But the higher the pipe, the more circular the orbit. Once the top of the pipe reaches geosync, it’s in orbit. If you were to step off of a platform you wouldn’t fall, you’d just drift. Gas released at this height wouldn’t swerve between altitudes as it orbited Earth, it would more or less stay where it is and instead would slowly fill up the thin ring that forms geosync. The most expensive and sought-after orbits around Earth would get dirtied up and be far less useful for satellites, but it’s a small price to pay.
We actually have examples of “pipes” releasing gas into their own orbits, so we know more or less what it would look like. Several of the moons in our solar system are geologically active and, having much lower gravity, eruptions from their surfaces don’t necessarily fall back to the ground.
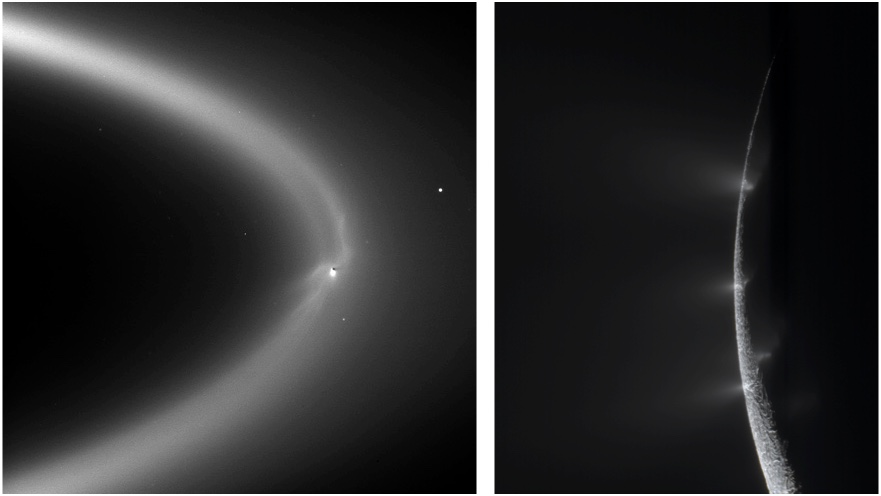
Enceladus’ ice geysers release water into (roughly) the same orbit around Saturn, forming one of Saturn’s many rings.
So between the Adams Line and geosynchronous orbit we’d have a temporary ring system, perhaps giving us time to think up an even crazier plan, and above geosync we’d have a fairly stable ring system (no ring systems are entirely stable). But if we want to get rid of Earth’s CO2 forever, and not just orbitally sequester it, we need it to escape from Earth’s gravity entirely.
At geosync objects orbit Earth once per day. Below that they orbit faster and above that they orbit slower (for example, the Moon, which is way beyond geosync, takes a month to orbit). So if the pipe is taller than about 42,000 km, the CO2 it releases will actually fly upward before falling into orbit, and the taller the pipe, the higher that orbit will be. Beyond about 53,000 km, the top of the pipe will be moving at escape velocity; the CO2 that comes out will fly away from Earth to find its way into orbit around the Sun.
Interesting fun-fact: as measured from the center of any planet, the height of the “Fling Line” is always the cube root of 2 (≈1.26) times the height of geosynchronous orbits. The Fling Line is a really good goal for anyone planning to build a space elevator, because it’s a free ticket to everywhere.
Swing hard enough and eventually centripetal force wins. Above the “Fling Line” (not an official name) stuff that’s released never comes back down.
You might worry about some small amount returning to Earth, but you should be equally worried about someone in Antarctica having bad breath; there’s a lot of room for that problem to diffuse.
So that there is the answer. If you want to solve global warming, you can just gather up and pump CO2 straight up about 53,000 km. Easy peasy.
The weakness of brilliant plans like this isn’t feasibility (this could technically be done), it’s the naysayers who point out that the cure might be worse than the disease. After all, carbon is an essential part of the Earth’s biological and chemical systems. We’ve added about half-again as much CO2 into the atmosphere in the last few centuries, taking us from below 300 ppm to about 400 ppm today. There’s been 400 ppm of CO2 in the Earth’s atmosphere before now, most recently in the Micene 10 million years ago. Way back then life on Earth was doing alright (we got grasslands and kelp forests out of it, which isn’t terrible). Having a bunch of CO2 around isn’t necessarily bad; like highway driving, the danger doesn’t come from any particular situation so much as sudden changes in situation. Given that, suddenly and irreversibly chucking a third of Earth’s CO2 into space has a fair chance of being a really bad idea.
Answer Gravy: If you’re wondering where the various heights came from, you’ll be thrilled to know that you can figure out the height of both the Adams Line and Fling Line (not the official names), with nothing fancier than algebra.
A Keplerian orbit (a regular elliptical or hyperbolic orbit) can be described as:
where r is the distance from the center of the Earth (or whatever you happen to be orbiting), e is the eccentricity (e=0 for a circle, 0<e<1 for an ellipse), 
If 
![\begin{array}{ll}r(\theta) = \frac{(hV_t)^2}{Gm[1+e\cos(\theta)]} \\[2mm]e\cos(\phi) = \frac{hV_t^2}{Gm}-1\\[2mm]e\sin(\phi) = \frac{hV_rV_t}{Gm}\end{array}](https://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Bll%7Dr%28%5Ctheta%29+%3D+%5Cfrac%7B%28hV_t%29%5E2%7D%7BGm%5B1%2Be%5Ccos%28%5Ctheta%29%5D%7D+%5C%5C%5B2mm%5De%5Ccos%28%5Cphi%29+%3D+%5Cfrac%7BhV_t%5E2%7D%7BGm%7D-1%5C%5C%5B2mm%5De%5Csin%28%5Cphi%29+%3D+%5Cfrac%7BhV_rV_t%7D%7BGm%7D%5Cend%7Barray%7D&bg=ffffff&fg=000000&s=0 "\begin{array}{ll}r(\theta) = \frac{(hV_t)^2}{Gm[1+e\cos(\theta)]} \\[2mm]e\cos(\phi) = \frac{hV_t^2}{Gm}-1\\[2mm]e\sin(\phi) = \frac{hV_rV_t}{Gm}\end{array}")
Normally you don’t know what the initial angular position is, you just know how high you are and how you’re moving.
This is a slightly streamlined version of the solution that can be found here. Jumping off of a platform that’s h above the center of the Earth and attached to said Earth means that 
Use the second equation to solve for e:
![\begin{array}{rcl}e\cos(\phi) &=& \frac{rV_t^2}{Gm} - 1 \\[2mm]-e\cos(180^o) &=& \frac{h(\frac{2\pi h}{t})^2}{Gm} - 1 \\[2mm]-e &=& \frac{h(\frac{2\pi h}{t})^2}{Gm} - 1 \\[2mm]e &=& 1 - \frac{h(\frac{2\pi h}{t})^2}{Gm} \\[2mm]e &=& 1 - \frac{4\pi^2 h^3}{Gmt^2} \\[2mm]e &=& \frac{Gmt^2 - 4\pi^2 h^3}{Gmt^2}\end{array}](https://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Brcl%7De%5Ccos%28%5Cphi%29+%26%3D%26+%5Cfrac%7BrV_t%5E2%7D%7BGm%7D+-+1+%5C%5C%5B2mm%5D-e%5Ccos%28180%5Eo%29+%26%3D%26+%5Cfrac%7Bh%28%5Cfrac%7B2%5Cpi+h%7D%7Bt%7D%29%5E2%7D%7BGm%7D+-+1+%5C%5C%5B2mm%5D-e+%26%3D%26+%5Cfrac%7Bh%28%5Cfrac%7B2%5Cpi+h%7D%7Bt%7D%29%5E2%7D%7BGm%7D+-+1+%5C%5C%5B2mm%5De+%26%3D%26+1+-+%5Cfrac%7Bh%28%5Cfrac%7B2%5Cpi+h%7D%7Bt%7D%29%5E2%7D%7BGm%7D+%5C%5C%5B2mm%5De+%26%3D%26+1+-+%5Cfrac%7B4%5Cpi%5E2+h%5E3%7D%7BGmt%5E2%7D+%5C%5C%5B2mm%5De+%26%3D%26+%5Cfrac%7BGmt%5E2+-+4%5Cpi%5E2+h%5E3%7D%7BGmt%5E2%7D%5Cend%7Barray%7D&bg=ffffff&fg=000000&s=0 "\begin{array}{rcl}e\cos(\phi) &=& \frac{rV_t^2}{Gm} - 1 \\[2mm]-e\cos(180^o) &=& \frac{h(\frac{2\pi h}{t})^2}{Gm} - 1 \\[2mm]-e &=& \frac{h(\frac{2\pi h}{t})^2}{Gm} - 1 \\[2mm]e &=& 1 - \frac{h(\frac{2\pi h}{t})^2}{Gm} \\[2mm]e &=& 1 - \frac{4\pi^2 h^3}{Gmt^2} \\[2mm]e &=& \frac{Gmt^2 - 4\pi^2 h^3}{Gmt^2}\end{array}")
and then plugging that into the first equation gives us the orbit:
![r(\theta) = \frac{(rV_t)^2}{Gm[1+e\cos(\theta)]} = \frac{4\pi^2 h^4}{Gmt^2[1+e\cos(\theta)]}=\frac{4\pi^2 h^4}{Gmt^2[1+\frac{Gmt^2 - 4\pi^2 h^3}{Gmt^2}\cos(\theta)]}=\frac{4\pi^2 h^4}{Gmt^2+(Gmt^2 - 4\pi^2 h^3)\cos(\theta)}](https://s0.wp.com/latex.php?latex=r%28%5Ctheta%29+%3D+%5Cfrac%7B%28rV_t%29%5E2%7D%7BGm%5B1%2Be%5Ccos%28%5Ctheta%29%5D%7D+%3D+%5Cfrac%7B4%5Cpi%5E2+h%5E4%7D%7BGmt%5E2%5B1%2Be%5Ccos%28%5Ctheta%29%5D%7D%3D%5Cfrac%7B4%5Cpi%5E2+h%5E4%7D%7BGmt%5E2%5B1%2B%5Cfrac%7BGmt%5E2+-+4%5Cpi%5E2+h%5E3%7D%7BGmt%5E2%7D%5Ccos%28%5Ctheta%29%5D%7D%3D%5Cfrac%7B4%5Cpi%5E2+h%5E4%7D%7BGmt%5E2%2B%28Gmt%5E2+-+4%5Cpi%5E2+h%5E3%29%5Ccos%28%5Ctheta%29%7D&bg=ffffff&fg=000000&s=0 "r(\theta) = \frac{(rV_t)^2}{Gm[1+e\cos(\theta)]} = \frac{4\pi^2 h^4}{Gmt^2[1+e\cos(\theta)]}=\frac{4\pi^2 h^4}{Gmt^2[1+\frac{Gmt^2 - 4\pi^2 h^3}{Gmt^2}\cos(\theta)]}=\frac{4\pi^2 h^4}{Gmt^2+(Gmt^2 - 4\pi^2 h^3)\cos(\theta)}")
If you plug in 
It’s good to double check. But to actually figure out what h is (this was the whole point) you want the lowest point in the orbit to be the Earth’s radius, R=6371 km (any lower and you hit the ground).
![\begin{array}{rcl}R &=& \frac{4\pi^2 h^4}{Gmt^2+(Gmt^2 - 4\pi^2 h^3)\cos(0^o)} \\[2mm]R &=& \frac{4\pi^2 h^4}{2Gmt^2 - 4\pi^2 h^3} \\[2mm]R(2Gmt^2 - 4\pi^2 h^3) &=& 4\pi^2 h^4 \\[2mm]0 &=& 4\pi^2 h^4 + 4\pi^2 Rh^3 - 2Gmt^2R\end{array}](https://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Brcl%7DR+%26%3D%26+%5Cfrac%7B4%5Cpi%5E2+h%5E4%7D%7BGmt%5E2%2B%28Gmt%5E2+-+4%5Cpi%5E2+h%5E3%29%5Ccos%280%5Eo%29%7D+%5C%5C%5B2mm%5DR+%26%3D%26+%5Cfrac%7B4%5Cpi%5E2+h%5E4%7D%7B2Gmt%5E2+-+4%5Cpi%5E2+h%5E3%7D+%5C%5C%5B2mm%5DR%282Gmt%5E2+-+4%5Cpi%5E2+h%5E3%29+%26%3D%26+4%5Cpi%5E2+h%5E4+%5C%5C%5B2mm%5D0+%26%3D%26+4%5Cpi%5E2+h%5E4+%2B+4%5Cpi%5E2+Rh%5E3+-+2Gmt%5E2R%5Cend%7Barray%7D&bg=ffffff&fg=000000&s=0 "\begin{array}{rcl}R &=& \frac{4\pi^2 h^4}{Gmt^2+(Gmt^2 - 4\pi^2 h^3)\cos(0^o)} \\[2mm]R &=& \frac{4\pi^2 h^4}{2Gmt^2 - 4\pi^2 h^3} \\[2mm]R(2Gmt^2 - 4\pi^2 h^3) &=& 4\pi^2 h^4 \\[2mm]0 &=& 4\pi^2 h^4 + 4\pi^2 Rh^3 - 2Gmt^2R\end{array}")
This is a fourth degree polynomial in h, and while it is technically possible to solve this by hand, you never want to solve this by hand. So plugging in the gravitational constant, 
The height of the Fling Line (not an official name), f, is a bit easier. You don’t have to mess around with orbits, you just have to set the speed your platform is moving at a given height, 
![\begin{array}{rcl}\frac{2\pi f}{t}&=&\sqrt{\frac{2Gm}{f}}\\[2mm]\frac{4\pi^2f^2}{t^2}&=&\frac{2Gm}{f}\\[2mm]h^3&=&\frac{2Gmt^2}{4\pi^2}\\[2mm]f&=&\sqrt[3]{\frac{2Gmt^2}{4\pi^2}}\end{array}](https://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Brcl%7D%5Cfrac%7B2%5Cpi+f%7D%7Bt%7D%26%3D%26%5Csqrt%7B%5Cfrac%7B2Gm%7D%7Bf%7D%7D%5C%5C%5B2mm%5D%5Cfrac%7B4%5Cpi%5E2f%5E2%7D%7Bt%5E2%7D%26%3D%26%5Cfrac%7B2Gm%7D%7Bf%7D%5C%5C%5B2mm%5Dh%5E3%26%3D%26%5Cfrac%7B2Gmt%5E2%7D%7B4%5Cpi%5E2%7D%5C%5C%5B2mm%5Df%26%3D%26%5Csqrt%5B3%5D%7B%5Cfrac%7B2Gmt%5E2%7D%7B4%5Cpi%5E2%7D%7D%5Cend%7Barray%7D&bg=ffffff&fg=000000&s=0 "\begin{array}{rcl}\frac{2\pi f}{t}&=&\sqrt{\frac{2Gm}{f}}\\[2mm]\frac{4\pi^2f^2}{t^2}&=&\frac{2Gm}{f}\\[2mm]h^3&=&\frac{2Gmt^2}{4\pi^2}\\[2mm]f&=&\sqrt[3]{\frac{2Gmt^2}{4\pi^2}}\end{array}")
You can calculate the height of geosync, s, by setting the centrifugal force equal to the gravitational force for an object orbiting exactly once per (sidereal) day, t:
![\begin{array}{rcl} \frac{\left(\frac{2\pi s}{t}\right)^2}{s}&=&\frac{Gm}{s^2}\\[2mm] \frac{4\pi^2s^2}{ht^2}&=&\frac{Gm}{s^2}\\[2mm] s^3&=&\frac{Gmt^2}{4\pi^2}\\[2mm] s&=&\sqrt[3]{\frac{Gmt^2}{4\pi^2}} \end{array}](https://s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Brcl%7D+%5Cfrac%7B%5Cleft%28%5Cfrac%7B2%5Cpi+s%7D%7Bt%7D%5Cright%29%5E2%7D%7Bs%7D%26%3D%26%5Cfrac%7BGm%7D%7Bs%5E2%7D%5C%5C%5B2mm%5D+%5Cfrac%7B4%5Cpi%5E2s%5E2%7D%7Bht%5E2%7D%26%3D%26%5Cfrac%7BGm%7D%7Bs%5E2%7D%5C%5C%5B2mm%5D+s%5E3%26%3D%26%5Cfrac%7BGmt%5E2%7D%7B4%5Cpi%5E2%7D%5C%5C%5B2mm%5D+s%26%3D%26%5Csqrt%5B3%5D%7B%5Cfrac%7BGmt%5E2%7D%7B4%5Cpi%5E2%7D%7D+%5Cend%7Barray%7D&bg=ffffff&fg=000000&s=0 "\begin{array}{rcl} \frac{\left(\frac{2\pi s}{t}\right)^2}{s}&=&\frac{Gm}{s^2}\\[2mm] \frac{4\pi^2s^2}{ht^2}&=&\frac{Gm}{s^2}\\[2mm] s^3&=&\frac{Gmt^2}{4\pi^2}\\[2mm] s&=&\sqrt[3]{\frac{Gmt^2}{4\pi^2}} \end{array}")
So that’s a cute, probably useless fact; for any planet, the Fling Line is always at ![\sqrt[3]{2}](https://s0.wp.com/latex.php?latex=%5Csqrt%5B3%5D%7B2%7D&bg=ffffff&fg=000000&s=0 "\sqrt[3]{2}")
Interesting. Have you ever discussed the fact that AGW is not possible; at least not with just fossil fuel consumption as we are doing now and in decreasing amounts, at that?
The earth would look good with a dry ice ring don’t you think…
@Chris
It would, but rings are awfully inconvenient for satellites and space travel in general. And there are enough problems there already without adding more to the plate.
isn’t that the ‘worst’ thing we could do? wouldn’t that be like wrapping the earth in a nice, hot thermal blanket?
@Anonymous
We wouldn’t be adding new CO2, we’d be rearranging it. There’d be a thin band in the sky that reflects heat back, but most of the sky (toward the north and south) would still be clear. Clearer even, since the CO2 that would have been there is thousands of miles above the equator.
Chris
Temperature high enough for ice form
(if it allow every planet must have rings )
BR
“We wouldn’t be adding new CO2, we’d be rearranging it.”
Most of the carbon dioxide on the planet earth is dissolved in water. If we take CO2 out of the lower atmosphere and push it into orbit, is it replaced with CO2 that bubbles out of the oceans?
@frank
Yes but it will be a net lower level. In the saltwater aquarium hobby we manipulate ph by changing the atmospheric co2 that the system is exposed to. Its as simple as opening a window or feeding air via a soda line co2 scrubber. Which leads to me question…
@the physicist
Would a 54000 km tube/tower be more feasible than binding it to a solid in a scrubber and burying it?
@Jeff
Probably not, as binding CO2 into a solid and burying it just requires growing a bunch of trees, and burying them.
The tube would probably be quicker, but not more feasible.
Hi,
I had a lucid dream whereby i imagined a large solar farm sucking CO2 out of the atmosphere out into space.
After find this article I presume the farm could sun orbit approx 15k above the earths surface. A pipe lowered into the atmosphere and a pipe extending to a point where the CO2 could be discharged.
Possible?
Could space travellers like Elon Musk use the CO2 ring as a refuelling stop, once they have escaped from earth’s atmosphere? That CO2 mixed with water and oxygen would power them on their way to Mars!
@W. William Woods
I don’t see why not, although if they did it might be more convenient to keep the CO2 packed in tanks. Alternatively, if the pipe is long enough, it could actually fling them to Mars or anywhere else in the solar system. There’s another post that talks about exactly that!
I envisaged floating tanks too….but, a naive question, could we “float” the gas up there in some kind of balloons and hold it in the balloons?
have you considered how a propulsion system could be used to push the CO2 through the tube/pipe and create a stream that could propel the gas further into space? would the gas lose velocity once it leaves the pipe?
@William Woods
Balloons won’t go higher than the atmosphere and can never be denser than the air around them. Even worse, CO2 is one of the heavier gases in our atmosphere. So by the time we’re using balloons, nothing is actually the better option.
@Johnnyms
As soon as it’s free of whatever is propelling it, the gas would start to fall which (if it were busy moving up) would mean slowing down.
That was fascinating and a much better answer than I expected, thank you so
much.:)
Pump the CO2 into space, form giant dry ice cubes, fling them to Mars – Mars gets an atmosphere!
I am thinking about this theory to get rid of Co2 since i was 12 years old now i am 48 years 😀 – the biggest problem no considers here is weight , the weight of the pipe from inside the atmosphere / ground until it reaches zero gravity is extreme , the shear force is extreme we have currently no material which can withstand this , the weight of carbon dioxide it self , is under the same law as a rocket , and need a giant amount of power to overcome the earth gravity , the power the pumps must have is also not practical – it is probably easier to build Aluminium tanks and liquefy the Co2 on earth and shoot the tanks with conventional rocket into orbit and direct them into a collision course with the sun / no worries it will not dilute the sun itself as the containers will melt long before they would touch it , and now since Rockets can be reused with space X it would be easier to do , i guess 🙂 but the amount of Co2 we are able to get out of the atmosphere is very small compared to the amount avaiable
What about the pressure differential. Would you need to pump or would it flow from higher pressure atmosphere to space?
@Patrick
No it wouldnt , otherwise it would do it now , its kept down by Gravity
Could you ignite the greenhouse gases. Or parts of them. (Methane)? Then changing the relationship between them. Does CO2 burn? What would happen?
CO2 does not burn … you need much energy
To split o2 and carbon, not sure if there us an actual procedure for it, as it a very stable and unreactive chemical
You can burn methane but first it needs to be collected and burning methane results in Co2 and water… also does not really help
So why aren’t we doing this already? Sounds cheaper than space travel. Bezos, Musk, Branson WYA?
Plus a nice ring of co2 dry ice sounds 🤩
Not a scientist but what if we pump the CO2 into the vast empty pockets in the earth that we pumped oil from and just lock them underground? Would it leak up slow enough for us to get a handle on things? Buy us some time?
Rather than pump the CO2 up, what about dropping pipe down from orbiting space stations that could focus on areas that had the biggest concentrations and sucking the CO2 up? Then package it in disposable rockets and shoot them at the sun?
@Justin Talbot
This approach is a part of our net zero strategy in Denmark (and elsewhere I imagine):
https://www.oedigital.com/amp/news/497455-semco-maritime-develops-pumping-system-for-danish-co2-storage-project
It seems feasible. why not try it. have to do something fast. bring it back to 1960 level. Action,please!
It seems feasible. why not try it. have to do something fast. bring it back to 1960 level. Action,please! no, I said something like that. It bears repeating
All these weather events hurricanes, typhoons… happening due to increasing in earthtemp and it caused by emissions and other forces. Which means earth trying to cool itself and it will get worse till something happen to our system. Plus our actions till this day is not enough to fix these issue and we can see it, its getting worse. I was planning to file a patent application regarding this topic.
So we need something that works fast ,deliver results fast , stable in the same time and should be cheap for everybody.
Imo, Its not realistic to pump CO2 to the space due to gravity but its not impossible. You need a space station to hold the pipe from above and a strong pipe to overcome the forces between earth and the station.
We need a system that able to bring all these emissions to same level that earth can handle so it can heal or its a cycle and its happing anyways because there is no cheaper fuel than fossil fuels.
Our modern world is derived by profit so if these is no money coming from it , nobody will invest.
Why we are all here? because we want to do something.
Till that day,
stay safe
Omar
Could we pump it to the moon? A bit more pipe needed but it would be contained in its atmosphere?
Could we create a massive slingshot/catapult powerful enough to get it out far enough? Just tossing ideas out lol
Thanks for the explanations. I have been thinking about this for a few years. I was on a train to Stockholm and was thinking about CO2 sinks and then looked up and thought why not into space? My physics are nowhere near good enough – I am an IPCC person who has worked on food security impacts – but I wondered if Fick’s difusion equations could be useful. We use them to model photosynthesis in plants as that is a diffusive process with a resistance network.
Having a sublimated CO2 white ring around Earth would also increase the global albedo,
– even if it was a little bit dirty.
The film of J Robert Oppenheimer and the process by which a cohort of the best mainly atomic physicists came together is a model, at exactly the right time, for saving planet Earth (as they said). Put simply, we need an equivalent initiative to the Manhattan Project, comprising the world’s best atmospheric physicists and engineers to design, build and implement machines that will allow the world to avoid cataclysmic global heating, cause primarily by the burning of fossil fuels. We need to allow big and even ‘wacky’ ideas to be examined for this purpose. The same spirit and intent that was engendered by the scientific community in the USA in World War Two and, more recently, at a global level during the Covid pandemic needs to be reapplied to deal with global heating, the dystopian consequences of which are become daily clearer.
Great discussion.
I wonder how the CO2 gas would be isolated to send up just that through the Fling pipe(s)?
If it can be isolated, the next questions are:
– what diameter pipe(s) would be needed?
– I’m thinking the pipes would bend due to the centrifical force of the earth’s spin, requiring more length assuming the pipe doesn’t snap.
– Would the pipe get wound up around the equator like a yo-yo’s string?
– Is there enough carbon on earth to make a pipe of the diameter and length required?
If the Fling pipe is not feasible is there a low energy or fusion/ fission method to break the CO2 gas up into useful carbon briquettes, and Oxygen?