Physicist: Electromagnetism and all the involved math are surprisingly visual sciences. Understanding Maxwell’s equations (the equations of electromagnetism) involves pictures aplenty.
In these equations and
are physical constants that dictate how strong the electric and magnetic forces are, but when doing calculations (or really whenever) it’s a pain to keep track of them. So, from now on, I’ll ignore them:
Where:
The electric and magnetic fields, “” and “
“, are like wind or flowing water; at every location they point in some direction, with some strength.
By the way, you’d expect “M” to be used for the magnetic field, but it’s already used for mass, so some genius decided “B” was a good letter for magnets. There are two strange symbols (operations) in these equations: “” and “
“. The first is called the “divergence”, and the second is called “curl”. Here’s what they mean.
Divergence () is a measure of how much a field comes together or flies apart. In what follows “
” is the flow of water or wind.
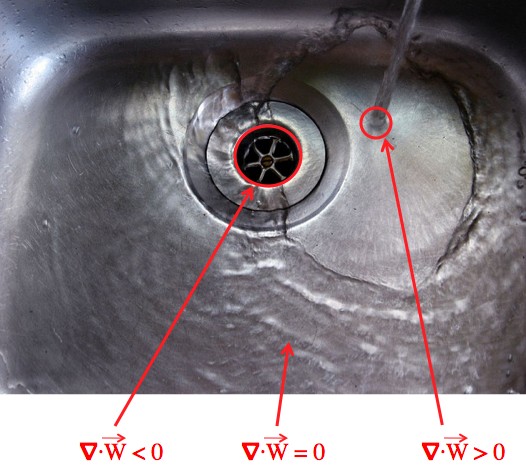
Restrict your attention only to the surface of the sink. Positive divergence means that the field is "flowing" out of a region. Negative divergence means that the field is "flowing" into a region. When the divergence is zero, then the amount that "flows" in must be equal to the amount that flows out.
Curl is how much the field “twists”. For some idea of what curl does to a field; in a whirlpool or a tornado all of the curl is in the center funnel, and the field (wind) wraps around where the curl is.

If W is the way the wind is blowing, then the tornado is the curl of W. Curl is the "twistiness" of the field, which points around the curl. Although the wind around the tornado is moving, all of the twisting is happening only at the tornado itself.
i) :
You can picture magnetic and electric fields in terms of “field lines”. The more field lines, the stronger the field. You can use this metaphor, for example, to picture why the fields lose strength as you get farther and farther away from their source (the lines spread out).
This first equation simply states that electric field lines () only start at positive charges (
), and only end at negative charges (
).
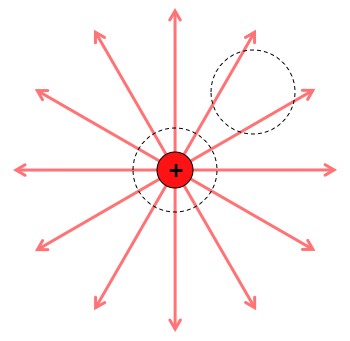
Electric field lines only begin and end at charges. Any region that contains no charges (or an equal number of positive and negative charges) will have the same amount of field lines entering it as exiting.
If a region contains no charges, then the number of field lines entering it is the same as the number of field lines exiting it. Again, “field lines” don’t exist. They’re just a really useful metaphor.
ii) :
This is the magnetic version of the electric equation above. This states that magnet field lines never begin or end. Instead, they must always form closed loops.
If somehow magnetic monopoles (“magnetic charges”) existed, then this equation would look exactly like (i). However, there are no magnetic monopoles.
iii) :
First the “J” part: This equation states that magnetic fields (B) literally curl around electrical currents (J).
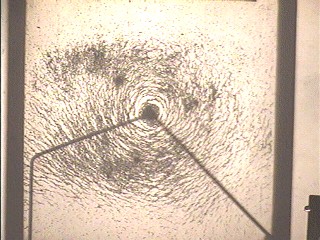
"Magnetic fields curl around current". This is a current-carrying wire going through a piece of paper with iron fillings on it. Iron fillings have the nice property that they tend to line up along magnetic fields.
So, in terms of the metaphors earlier, an electric current is like the funnel of a tornado, and the magnetic field is like the wind whipping around the funnel.
A different, but equivalent, way of stating this equation is: “if there’s a magnetic field running around a loop, then there must be a current running through the loop”.
Say you have a farm and it’s completely enclosed by a fence. Then this statement is equivalent to saying “if there’s wind blowing in the same direction all the way around the fence, then there must be a tornado in my farm”. Basically, if the wind is moving in circles, it must be twisting around at some point, and that’s the curl.
Notice that the word “through” was underlined (for emphasis!). When a scientist talks about going through a loop, it’s important to have a rigorous definition. After all, what if the loop is really weird shaped? So you find a “spanning surface”. A spanning surface is any surface that has the loop as a boundary.
The current is defined to be “going through the loop” if it passes through that loop’s spanning surface. This may seem like a useless, ad-hoc way of defining “through”, but it turns out that the math likes it. It falls out of a theorem called “Stokes theorem“. What’s very important is that the current needs to be passing through any spanning surface.
Which brings us to the second part of the equation:
What if your wire has a capacitor in it? A capacitor (at its most basic) is a wire going to a plate, then a gap, then another plate, then the wire continues (top diagram in the picture below). A current, which is just moving charge, causes those charges to build up on one side, and the opposite charge to build up on the other side. But no actual charge is moving from one plate to the other.
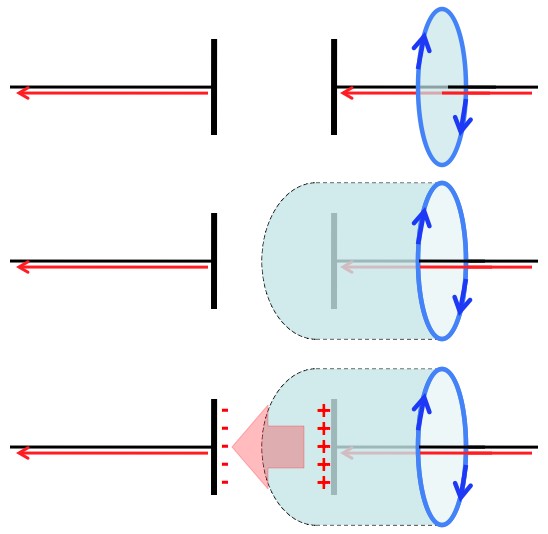
Pick a loop in space. If a current goes through a surface that spans that loop, then the current causes a magnetic field to run around that loop. But in a capacitor a changing electric field takes the place of the current.
So, if you were cruel enough to pick a spanning surface that goes through the capacitor, where there’s no current (middle diagram above), instead of through the wire, where there is a current (top diagram), you’d get a new and contradictory result.
But the situation itself hasn’t changed! No matter what surface you pick, the magnetic field around the loop has to be the same. It can’t be just current that makes the magnetic field curl around. So, what’s going on in the capacitor?
Well, as current flows into one side a positive charge builds up, and as current flows out of the other side a negative charge builds up. As a result, an increasing electric field appears in between the plates of the capacitor (bottom diagram).
The conclusion is that current () and changing electric fields (
) create curl in magnetic fields;
iv):
In terms of modern society, this is arguably the most important equation, since the behavior of electrical turbines are dictated by this equation. Like the last equation, this equation states that a changing magnetic field creates curl in the electric field.
If you have an electric field that curls in a circle, then you can generate current and electrical power.
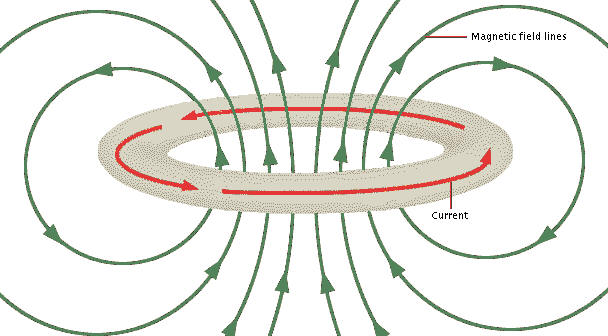
A changing magnetic field creates curl in the electric field. So if you increase the magnetic field through a loop of wire, there will be an electric field along the wire (tornado makes wind along the fence). When an electric field runs along a wire it pushes charges along it. Induced current!
So if you’ve got a loop of wire sitting next to a magnet, there will be no current. But, it you move that magnet, the magnetic field through the loop changes, and there will be current.
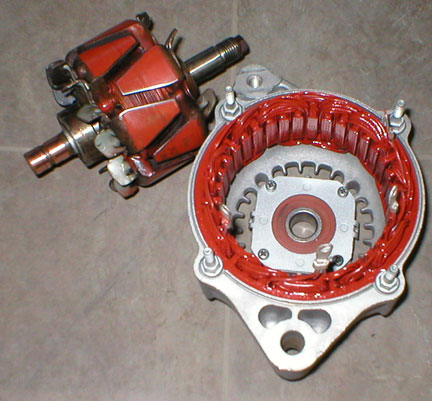
An automotive alternator. When spinning it will change the magnetic field through those loops of wire, and generate current.
Most power plants (hydro, nuclear, gas, coal, wind) simply spin generators, which (basically) move big magnets back and forth, changing the magnetic field through loops of wire and creating current. In fact, it changes the magnetic field through those loops 60 times a second, which is why the electrical power from your outlet, in turn, also switches 60 times every second (alternating current).
Solar panels are the glaring exception, but other than that, effectively all power is made the same way:
Notice, by the way, that unlike equation (iii), there’s no “J” term. J is moving electric charge. If there were such a thing as “magnetic charge”, then you could have “magnetic current”, and there would be a magnetic current term in equation (iv).
But there’s not.
The speed of light: Around the turn of the last century (1900) some smart people were noticing that Maxwell’s equations implied that changing electric fields created magnetic fields (iii) and that changing magnetic fields created electric fields (iv). They started wondering if electric and magnetic fields could sustain each other without the need for electrical charges or currents.
The short answer is yes. These self-sustaining fields are in fact light (isn’t that weird?). So when you see a chunk of sunlight, what you’re seeing is just a moving charge on the surface of the sun, which creates a changing electric field, which creates a changing magnetic field, which creates… until those fields interact with the chemicals in your eye and register as “light”, 93 million miles later.
Answer gravy:
The long answer requires some vector calculus. Take Mawell’s equations in vacuum (no charges, no currents):
I’ll just try to solve for the electric field, so taking the time derivative of (iii) and then plugging in (iv) yields:
Vector calculus is one of those corners of mathematics resplendent with an amazing array of identities that you pretend to memorize, but secretly look up in books 3 minutes before you’re tested. Here’s one:
I was thinking briefly about deriving this, but if you know (mathematically) what these symbols mean, then you can prove this yourself (it’s pretty easy). If you don’t, then it’s just scary. Back to the point:
Here I’ve used that there are no charges, so .
This last equation seems to be written in a very strange way, but check it! The general wave equation is written: , where A is a wave, and v is the propagation speed of that wave. So, the electric field E, propagates as a wave at
(light speed).
I suspect that when this was first figured out, the dudes involved shit themselves. At that time there had been no previous (direct) indication that electricity and magnetism had anything to do with light.
Even more profound, since all physical laws are independent of how fast you’re moving (physics is the same whether you’re moving or sitting still), there was now a speed that’s independent of how fast you’re moving. Relativity!








Isn’t the upside-down delta the gradient operator? Why does it seem to have a role as a variable in here?
It’s the gradient among other things, but in this post it’s only being used in divergences and curls. I should have defined it a bit more explicitly, but I didn’t want to get bogged down too much with math. It’s usually called the “del operator“, and it’s used all over the place in vector calculus in the various incarnations of the derivative you find there.
Nice !
I find it not scary but beautiful.
I ran across maxwells stuff when researching for the alternator equations.
I have a bit of a better understanding of Vector Calculus now with this article.
I find it awesome that the propagation is lightspeed, and leads to relativity.
(I knew it was just not WHY)
Now, I am going to have to go back and reread where Einstein got the fodder for his theory ! Astounding how it all ties in together.
-Watchman
Pingback: Q: How much of physics can be deduced from previous equations/axioms? | Ask a Mathematician / Ask a Physicist
Pingback: Q: What is “spin” in particle physics? Why is it different from just ordinary rotation? | Ask a Mathematician / Ask a Physicist
Pingback: Q: Why do nuclear weapons cause EMPs (electromagnetic pulses)? | Ask a Mathematician / Ask a Physicist
The magnetic field outside & at the end of solenoid looks diverging & converging, then why the divergence is zero there.
If you look at the picture in part (i), you’ll notice that when an area has zero divergence, them when you draw any “bubble” you’ll have the same number of lines entering as leaving. In the case of the solenoid, the field lines do get farther apart (which to any reasonable person would mean diverging), but since they never start or end anywhere, if a line enters a “bubble” it will exit it.
According to the mathematical definition of divergence (as opposed to the reasonable one), the magnetic field has zero divergence.
Pingback: Q: How would the universe be different if π = 3? | Ask a Mathematician / Ask a Physicist
good …………………………
Beautiful and simple explanation… thanks a lot!!
I never could understand Maxwell’s equations. Maybe that’s because I haven’t taken Calculus yet. 🙂
Pingback: LEARN: What Even is Pi? – Hate Love LEARN MATH
Great explanation !!!
Mainly due to the examples … thank you very much !!!
Pingback: Understanding Energy Interference | Chakra Healing Sounds