Physicist: When you’re doing math with numbers that aren’t known exactly, it’s necessary to keep track of both the number itself and the amount of error that number carries. Sometimes this is made very explicit. You may for example see something like “3.2 ± 0.08”. This means : “the value is around 3.2, but don’t be surprised if it’s as high as 3.28 or as low as 3.12… but farther out than that would be mildly surprising.”

120 ± 0.5 cm, due to hair-based error.
However! Dealing with two numbers is immoral and inescapably tedious. So, humanity’s mightiest accountants came up with a short hand: stop writing the number when it becomes pointless. It’s a decent system. Significant digits are why driving directions don’t say things like “drive 8.13395942652 miles, then make a slight right”. Rather than writing a number with its error, just stop writing the number at the digit where noises and errors and lack of damn-giving are big enough to change the next digit. The value of the number and the error in one number. Short.
The important thing to remember about sig figs is that they are an imprecise but typically “good enough” way to deal with errors in basic arithmetic. They’re not an exact science, and are more at home in the “rules of punctuation” schema than they are in the toolbox of a rigorous scientist. When a number suddenly stops without warning, the assumption that the reader is supposed to make is “somebody rounded this off”. When a number is rounded off, the error is at least half of the last digit. For example, 40.950 and 41.04998 both end up being rounded to the same number, and both are reasonable possible values of “41.0” or “41±0.05”.
For example, using significant figures, 2.0 + 0.001 = 2.0. What the equation is really saying is that the error on that 2.0 is around ±0.05 (the “true” number will probably round out to 2.0). That error alone is bigger than the entire second number, never mind what its error is (it’s around ±0.0005). So the sum 2.0 + 0.001 = 2.0, because both sides of the equation are equal to 2 + “an error of around 0.05 or less, give or take”.

2.0 + 0.001 = 2.0. The significant digits are conveying a notion of “error”, and the second number is being “drowned out” by the error in the first.
“Rounding off” does a terrible violence to math. Now the error, rather than being a respectable standard deviation that was painstakingly and precisely derived from multiple trials and tabulations, is instead an order-of-magnitude stab in the dark.
The rules regarding error (answer gravy below) show that if your error is only known to within an order of magnitude (where a power of ten describes its size), then when you’re done adding or multiplying two numbers together, what results will have an error of the same magnitude in the sense that you’ll retain the same number of significant digits.
For example,
![\begin{array}{ll} 1234\times 0.32 \\ = (1234 \pm 0.5)(0.32\pm 0.005) \\ = \left([1.234\pm 0.0005] 10^3\right)\left([3.2\pm 0.05] 10^{-1}\right) & \leftarrow\textrm{Scientific Notation} \\ = \left(1.234\pm 0.0005\right)\left(3.2\pm 0.05\right)10^2 \\ = \left(1.234\times3.2 \pm 1.234\times0.05 \pm 3.2\times0.0005 \pm 0.05\times0.0005\right)10^2 \\ = \left(3.9488 \pm 0.0617 \pm 0.0016 \pm 0.000025\right)10^2 \\ \approx \left(3.9488 \pm 0.0617\right)10^2 \\ \approx \left(3.9488 \pm 0.05\right)10^2 \\ = 3.9 \times 10^2 \end{array} \begin{array}{ll} 1234\times 0.32 \\ = (1234 \pm 0.5)(0.32\pm 0.005) \\ = \left([1.234\pm 0.0005] 10^3\right)\left([3.2\pm 0.05] 10^{-1}\right) & \leftarrow\textrm{Scientific Notation} \\ = \left(1.234\pm 0.0005\right)\left(3.2\pm 0.05\right)10^2 \\ = \left(1.234\times3.2 \pm 1.234\times0.05 \pm 3.2\times0.0005 \pm 0.05\times0.0005\right)10^2 \\ = \left(3.9488 \pm 0.0617 \pm 0.0016 \pm 0.000025\right)10^2 \\ \approx \left(3.9488 \pm 0.0617\right)10^2 \\ \approx \left(3.9488 \pm 0.05\right)10^2 \\ = 3.9 \times 10^2 \end{array}](//s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Bll%7D++++1234%5Ctimes+0.32+%5C%5C++++%3D+%281234+%5Cpm+0.5%29%280.32%5Cpm+0.005%29+%5C%5C++++%3D+%5Cleft%28%5B1.234%5Cpm+0.0005%5D+10%5E3%5Cright%29%5Cleft%28%5B3.2%5Cpm+0.05%5D+10%5E%7B-1%7D%5Cright%29+%26+%5Cleftarrow%5Ctextrm%7BScientific+Notation%7D+%5C%5C++++%3D+%5Cleft%281.234%5Cpm+0.0005%5Cright%29%5Cleft%283.2%5Cpm+0.05%5Cright%2910%5E2+%5C%5C++++%3D+%5Cleft%281.234%5Ctimes3.2+%5Cpm+1.234%5Ctimes0.05+%5Cpm+3.2%5Ctimes0.0005+%5Cpm+0.05%5Ctimes0.0005%5Cright%2910%5E2+%5C%5C++++%3D+%5Cleft%283.9488+%5Cpm+0.0617+%5Cpm+0.0016+%5Cpm+0.000025%5Cright%2910%5E2+%5C%5C++++%5Capprox+%5Cleft%283.9488+%5Cpm+0.0617%5Cright%2910%5E2+%5C%5C++++%5Capprox+%5Cleft%283.9488+%5Cpm+0.05%5Cright%2910%5E2+%5C%5C++++%3D+3.9+%5Ctimes+10%5E2++++%5Cend%7Barray%7D&bg=ffffff&fg=000&s=0)
This last expression could be anywhere from 3.8988 x 102 to 3.9988 x 102. The only digits that aren’t being completely swamped by the error are “3.9”. So the final “correct” answer is “3.9 x 102“. Not coincidentally, this has two significant digits, just like “0.32” which had the least number of significant digits at the start of the calculation. The bulk of the error in the end came from “±1.234×0.05”, the size of which was dictated by that “0.05”, which was the error from “0.32”.
Notice that in the second to last step it was callously declared that “0.0617 ≈ 0.05”. Normally this would be a travesty, but significant figures are the mathematical equivalent of “you know, give or take or whatever”. Rounding off means that we’re ignoring the true error and replacing it with the closest power of ten. That is, there’s a lot of error in how big the error is. When you’re already introducing errors by replacing numbers like 68, 337, and 145 with “100” (the nearest power of ten), “0.0617 ≈ 0.05” doesn’t seem so bad. The initial error was on the order of 1 part in 10, and the final error was likewise on the order of 1 part in 10. Give or take. This is the secret beauty of sig figs and scientific notation; they quietly retain the “part in ten to the ___” error.
That said, sig figs are kind of a train wreck. They are not a good way to accurately keep track of errors. What they do is save people a little effort, manage errors and fudges in a could-be-worse kind of way, and instill a deep sense of fatalism. Significant figures underscore at every turn the limits either of human expertise or concern.
By far the most common use of sig figs is in grading. When a student returns an exam with something like “I have calculated the mass of the Earth to be 5.97366729297353452283 x 1024 kg”, the grader knows immediately that the student doesn’t grok significant figures (the correct answer is “the Earth’s mass is 6 x 1024 kg, why all the worry?”). With that in mind, the grader is now a step closer to making up a grade. The student, for their part, could have saved some paper.
Answer Gravy: You can think of a number with an error as being a “random variable“. Like rolling dice (a decidedly random event that generates a definitively random variable), things like measuring, estimating, or rounding create random numbers within a certain range. The better the measurement (or whatever it is that generates the number), the smaller this range. There are any number of reasons for results to be inexact, but we can sweep all of them under the same carpet labeling them all “error”; keeping track only of their total size using (usually) standard deviation or variance. When you see the expression “3±0.1”, this represents a random variable with an average of 3 and a standard deviation of 0.1 (unless someone screwed up or is just making up numbers, which happens a lot).
When adding two random variables, (A±a) + (B±b), the means are easy, A+B, but the errors are a little more complex. (A±a) + (B±b) = (A+B) ± ?. The standard deviation is the square root of the variance, so a2 is the variance of the first random variable. It turns out that the variance of a sum is just the sum of the variances, which is handy. So, the variance of the sum is a2 + b2 and (A±a) + (B±b) = A+B ± √(a^2+b^2).
When adding numbers using significant digits, you’re declaring that a=0.5 x 10-D1 and b=0.5 x 10-D2, where D1 and D2 are the number of significant digits each number has. Notice that if these are different, then the bigger error takes over. For example, 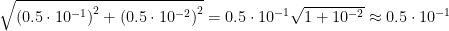 . When the digits are the same, the error is multiplied by √2 (same math as last equation). But again, sig figs aren’t a filbert brush, they’re a rolling brush. √2? That’s just another way of writing “1”.
. When the digits are the same, the error is multiplied by √2 (same math as last equation). But again, sig figs aren’t a filbert brush, they’re a rolling brush. √2? That’s just another way of writing “1”.

The cornerstone of “sig fig” philosophy; not all over the place, but not super concerned with details.
Multiplying numbers is one notch trickier, and it demonstrates why sig figs can be considered more clever than being lazy normally warrants. When a number is written in scientific notation, the information about the size of the error is exactly where it is most useful. The example above of “1234 x 0.32” gives some idea of how the 10’s and errors move around. What that example blurred over was how the errors (the standard deviations) should have been handled.
First, the standard deviation of a product is a little messed up:  . Even so! When using sig figs the larger error is by far the more important, and the product once again has the same number of sig figs. In the example, 1234 x 0.32 = (1.234 ± 0.0005) (3.2 ± 0.05) x 10-2. So, a = 0.0005 and b = 0.05. Therefore, the standard deviation of the product must be:
. Even so! When using sig figs the larger error is by far the more important, and the product once again has the same number of sig figs. In the example, 1234 x 0.32 = (1.234 ± 0.0005) (3.2 ± 0.05) x 10-2. So, a = 0.0005 and b = 0.05. Therefore, the standard deviation of the product must be:
![\begin{array}{ll} \sqrt{A^2b^2 + B^2a^2 + a^2b^2} \\[2mm] = Ab\sqrt{1 + \frac{B^2a^2}{A^2b^2} + \frac{a^2}{A^2}} \\[2mm] = (1.234) (0.05) \sqrt{1.0000069} \\[2mm] \approx(1.234)(0.05)\\[2mm] \approx 0.05 \end{array} \begin{array}{ll} \sqrt{A^2b^2 + B^2a^2 + a^2b^2} \\[2mm] = Ab\sqrt{1 + \frac{B^2a^2}{A^2b^2} + \frac{a^2}{A^2}} \\[2mm] = (1.234) (0.05) \sqrt{1.0000069} \\[2mm] \approx(1.234)(0.05)\\[2mm] \approx 0.05 \end{array}](//s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Bll%7D++++%5Csqrt%7BA%5E2b%5E2+%2B+B%5E2a%5E2+%2B+a%5E2b%5E2%7D+%5C%5C%5B2mm%5D++++%3D+Ab%5Csqrt%7B1+%2B+%5Cfrac%7BB%5E2a%5E2%7D%7BA%5E2b%5E2%7D+%2B+%5Cfrac%7Ba%5E2%7D%7BA%5E2%7D%7D+%5C%5C%5B2mm%5D++++%3D+%281.234%29+%280.05%29+%5Csqrt%7B1.0000069%7D+%5C%5C%5B2mm%5D++++%5Capprox%281.234%29%280.05%29%5C%5C%5B2mm%5D++++%5Capprox+0.05++++%5Cend%7Barray%7D&bg=ffffff&fg=000&s=0)
Notice that when you multiply numbers, their error increases substantially each time (by a factor of about 1.234 this time). According to Benford’s law, the average first digit of a number is 3.440*. As a result, if you’re pulling numbers “out of a hat”, then on average every two multiplies should knock off a significant digit, because 3.4402 = 1 x 101.
Personally, I like to prepare everything algebraically, keep track of sig figs and scientific notation from beginning to end, then drop the last 2 significant digits from the final result. Partly to be extra safe, but mostly to do it wrong.
*they’re a little annoying, right?
, is
. Not too likely.