Q: Is the Alcubierre warp drive really possible? How close are we to actually building one and going faster than light?

Posted on February 19, 2013 by The Physicist

The original question was: Is this f’real?

Astronomer: The Alcubierre spacetime metric is named after a Miguel Alcubierre, a physicist who was also a Star Trek fan. It describes a type of bubble in space, where you could put, for example, a spaceship named USS Enterprise. Within the bubble space is isn’t doing anything weird, but in front of the bubble you scrunch up space and behind the bubble you stretch it out. If you could create space that was warped in this particular way, you could sit in your spaceship inside the bubble and move space around you faster than light in a way that would make it seem like you’d traveled at speeds faster than light. In reality, only the space itself would have travelled faster than light, which doesn’t break the speed limit rules imposed by relativity.

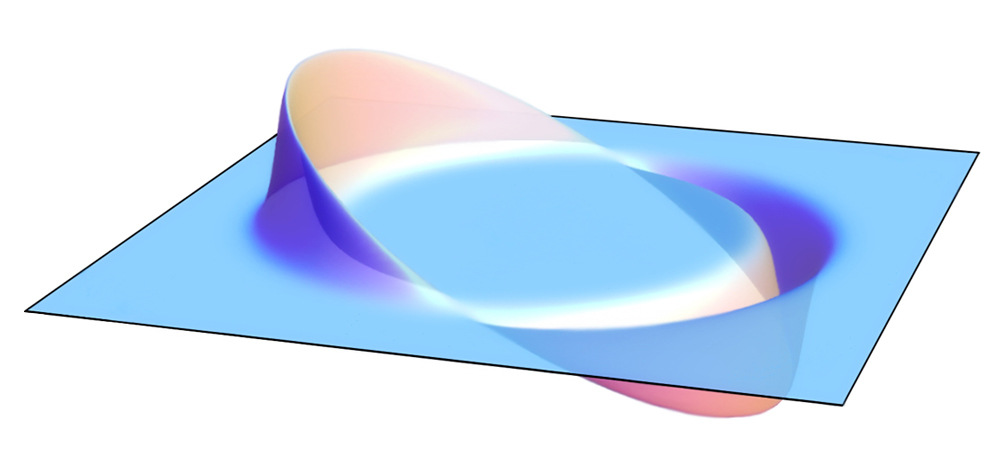
Bend some space like this and you can head for the second star to the right, and go straight on till morning!

Bend some space like this and you can head for the second star to the right, and go straight on till morning!

The Alcubierre metric is a real solution to Einstein’s equations, which describe the ways in which space is allowed to bend or warp. There is no theoretical reason to think space couldn’t bend in this way, except for one very major problem. In the equations that describe the Alcubierre metric, one of the mass terms has to be negative for things to work out.

Know where I can get some negative mass? Didn’t think so. Negative mass, which is fundamentally different than anti-matter, would experience gravity as a repulsive force. Too bad nobody has ever discovered any negative mass floating around out there or made any substantial amount it. You can make really tiny amounts of negative mass/energy for very short periods of time in the lab, by “borrowing energy from the uncertainty principle,” but it’s not clear this could be scaled up to the macroscopic level like it would need to be to create a useful warp bubble.

It’s probably best negative mass doesn’t exist, because it’s incredibly creepy! For example, imagine you had a chunk of normal mass and a chunk of negative mass in space. The negative mass would fall towards the regular mass, but at the same time create “repulsive gravity” which would then push the normal mass away. Lather, rinse, repeat, and you could theoretically accelerate those two chunks off into the stars forever. This smells suspiciously like perpetual motion, and would break the well established principle of conservation of energy. Given that, it’s understandable that we’re not packing the SPF 5000 for our super fast trip to Alpha Centauri Bb.

Physicist: It is for real, but don’t go reserving flights.  There has been some theoretical research into Warp drives for about 20 years starting, not surprisingly, within months of the end of TNG.  Back in the day Einstein figured out how mass/energy and the curvature of spacetime relate to each other with the narcissistically named “Einstein field equations“.  These equations tell you how spacetime will be stretched and curved in the presence of a distribution of matter (for example, put a bunch of matter in one place, and spacetime will “pucker” in such a way that we get gravity).  But these equations can be turned on their head; if you want spacetime twisted up in a particular way, you can find out how matter and energy need to be arranged to make that happen.

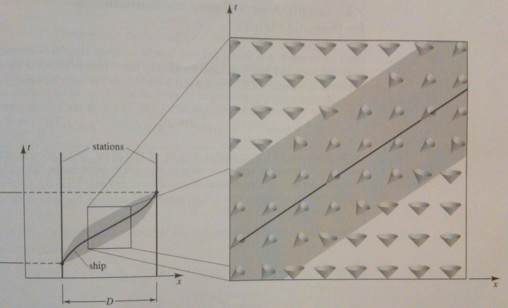
By bending spacetime in a particular way you can make it so that locally you move slower than light, but that the overall effect is faster-than-light travel.

By bending spacetime in a particular way you can make it so that locally you move slower than light, but that the overall effect is faster-than-light travel.  The arrangement of matter and energy that allows for this is unfortunately impossible.  This diagram is from page 145 of “Gravity”, by Hartle.

Warp drive research always hinges on the idea that we should be able to arbitrarily manipulate stunning amounts of energy, and moreover, that a hell of a lot of that energy can be negative (essentially, “magical freeze-ray” powered warp drives).  To date, we haven’t been able to isolate negative energy independent of a burst of positive energy (in any form), and there have been a few arguments (not proofs) that there may be an uncertainty-principle sort of thing that forces this to always be the case.  Negative energy and matter carry with them an amazing array of fundamental issues.  For example, if you bring matter and anti-matter together you get a big boom, resulting in a big increase in entropy (as light and new particles fly in every direction), but if you bring matter and negative-matter together they just quietly blink out, resulting in a big decrease in entropy (which is a thermodynamic no-no).

But! Assuming that negative energy can be harvested (even in theory), and very carefully controlled and manipulated in spectacular ways, and if a number of very fundamental theoretical issues can be ironed out, then this is totally a way to travel faster than light in a very Star Trek kind of way (as opposed to a BSG “jump drive“).  Warp drives have been in the news a bit recently because it was shown that there are “spacetime geometries” that require less of the very-probably-impossible negative-matter than was originally thought (presumably, also because warp drives are awesome).  This is a little akin to saying “my unicorn powered wish-making machine is now more feasible, because the unicorns can be any color, not just blue”.

For most physicists the Alcubierre drive is a cute tool for teaching general relativity, but in application smells a lot more like perpetual motion and anti-gravity machines than a device we’re ever likely to see.

There are some hopeful legit physicists, but not many.

Posted in -- By the Physicist, -- Guest Author, Physics, Relativity | 59 Comments

Q: Is darkness a wave the way light is a wave? What is the speed of dark?

Posted on February 14, 2013 by The Physicist

Physicist: The very short answer is no: darkness is not a wave.  There are no waves in the dark for very much the same reason that there’s no surfing (or ocean waves) in Death Valley.  Darkness, being an absence of electromagnetic waves (light) has nothing to do any waving.

There are waves in the ocean, because in the ocean there's stuff to do the waving.

There are waves in the ocean, because in the ocean there’s stuff to do the waving.  Having nothing to wave, deserts are waveless.  Darkness doesn’t involve waves because it’s a lack of electromagnetic waves.

If you define the speed of dark to be the speed at which you could find out about someone turning out the lights, it’s exactly the same as the speed of light: 671 million mph, or 2.99 x 108 m/s, or about 1 foot per nanosecond.  For example, if the Sun were to suddenly go out (unlikely), then the darkness wouldn’t get to the Earth for a solid 8 minutes.

The somewhat longer answer (that sound like it undermines the short answer, but totally doesn’t) is that “darkness”, while not a wave itself, can be produced by light waves.  Light waves, rather than merely being there or not can add and subtract from each other (at each tiny point in space).

You'd think something like light and dark would be an either/or sort of thing.

You’d think something like light and dark would be an either/or sort of thing, like eggs (for example).  But while eggs always add, light (and any other kind of wave) can add or subtract.

So if you’re looking at a particular point in space there can be light waves present, but they may cancel each other out, creating a little dark patch.  In this sense light has more in common with money than most “actual” things.  You can have positive money (colloquially; “bones”) or you can have negative money or debt (vernacularly; “boned”), and it’s not unless you have neither, or equal amounts of both, that you’re exactly broke.

White =1, Black = -1, Grey = 0

White=1, Black=-1, Grey=0. Notice that the patches are moving faster in-between the sources.

Light is similar.  Correctly prepared light can produce doubly bright or completely dark regions.  What’s really slick, is that these regions can move faster than light!  There are a lot of effects like this that get a lot of people excited, but before you get too excited; don’t.  It has to do with the difference between “phase velocity” and “group velocity”, but the basic idea is that these dark and light patches move faster than light in exactly the same way that the intersection of the blades of a pair of scissors move faster than either blade.  There’s an old post that talks about one of the more exceptional examples of this.

Long story short: darkness isn’t a wave itself because it isn’t anything, if it has a speed it’s the same as light speed, and also light is profoundly weird stuff (and not to be taken lightly).

 

The ocean, desert, basket, and hypnotic pictures are from here, here, here, and here.

Posted in -- By the Physicist, Philosophical, Physics | 40 Comments

Q: Is it a coincidence that a circles circumference is the derivative of its area, as well as the volume of a sphere being the antiderivative of its surface area? What is the explanation for this?

Posted on February 9, 2013 by The Physicist

Physicist: For those of you not hip to the calculus groove, here’s what’s going down:  The derivative of Y with respect to X, written \frac{dY}{dX}, is just a description of how fast Y changes when X changes.  It so happens that if Y=X^N, then \frac{dY}{dX}=NX^{N-1}.  So, for example, if Y=5X^3, then \frac{dY}{dX} = 15X^2.  The area of a circle is \pi R^2, and the circumference is 2\pi R, which is the derivative.  The volume of a sphere is V = \frac{4}{3}\pi R^3, and the surface area is S = 4\pi R^2, which is again the derivative.  This, it turns out, is no coincidence!

If you describe volume, V, in terms of the radius, R, then increasing R will result in an increase in V that’s proportional to the surface area. If the surface area is given by S(R), then you’ll find that for a tiny change in the radius, dR, dV = S(R)dR, or \frac{dV}{dR} = S(R).

You can think of

You can think of a sphere as a series of very thin surfaces added together.  This is another, equivalent, way of describing the situation.  Each layer adds (surface area of layer)x(thickness of layer) to the volume.

You can think of this like painting a spherical tank. The increase in volume, dV, is the amount of paint you use, and the amount of paint is just the surface area, S(R), times the thickness of the paint, dR.  This same argument can be used to show that the volume is the integral of the surface area (just keep painting layer after layer).

It’s a little easier to keep track of what’s going on with circles.

If you increase the radius of a circle by a tiny amount, dR, then

If you increase the radius of a circle by a tiny amount, dR, then the area increases by (2πR)(dR).

The same “derivative thing” holds up for the circumference vs. the area of a circle.  The change in area, dA, is dA = (2πR)dR.  So, \frac{dA}{dR}=2\pi R.  That is, the derivative of the area is just the circumference.

This, by the way, is one of the arguments for using “τ” instead of “π”. τ = 2π, so the area of a circle is \pi R^2 = \frac{1}{2}\tau R^2. This makes the “differential nature” of the circumference a little more obvious.

Answer gravy: By the way, this argument is only exact when the thickness of the new layer “goes to zero“.  Basically, the top of the new layer is a little longer/bigger than the bottom of the new layer.  So if the area is A=\pi R^2, and the change in radius is \Delta R, then \Delta A = \pi(R+\Delta R)^2 - \pi R^2 = \pi R^2+2\pi R\Delta R + \pi (\Delta R)^2-\pi R^2 = 2\pi R\Delta R + \pi (\Delta R)^2

This extra little \pi (\Delta R)^2 is a result of the top and bottom of the new layer being very slightly different lengths.  But when ΔR is small, (ΔR)2 is really small.  For example, if ΔR is one thousandth, then (ΔR)2 is one millionth.  The whole idea behind calculus is that when the scales get very small you can just ignore these “extra tiny” terms.  In fact, this is the essential difference between dA and ΔA, and how the derivative is defined:

\frac{dA}{dR}=\lim_{\Delta R\to 0}\frac{\Delta A}{\Delta R} = \lim_{\Delta R\to 0}\frac{1}{\Delta R}\left(2\pi R\Delta R + \pi (\Delta R)^2\right) = \lim_{\Delta R\to 0} (2\pi R + \pi\Delta R) = 2\pi R
Posted in -- By the Physicist, Math | 14 Comments

Q: If hot air rises, why is it generally colder at higher elevations?

Posted on February 3, 2013 by The Physicist

Physicist: Beautiful question!

The short answer is that if you have a warm bubble of air it will rise, but as it does it’ll expand in the lower pressure environment, which causes it to cool.

Weather balloons are intentionally under-filled on the ground so that when they're at altitude they'll be the right size.

Weather balloons are intentionally under-filled on the ground so that when they’re at altitude they’ll be the right size.  Shown here are professional weather scientists (left) and other (right).

If you’re not familiar with the ideal gas law (compression heats, expanding cools), then here’s a better way to think about it.  Gases (which includes air) can be though of as a hell of a lot of perfectly bouncy rubber balls.  Each molecule is ricocheting very fast between other molecules and the ground.  But, like anything that moves, when they move upward they slow down.

Air molecules are like rubber balls.

Air molecules are like rubber balls.  They move faster when lower and slower when higher.  Air not shown.

This random movement is what we in the biz’ call “heat energy”.  Heat is literally just the random motion of atoms.  When an air molecule is close to the ground it’s moving pretty fast, but if it has a chance to bounce higher, then it will slow down during the trip.  When it’s a little higher, all of the other air molecules around will have had similar experiences and will also be moving a little slower.  Slower motion = lower temperature.

You can extend this a bit and say that air near the surface of the Earth is moving about as fast as it would be if it had been dropped from the top of the atmosphere (ignoring air resistance, ironically), which is about half a kilometer per second at room temperature.  This is a little ballpark, because the atmosphere is more complicated than air just being stacked up.

When a ball or air molecules are bouncing, they exchange “kinetic energy” for “gravitational potential energy“, but overall the total energy stays the same.  So air actually maintains the same amount of total energy regardless of height, it’s just a question of how much of that energy is tied up in gravitational potential (being up high) or kinetic energy (moving fast).  When a vertical column of air has the same amount of energy, then “bubbles” of air are free to move up and down, and those bubbles will have the same temperature of the surrounding air when they arrive.

An inversion layer, where the temperature suddenly increases as you go up, stops air from mixing properly.

However, sometimes this isn’t the case.  Temperature drops as you go up, but hot air still rises through cold air.  If you’re moving upward and find that suddenly the temperature increases (instead of slowly decreasing), then you’ve hit an “inversion layer“.  This happens a lot in valleys and canyons.  The warmer layer has more energy than the colder layer just beneath it (so it’s hotter and lighter), and this stops the air layers from mixing.  The cooler air doesn’t have the extra energy needed to rise through the warmer air above it, so the air layers stack up like oil on water.  Since the layers are just sitting on top of each other, they’re free to behave very differently.  If you’ve ever been outside and seen clouds at different altitudes moving at different speeds or different directions, then you’re probably looking at clouds separated by an inversion layer or two.

The rubber ball picture is from an over-the-top ad, that can be found here.

Posted in -- By the Physicist, Physics | 10 Comments

Q: What is quantum teleportation? Why can’t we use it to communicate faster than light?

Posted on January 29, 2013 by The Physicist

Physicist: Contrary to its exciting name, quantum teleportation doesn’t involve any physical stuff suddenly disappearing and then reappearing somewhere else.  Instead it’s a cute, clever, technique for transferring an unknown quantum state of one system (usually a single particle) to another, specially prepared, system.  That sounds a lot cooler and more interesting than the reality.  Before even getting into quantum teleportation, I’ll describe how to do “classical teleportation”, and you can judge whether “teleport” is even the appropriate word.  This is great at parties (or, more likely, Dr. Who marathons at your mom’s house)!

Classical teleportation:

i) Get three coins (hereafter called A, B , and C), and set up B and C to be the same side up (but which side is up should be completely random).

ii) Flip A.

iii) Compare A and B.  If they’re the same, then leave C alone.  If they’re different, then turn C over.

iv) What was the heads/tails state of A is now the state of C.

This was the result of a google search for "ugliest coin". Doesn't seem that bad.

B and C start the same. If A and B are the same, then C and A are already the same. If A and B are different, then flipping C will make A and C the same.  This works independently of the heads/tails configurations (as long as B and C start the same), so you don’t even need to know what faces are up.

What’s deeply spooky about this is that you can set it up so that the state of A is transmitted to C, without ever knowing what the states of any of the coins are.  In step one make B and C the same, then while holding them together, turn them over several times until you’ve lost track of how many times you’ve done it, then cover them.  They’ll be the same, but you won’t know which side they both have up.  Do the same sort of thing in step three: bring A and B together, turn them over several times, then see if they’re the same or different.

Or just get someone else to do it for you.

The “teleportation” comes in because you can move C as far away as you like.  Once you compare A and B you can then shout (or, for a long distance teleport, call on the phone) “same” or “different”, and whoever has C can leave it alone or flip it over.  The unknown state of A has been teleported to C.  Notice that, until you tell C’s owner about the result of the same/different measurement, the state of C has absolutely nothing to do with the state of A.  This is the big limitation of teleportation and why it isn’t faster than light; you have to communicate the results of the measurement, and that communication is through ordinary, not-faster-than-light channels.  Without that communication C remains random and has nothing to do with A.

Whether or not this trick qualifies as remarkable, or even deserves the moniker “teleportation”, is a matter of personal preference.  That said, this brand of teleportation can send physical objects from place to place exactly as effectively as an email can.

Ever notice that the moment Riker is left in charge everything goes horribly wrong?

Quantum teleportation is not this. Not this at all.

Quantum teleportation has the same limitations and works very similarly.

Answer gravy: And here’s how.  There are several teleportation schemes and, believe it or not, this is the simplest.

The basic idea is the same as the coin trick; you start with two particles, B and C, that are entangled.  Then you bring in particle A that’s in an unknown state and compare it to B (in a way that doesn’t involve directly measuring the states of either).  Then the results of that comparison are communicated, and C is altered.

A “qubit“, is like a regular “bit” (or a coin), except that instead of just 0 or 1 (heads or tails) it can be in a combination of both.  What is physically meant by 1 or 0, or how a qubit is manipulated, depend on the system involved.  It could be electron spins manipulated by magnets, or photon paths manipulated by beam splitters, or a dozen other things.  Doesn’t really matter.

The notation here is a little tricky, but stay with me.  In what follows “|\cdot\,\cdot\,\cdot\rangle” is the notation for a state of all three qubits.  So, for example, |101\rangle is the state A=1, B=0, and C=1.  In quantum mechanics things can be in more than one state at once.  For example, B and C will start in the entangled state \frac{1}{\sqrt{2}}|00\rangle+\frac{1}{\sqrt{2}}|11\rangle, which means that they’re in a 50/50 combination of “both zero” and “both one”.

That √2 is there because when you actually do a measurement, the probability of finding a particular state is the coefficient squared.  This is called the “Born Rule“, and don’t worry about it.  Those √2’s are mostly just clutter.

"Do stuff" is an extremely technical term.

How to quantum teleport the state of A onto C.

The initial state of all three qubits, “I” in the picture above, is:

\begin{array}{ll}(\alpha|0\rangle+\beta|1\rangle)(\frac{1}{\sqrt{2}}|00\rangle+\frac{1}{\sqrt{2}}|11\rangle) \\= \frac{\alpha}{\sqrt{2}}|000\rangle+\frac{\beta}{\sqrt{2}}|100\rangle+\frac{\alpha}{\sqrt{2}}|011\rangle+\frac{\beta}{\sqrt{2}}|111\rangle\end{array}

Where the state of A, \alpha|0\rangle+\beta|1\rangle, which is the state to be teleported, is completely unknown (α and β are unknown).  The first thing that’s done is a “Controlled Not Gate”.  An ordinary Not Gate flips the bit, |0\rangle\to|1\rangle and |1\rangle\to|0\rangle.  But a Controlled Not has a “control bit” and a “target bit”.  If the control bit is 0, then nothing happens, and if the control bit is 1, then the target bit is flipped.  In this case, A is the control bit and B is the target.  This interaction makes the state of A and the state of B entangled.  After the Controlled Not the state of the three qubits, “II” in the picture above, is:

\frac{\alpha}{\sqrt{2}}|000\rangle+\frac{\beta}{\sqrt{2}}|110\rangle+\frac{\alpha}{\sqrt{2}}|011\rangle+\frac{\beta}{\sqrt{2}}|101\rangle

Notice that after the CNot gate the qubits can no longer be factored apart, the way the state at I could be factored.  This is symptomatic of entangled systems.  Next comes the “Hadamard Gate”, which only exists for quantum systems.  CNot gates can be done in a regular computer, but the Hadamard Gate is all quantum, baby.  Here’s what it does: |0\rangle\to\frac{1}{\sqrt{2}}|0\rangle-\frac{1}{\sqrt{2}}|1\rangle and |1\rangle\to\frac{1}{\sqrt{2}}|0\rangle+\frac{1}{\sqrt{2}}|1\rangle.  This step is hides what state A started out as, so when a measurement is done (in a moment) you don’t directly measure A.  This is important, because if you measure a state it collapses*.  So instead of having \alpha|0\rangle+\beta|1\rangle, you’re left with just |0\rangle or just |1\rangle, which are different from A.  After applying the Hadamard Gate to A, the state of the three qubits, “III” in the picture above, is:

\frac{\alpha}{2}|000\rangle-\frac{\alpha}{2}|100\rangle+\frac{\beta}{2}|010\rangle+\frac{\beta}{2}|110\rangle+\frac{\alpha}{2}|011\rangle-\frac{\alpha}{2}|111\rangle+\frac{\beta}{2}|001\rangle+\frac{\beta}{2}|101\rangle

This can be rearranged a bit,

\frac{1}{2}|00\rangle(\alpha|0\rangle+\beta|1\rangle) + \frac{1}{2}|01\rangle(\beta|0\rangle+\alpha|1\rangle) + \frac{1}{2}|10\rangle(-\alpha|0\rangle+\beta|1\rangle) + \frac{1}{2}|11\rangle(\beta|0\rangle-\alpha|1\rangle)

Now when A and B are measured, they can have one of four possible results, 00, 01, 10, and 11.  When a measurement is made, then what remains are all of those states consistent with the measurement.  For example, if A is measured to be 1, then the state remaining is \frac{1}{\sqrt{2}}|10\rangle(-\alpha|0\rangle+\beta|1\rangle) + \frac{1}{\sqrt{2}}|11\rangle(\beta|0\rangle-\alpha|1\rangle), and if B is then found to be 0, then the state remaining is |10\rangle(-\alpha|0\rangle+\beta|1\rangle).

Each of the four results will mean that C is left in one of four states:

\begin{array}{cc} \textrm{Result} & \textrm{State of C} \\ 00 & \alpha|0\rangle+\beta|1\rangle \\ 01 & \beta|0\rangle+\alpha|1\rangle \\ 10 & -\alpha|0\rangle+\beta|1\rangle \\ 11 & \beta|0\rangle-\alpha|1\rangle \end{array}

If the person in charge of C (the Custodian?) is then told the results, no matter how far away they are, they can perform some basic fixes.  For example, if the result is 00, then they leave the state alone.  If the result is 01, they run C through a Not gate (which switches 0 and 1).  For the others a “Phase Gate” (another quantum-only kind of logic gate) is used, which takes |0\rangle\to|0\rangle and |1\rangle\to-|1\rangle.

Here’s the important bit.  Just like the “classical teleportation” above, without knowledge of what the results of the AB measurement were, the person in charge of C (the Controller?) can’t turn C into the original A state.  If they were to measure C, without doing any of the fixes, they’d find that the probability of getting a zero or a one is exactly 50%, instead of |α|2 and |β|2 which it should be (again, by the Born rule).  Each of the four measurements are equally likely, and the chance of getting a “1” is the sum of chances from each state: 0.25(|β|2+|α|2+|β|2+|-α|2) = 0.25(1+1) = 0.5 (since |β|2+|α|2=1).

You may suspect that there’d be some way to manipulate C, without getting the results of the measurement, that would allow you to overcome this lack of information.  Turns out: nope.  It’s much harder to see why, and it dips into quantum information theory, but the proof is fairly “straightforward”.  This is just for those of you who really feel like looking this stuff up, so it’ll be fast.

The “probability density matrix” for C, before any information is received, is \left[\begin{array}{cc}\frac{1}{2}&0\\0&\frac{1}{2}\end{array}\right] or one half times the identity matrix (those halves are the 50/50 probabilities from a moment ago).  You can figure this out by “tracing out” all of the unknown components (A and B).  Everything that you can do to the state C, including all of the stuff mentioned earlier like Not gates, and Hadamard Gates, and whatnot, amounts to a coordinate change on the probability density matrix.  But since it’s essentially just the identity matrix, none of them change anything.  The α’s and β’s of the original state never show up in C (not even a little) before the results of the measurement (00, 01, 10, or 11) are communicated.

So once again, without ordinary communication, nothing about A is conveyed to C, and if you only had access to C, you’d never know that a measurement was even done.

*Not really.  When quantum systems interact they become entangled.  From inside of a system this looks like “wave function collapse” which causes no end of paradoxes and problems, but from outside of the systems involved interactions don’t cause collapses, just entanglement.  There’s a whole post about this brain trip here.

Posted in -- By the Physicist, Entropy/Information, Physics, Quantum Theory | 28 Comments

Q: Since all particles display wave-like characteristics, does that imply that one could use destructive wave interference to destroy or at least drastically change a particle?

Posted on January 24, 2013 by The Physicist

Physicist: The wave-like property of particles allows you to do a lot of cute things with particles that would otherwise seem impossible, but making a particle disappear isn’t one of them.  You can use destructive interference to make it very unlikely to find a particle in particular places, which is exactly what’s happening in the double slit experiment, and is also the basis behind how “diffraction gratings” work.

Lasers are one of the big reasons to go into physics. Sure, there's the whole "understanding the nature of reality" thing, but it's mostly about lasers.

The wave nature of things (in this case light) can be used to get it to go places it normally wouldn’t (right), or not go places it normally would (left).  By using two slits you can interfere the light waves to cause the light to never show up in places where it would when passing through one slit (dark bands in in the lower left).

But in order to destroy a particle using destructive interference you’d need to ensure that there was nowhere left with any constructive interference.  In the pictures above there are dark regions, but also light regions.  Turns out there are some very solid mathematical principles and physical laws that don’t allow waves to behave that way (not just waves in particle physics, but waves in general), not the least of which is the conservation of energy.  So, say you’ve got some kind of waves bouncing around (light waves, sound waves, particle fields, whatever), and you want to make new waves to cancel them out.  You can certainly do that, but only in small regions.

If everyone on the air plane just turned off their noise-cancelling headphones, then the flight would be almost silent.

How noise canceling headphones work.  Rather than destroying sound around them, they cancel out the sound on one side that’s coming from the other.  But ultimately they’re just speakers that make the environment louder overall.

The new waves add energy to the overall system, so no matter how hard you try to cancel things out, you’ll always end up adding energy somewhere, it’s just a question of where.  Noise canceling headphones are a beautiful example.  They actively produce sound in such a way that they destructively interfere with sound waves coming from nearby, in the anti-headward direction.  Although they create quiet in the ears, they create noise everywhere else.  There’s no getting around it.

Yes; this is art.

Say you’ve got a bunch of noise bouncing about.  Noise canceling techniques can create a quite region, but end up generating more total noise outside of that region.

Similarly, you could try to kill off particles by creating new waves in the particles field (each kind of particle has a corresponding field, for example light particles are called “photons” and they have the electromagnetic field) that cancel out the particle.  You can totally create a particle “dead zone”, assuming you know exactly what the particle’s wave form is.  Headphones need this as well; you can’t just get rid of sound, you first need to know exactly what the sound is first.  However, anything you try will end up adding more particles , and won’t destroy the particle you’re worried about anyway, just redirect it.

There are a few ways to destroy a particle.  You can chuck it out (which is as good as destroying it, for most practical purposes), or you can annihilate it.  But annihilation is a bit of a cheat; you just put a particle in a chamber with its anti-particle and let nature take its course.  But that doesn’t use waves and interference (in the way we normally think of waves), they just combine, disappear, and leave behind a burst of energy.  But not in a cool, wavey interferey, kind of way.

The pictures at the top of this post are from here and here.

Posted in -- By the Physicist, Particle Physics, Physics, Quantum Theory | 4 Comments