Ask a Mathematician / Ask a Physicist

Q: Is the edge of a circle with an infinite radius curved or straight?

Posted on April 21, 2011 by The Physicist

Physicist: Straight.

In fact, in mathematics the “curvature” of a curve is usually defined as the “reciprocal of the radius of the osculating circle”. This is fancy talk for: fit a circle into the curve as best you can, then measure the radius of that circle, and flip it over.

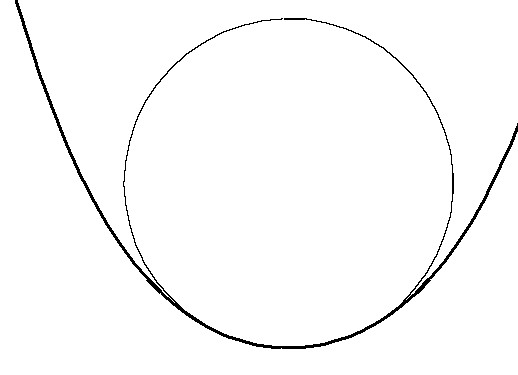
At any given point the osculating (or "kissing") circle is the circle that fits a curve as closely as possible.

It makes sense; a smaller circle should have a higher curvature (it’s turning faster) but it has a smaller radius, R. So, use 1/R, which is big when R is small, and small when R is big. There are some more technical reasons to use 1/R (like that you can apply it directly to calculating the centrifugal force on a point following that path, or that it gives rise to the entirely kick-ass Descartes’ theorem), but really it’s just one of the more reasonable definitions.

So, just working with the standard definition, you say: the curvature of a circle is 1/R, if I let the radius become infinite, the curvature must go to zero. Zero curvature means no bending of any kind. Must be a line.

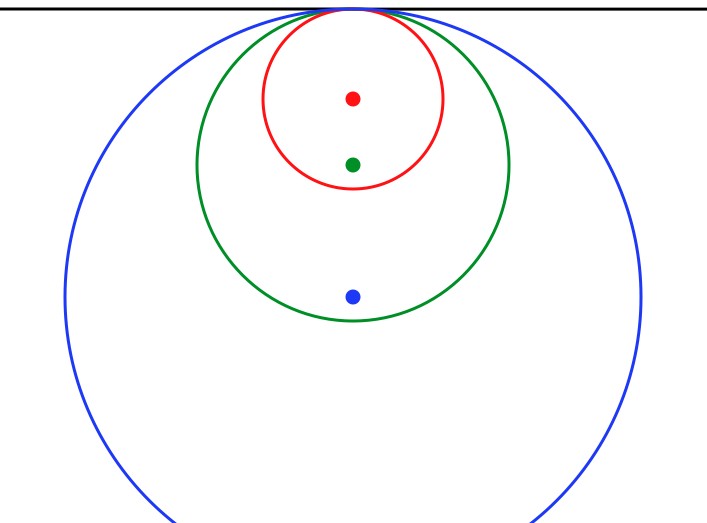
Nail down the edge of a circle. As the center gets farther and farther away, the radius gets larger, and the curvature gets smaller. When the "circle is centered at infinity" the curvature drops to zero, and the edge becomes a straight line (black).

Old school topologists get very excited about this stuff.

Say you have two lines on a plane. They’ll always intersect at exactly one point, unless they’re parallel in which case they’ll never intersect at all. But the Greek Geometers, back in the day, didn’t like that; they wanted a more universal theorem. So they included the “line at infinity” with their plane, and created the “projective plane”. In so doing they created a new space where every pair of straight lines intersect at one point, no matter what.

To picture this, imagine the ground under your feet as the plane and the horizon as the line-at-infinity.

The tracks are parallel, so they'll never meet. But, they look like they meet at the horizon. So why not (mathematically speaking) "include" the horizon and define that as the place where the tracks meet?

Parallel lines meet at two points on the horizon (in opposite directions). So the line at infinity is weirdly defined with opposite points on the horizon being the same point. Mathematicians would say “antipodal points are identified”. In the projective plane two lines always meet at one point.

Notice that east-west parallel lines meet at the east-west point on the line at infinity, and north-south parallel lines meet at the north-south point at infinity. So you do need the entire horizon, not just a single “far away point”. A single point would yield the Riemann sphere, which is also good times.

Back to the point. If you think about circles in this new-fangled projective plane, one of the first questions that comes to mind is “what happens if the circle includes a point on the line at infinity?”.

No matter how big the circle is, or where its center is, the whole thing will always be in the plane (not all the way out to the line at infinity). If the circle does have a point on the horizon, then you’ll find that the center also has to be at infinity (if it’s in the plane, then it’ll be closer to some points on the circle than others, but the center point is the same distance to every point on the circle). Specifically, the center will be on the line at infinity exactly 90° away from where the circle intersects the line.

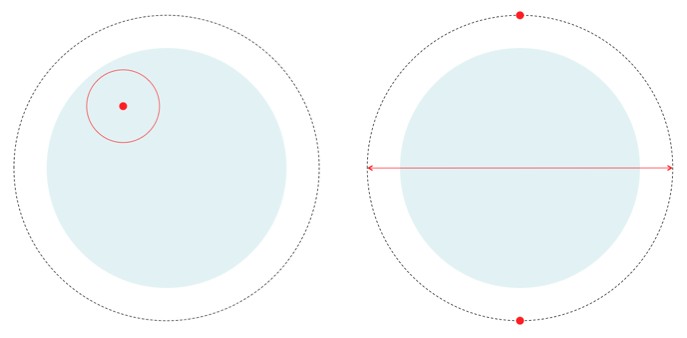
The projective plane, which includes the usual infinite plane (light blue) and the line at infinity (dashed line), and two examples of circles. Note that, although the plane is infinite, the line at infinity wraps around it in the same way that the horizon would still wrap around you even if the Earth were flat and infinite (this is an abstract picture). Left: a circle and its center in the in the ordinary plane. Right: a circle that passes through the east-west point on the line-at-infinity. Its center is at the north-south point on the line-at-infinity.

This agrees surprisingly well with the intuition behind the more colorful circle picture above. Science and math truly are a beautiful tapestry of interconnections and nerding right the hell out.

Posted in -- By the Physicist, Geometry, Math | 26 Comments

Q: As a consequence of relativity, objects becomes more massive when they’re moving fast. What is it about matter that causes that to happen?

Posted on April 17, 2011 by The Physicist

Physicist: The laws of the universe are relativistic. That is, Einstein was right and Newton, although accurately and intuitively describing the world around us, was wrong. When you try to translate the cleaner Einstein laws into a Newtonian form you find that the mass, M, has a $\gamma$ sitting next to it. Normally it has almost no effect, but at very high speeds it becomes important. The Newtonian interpretation (where you don’t allow for messed up time and space, but you do allow for alchemy) of that $\gamma$ is that the mass is “exaggerated” by a factor of $\gamma$ .

$\gamma$ is an excellent way to talk about how much relativity is having an impact on distance, time, and mass. For those of you who’d like to do some back-of-the-envelope relativity $\gamma=\frac{1}{\sqrt{1-\beta^2}}$ , where $\beta$ is the fraction of the speed of light that the object in question is traveling ( $\beta = \frac{v}{c}$ ).

The source of the $\gamma$ may be obscure, but you’ll find that when you try to write the laws of the universe (which prefer relativity) in a form we’re used to it always seems to show up.

Matter does two big things: it has inertia, and it makes gravity. Every attempt to measure matter always comes down to one or both of these properties.

Inertial mass means that matter is difficult to push around. This is summed up by Newton’s second law of motion: F=MA.

(Historical side note: Newton himself originally wanted to call this the “second law of suck it Leibnitz“, but in committee he was talked down to the more demure “Newton’s second law”. At one time Newton was discovering as many as 9 new Leibnitz-insulting laws a week.)

Here’s a thumbnail sketch of an example of $\gamma$ showing up:

It turns out that, in relativity, the true law is: f=Ma. (Notice the difference?)
Einstein realized that in order to rewrite Newton’s laws for relativity he needed to be careful about whose time he was talking about. As it happens, the correct choice is the on-board time of the moving mass (which is what a clock on the object would read). So here I’m using lower-case to talk about force and acceleration in terms of on-board time, and upper case to talk about them in terms of “world time”, which is the usual time we’re used to talking about.

The ratio of the “on-board time” ( $\tau$ ) to the “world time” (T) is $\gamma$ .
As a (non-obvious) result, $f = \gamma F$ . A (hand-waving) way to think about this is “if I push (F) that space ship for a while (T), they’ll think I was pushing (f) longer, because their clock ( $\tau$ ) is ticking slower”.
At the same time, that acceleration (a) is in terms of on-board time, but if you want A (the acceleration to everyone else) you have to write it in terms of world time. Acceleration is distance per second per second, so:
$a=\frac{d}{\tau^2}=\frac{T^2}{\tau^2}\frac{d}{T^2}=\gamma^2\frac{d}{T^2}=\gamma^2 A$
and so
$\begin{array}{ll}f=Ma\\\Rightarrow\gamma F=Ma\\\Rightarrow\gamma F=M\gamma^2 A\\\Rightarrow F=\gamma MA\end{array}$

So if you look at the forces involved in pushing an object (that’s moving so fast that $\gamma$ is noticeable), you find that it’s harder to push than it “should” be (by a factor of $\gamma$ ). The easiest way to interpret that is as an increase in mass.

You could just as easily say that “force mysteriously gets weaker” by looking at this last equation as: $\frac{1}{\gamma}F=MA$ . But that wouldn’t be consistent with the interpretation of…

Gravitational mass, which creates gravity and pulls other matter toward it, is the second property of matter. But matter isn’t really what creates gravity, it’s energy.

For example, if you had a crazy big laser beam (all energy, no matter), that beam would have some gravity. Similarly, if you have a moving rock (one with kinetic energy) it will have more gravity than a perfectly stationary one. And the kinetic energy of an object with mass M is given by $K=\gamma Mc^2$ , which is very, very close to $Mc^2+\frac{1}{2}Mv^2$ (the “rest mass energy” plus the “Newtonian energy“). Point is: there’s another $\gamma M$ .

These last statements are wrist-breakingly hand-wavy, but they do hold up. The Frankenstein created by combining gravity and relativity is called “general relativity”, and it’s nasty enough that cute tricks like “multiply by $\gamma$ ” don’t come close to telling the whole story, or even enough to be useful.

Posted in -- By the Physicist, Physics, Relativity | 12 Comments

Q: What is the evidence for the Big Bang?

Posted on April 10, 2011 by The Physicist

Physicist: The very short answer is: all the galaxies in the universe are flying apart, so at some point in the distant past they must have been very close together. It would have been so close and so dense that all the matter in the universe would have been extremely hot.
It so happens that, since light takes a while to get from place to place, we can actually see that early age of the universe by looking really far out. That ancient heat now takes the form of the “cosmic microwave background”, which we can see with radio telescopes (and hear with regular radios). We can then follow the rough history of the universe forward. By looking at distant galaxies we can even see how the universe has changed up until now.

The Hubble Ultra Deep Field survey. Although this is not a "true color" picture, almost all of the color variation originates from the "cosmological red shift", an effect caused by the expansion of space which increases with distance. This square is less than one eighth the size of the moon on each side, and it was selected because it's one of the emptiest regions in the sky.

The longer answer is a bit more specific. Back in 1929 Edwin Hubble (of telescope fame) discovered that the farther things are away from us, the faster they’re receding.

Hubble's original data. Errors are often big in astronomy (and even bigger in astrology). The data has gotten a lot better since then. Image remorselessly stolen from: http://www.astro.ucla.edu/~wright/cosmo_01.htm

The first question that came to his mind was: “what?” followed shortly after by “what does this say about the universe in the distant past?”
If you look at the motion of all of the matter in the universe and “run time backward” you find that somewhen around 15 billion (give or take) years ago the distance between everything would have been zero. Having everything pressed together early on implies that the universe would have been ludicrously hot, and that the universe we see today is composed of the cooled off, still flying apart, remnants of that early super-furnace.

Btw, the term “Big Bang”, an understated name coined by cosmologist Fred Hoyle, really doesn’t convey either the bignness nor the bangness of the beginning of the universe. Other suggested names include the fairly popular “Horrendous Space Kablooie!”.

Excellent suggestion.

Oddly enough, the Big Bang isn’t a simple as a big explosion happening somewhere, followed by all the matter of the universe flying away like shrapnel. Immediately after an explosion you’ll find that the material involved is moving in every direction, at many different speeds. But you can say even more; the distribution of those speeds is roughly Gaussian, so the faster the speed, the less stuff you’ll find traveling that fast.

The distribution of matter resulting from an explosion. This is not how matter is distributed in the universe. Not at all.

So, if the Big Bang were just an explosion somewhere, then the distribution of the matter in the universe would follow suit: the more distant matter would be the fastest stuff, and there wouldn’t be much of it.

However, that’s not at all what we see. Instead, the galaxies we see are distributed (roughly) evenly throughout the universe. Cosmologists call this “homogeneity”, and the chance of an explosion producing it by accident is zero.

So, the universe is expanding, and yet it’s not expanding from a single point. This, and a wide variety of other bizarre (complicated) effects, can be easily explained by “metric expansion”. A good way to talk about metric expansion is to think of space as the surface of an expanding balloon.

Metric expansion. Even without moving, objects find themselves farther apart. Even more profound, there's no center to the expansion. Every point is as good as any other.

Draw a couple points on a balloon with a pen and then blow it up (pardon, “inflate it”). I’ll wait.

You’ll notice that the points move away from each other at a speed proportional to the distance between them, and that the points aren’t really moving at all.
There’s a nice symmetry here in that no point is at the center of the expansion. In addition (and this is what makes it real science) the metric expansion description provides a testable hypothesis: you should be able to see what the universe looked like when it was extremely small and hot.

When you look out into space you see the heat of the early universe. Shown here is a sketch of where in the early universe the light that we'll eventually see is emitted (left), and the situation now, when we receive that light. Notice that there's nothing special about our location, every point in the universe experiences the same thing.

If the universe started as an explosion in one location, then the light from it would be long gone, and if we looked way out into space (and far back in time) all we would see is an extremely empty ancient universe. But again, that’s not what we see.

At about the same time that Hubble and his bosom buddies in the theoretical cosmology community were contemplating this stuff a couple of astronomers testing out a new radio telescope (“the Horn”) found themselves saddled with intractable equipment problems. They wanted to use radio waves to look at stars, but before they started they decided to zero-out their equipment by pointing the Horn at the emptiest patch of sky they could find. However, they soon found that the entire sky has a uniform radio hum that was completely independent of direction. This is now called the “Cosmic Microwave Background” (CMB), and it’s the oldest stuff in the universe (other than everything else).

"The Horn" is the radio telescope that first (accidentally) detected the microwave background. To this day no one knows why it's called the Horn. Some mysteries are just too big.

Originally it was thermal radiation from the extremely hot early universe. “Hot”, by the way, is an understatement. Imagine a fire that isn’t red or white hot, but “gamma ray hot”. As in, the light it produces would irradiate you. However, because of the metric expansion it has since been red-shifted (a lot) and now has such a low frequency that it’s barely noticeable.

Light (being a wave, that's bigger than a point) gets stretched out by the expansion of space. What started out as very high-frequency, short-wavelength light has become low-frequency, long-wavelength light (specifically: microwaves).

If you tune your radio to an empty channel a substantial fraction of the static you’ll hear is the CMB (especially at night, the sun is “noisy”).

Unfortunately, when matter gets hot enough it becomes ionized, and ionized stuff is good at scattering light (that’s why you can bounce radio transmissions off of the ionosphere). So, the CMB, the farthest back we can physically see, is from the “photon decoupling event“, when the universe cooled enough for the matter to become non-ionized and allow light to pass safely through. This happened around 400,000 years after the big bang, which seems like a lot, but it’s only about 0.003% of the universe’s current age.

Most of the other evidence for the big bang is a bit more complicated and circuitous. For example, cosmological computer models that describe how galaxies form and move tend to work very well using the big bang as a starting point, and tend to work not even a little otherwise.

With very careful analysis (using general relativity and detailed observations of the universe today) we can talk about things even farther back than the photon decoupling event, down to within a second of the big bang itself.

However, at time zero it’s hard to say much of anything with any kind of certainty. The closer you get to the first moments of the universe, the louder and more cantankerous physicists become. You’d be hard pressed to find a cosmologist who disagrees that the big bang happened, and just as hard pressed to find two who agree on all the details.

Posted in -- By the Physicist, Astronomy, Physics | 13 Comments

Q: Is there a formula to find the Nth term in the Fibonacci sequence?

Posted on April 8, 2011 by The Physicist

Physicist: Hells yes! It’s $f_n \approx \frac{1}{\sqrt{5}} \left(\frac{1+\sqrt{5}}{2}\right)^{n+1}$ , where the “≈” is close enough that you can round to the nearest integer. Astute readers will notice that $\frac{1+\sqrt{5}}{2}$ is the golden ratio, and may wonder if this is a coincidence. Yes.

Everything after this is a detailed, math-heavy explanation of where this formula comes from.

The “Fibonacci sequence” is defined as a sequence of numbers $f_0, f_1, f_2, \cdots$ such that you have the recursion: $f_n = f_{n-1}+f_{n-2}$ , and the restrictions: $f_0 = 1$ and $f_1 = 1$ .

Explicitly, the Fibonacci sequence is: 1, 1, 2, 3, 5, 8, 13, 21, … That is, the recursion says that every term is the sum of the previous two.

You can also talk about “generalized Fibonacci sequences”, where these restrictions and/or the recursion are changed. For example: $f_n = 2f_{n-1}+3f_{n-2}$ , with $f_0 = 5$ and $f_1 = 2$ . This derivation is for the ordinary sequence, but it can be altered to suit any generalized Fibonacci sequence. Here it is:

Every now and again it’s useful to encode a string of numbers in a “generating function“. For obscure (and unimportant to this post) reasons, you can write many functions as infinitely long polynomials. For example: $\sin{(x)} = x-\frac{1}{6}x^3+\frac{1}{120}x^5 \cdots$ . The generating function for a sequence of numbers $f_0, f_1, f_2, f_3, \cdots$ is $g(x) = f_0 + f_1 x + f_2 x^2 + f_3 x^3 + \cdots = \sum_{n=0}^\infty f_n x^n$ . So, sin(x) is the generating function for the sequence $0, 1, 0, -\frac{1}{6},0,\frac{1}{120}, \cdots$ . If you can find a simple form for this function g, then bully. You’ve got a very straight forward way of writing an infinite string of numbers. The value of x doesn’t have anything to do with anything. The powers of x are really just there to keep the numbers straight. Now check this out!

$\begin{array}{ll}g(x) = \sum_{n=0}^\infty f_n x^n\\xg(x) = \sum_{n=0}^\infty f_n x^{n+1} = \sum_{n=1}^\infty f_{n-1} x^n \\x^2 g(x) = \sum_{n=0}^\infty f_n x^{n+2} = \sum_{n=2}^\infty f_{n-2} x^n\end{array}$

You can take the recursion and use it to find a relationship between these three slightly different functions. Here’s a good first guess: $g(x) = xg(x)+x^2g(x)$

You can write this out, group by powers of x, and then use the recursion. However (if you look at the definitions above for g, xg, and x²g), each sum starts at a different value of n. That needs to be dealt with first:

$\begin{array}{ll}i)&\sum_{n=0}^\infty f_nx^n=\sum_{n=1}^\infty f_{n-1}x^n+\sum_{n=2}^\infty f_{n-2}x^n\\ii)&\Rightarrow f_0+f_1x+\sum_{n=2}^\infty f_n x^n=f_0x+\sum_{n=2}^\infty f_{n-1}x^n+\sum_{n=2}^\infty f_{n-2}x^n\\iii)&\Rightarrow f_0+f_1x+\sum_{n=2}^\infty f_nx^n=f_0x+\sum_{n=2}^\infty (f_{n-1}+f_{n-2}) x^n\\iv)&\Rightarrow f_0+f_1x=f_0x+\sum_{n=2}^\infty (f_{n-1}+f_{n-2}-f_n)x^n\\v)&\Rightarrow f_0+f_1x=f_0x\\vi) &\Rightarrow 1+x =x\end{array}$

ii) pulling out the n=0,1 terms

iii-iv) grouping by powers of x

v) f_n = f_n-1+f_n-2

vi) f₀ =1 and f₁=1

This doesn’t quite line up, so the guess wasn’t perfect. There should have been an extra “+1” on the right side of the original equation: $g(x) = xg(x)+x^2g(x)+1$

Armed with this latest equation we can actually solve for g:

$\begin{array}{ll}g(x)=xg(x)+x^2g(x)+1\\\Rightarrow -1=xg(x)+x^2g(x)-g(x)\\\Rightarrow (x^2+x-1)g(x)=-1\\\Rightarrow g(x)=\frac{-1}{x^2+x-1}\\\Rightarrow g(x)=\frac{-1}{(x-R_+)(x-R_-)}\end{array}$

Here $R_+ = \frac{-1+\sqrt{5}}{2}$ and $R_- = \frac{-1-\sqrt{5}}{2}$ . The only reason for writing it this way is that leaving all those roots and fractions in makes this look like a math blizzard.

So far, using what is known about the Fibonacci sequence, we’ve found a nice closed equation for the generating function (g), which “encodes” the sequence. Hopefully, we can use this fancy new equation to figure out what each f_n must be. Again, the function (g) itself does nothing. The only reason it’s around is so that we can look at the coefficients when it’s written in the form of a (Taylor) polynomial.

Now using “partial fractions” you can pull this one kinda-complicated fraction into two not-so-complicated fractions (that’s where the “ $\frac{1}{\sqrt{5}}$ ” comes from):

$\begin{array}{ll} g(x)=\frac{-1}{(x-R_+)(x-R_-)}\\=\frac{1}{\sqrt{5}}\left[\frac{-1}{x-R_+}+\frac{1}{x-R_-} \right]\\=\frac{1}{\sqrt{5}}\left[\frac{1}{R_+-x}-\frac{1}{R_--x} \right]\\=\frac{1}{\sqrt{5}}\left[\frac{1}{R_+}\frac{1}{1-\frac{x}{R_+}}-\frac{1}{R_-}\frac{1}{1-\frac{x}{R_-}} \right]\end{array}$

It so happens (and this is the point of the entire excercise) that functions of the form “ $\frac{1}{1-\square}$ ” can be written as: $\frac{1}{1-\square} = \sum_{n=0}^\infty \square^n = 1+\square+\square^2+\square^3+\cdots$ . This is called a “geometric series“. For example: $1+\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\cdots = \sum_{n=0}^\infty \left(\frac{1}{2}\right)^n = \frac{1}{1-\frac{1}{2}} = 2$ .

So, with that in mind:

$\begin{array}{ll} g(x)=\frac{1}{\sqrt{5}}\left[\frac{1}{R_+}\frac{1}{1-\frac{x}{R_+}}-\frac{1}{R_-}\frac{1}{1-\frac{x}{R_-}} \right]\\=\frac{1}{\sqrt{5}}\left[\frac{1}{R_+}\sum_{n=0}^\infty \left(\frac{x}{R_+}\right)^n -\frac{1}{R_-}\sum_{n=0}^\infty \left(\frac{x}{R_-}\right)^n \right]\\=\frac{1}{\sqrt{5}}\left[\sum_{n=0}^\infty \left(\frac{1}{R_+}\right)^{n+1}x^n -\sum_{n=0}^\infty \left(\frac{1}{R_-}\right)^{n+1}x^n \right]\\=\sum_{n=0}^\infty \frac{1}{\sqrt{5}}\left(\frac{1}{R_+}\right)^{n+1}x^n -\sum_{n=0}^\infty \frac{1}{\sqrt{5}}\left(\frac{1}{R_-}\right)^{n+1}x^n\\=\sum_{n=0}^\infty \left[\frac{1}{\sqrt{5}}\left(\frac{1}{R_+}\right)^{n+1}-\frac{1}{\sqrt{5}}\left(\frac{1}{R_-}\right)^{n+1}\right]x^n\\\end{array}$

But g was originally defined as $g(x) = \sum_{n=0}^\infty f_n x^n$ . These f_n in front of each power of x must be the same as these weird things above.

$\begin{array}{ll}f_n = \frac{1}{\sqrt{5}}\left(\frac{1}{R_+}\right)^{n+1}-\frac{1}{\sqrt{5}}\left(\frac{1}{R_-}\right)^{n+1}\\= \frac{1}{\sqrt{5}}\left(\frac{2}{-1+\sqrt{5}}\right)^{n+1}-\frac{1}{\sqrt{5}}\left(\frac{2}{-1-\sqrt{5}}\right)^{n+1}\\= \frac{1}{\sqrt{5}}\left(\frac{2}{-1+\sqrt{5}}\frac{1+\sqrt{5}}{1+\sqrt{5}}\right)^{n+1}-\frac{1}{\sqrt{5}}\left(\frac{-2}{1+\sqrt{5}}\frac{1-\sqrt{5}}{1-\sqrt{5}}\right)^{n+1}\\= \frac{1}{\sqrt{5}}\left(\frac{1+\sqrt{5}}{2}\right)^{n+1}-\frac{1}{\sqrt{5}}\left(\frac{1-\sqrt{5}}{2}\right)^{n+1}\end{array}$

Notice: 1) $\frac{1+\sqrt{5}}{2} = \phi$ is the golden ratio (not that it matters), and 2) $\left|\frac{1-\sqrt{5}}{2}\right| \approx 0.6$ . This means that the second term is smaller than one, and each successive power is progressively smaller. So, rather than calculate it, ignore it!

Were you so inclined you could take any initial conditions (the f₀ and f₁) and any recursion (of the form f_n = Af_n-1+Bf_n-2) and, using the method above, find a closed form for it as well. The only problem you may run into is finding yourself with a polynomial that can’t be factored (x²+x-1 had factors, but it needn’t have). If that happens, that’s bad…

Don’t know what to tell you.

Posted in -- By the Physicist, Equations, Math | 22 Comments

Q: Why is the integral/antiderivative the area under a function?

Posted on April 4, 2011 by The Physicist

Physicist: If you’ve taken calculus, then at some point you learned that to find the area under a function (generally written $\int_A^B f(x) \, dx$ ) you need to find the anti-derivative of that function. The most natural response to these types of theorems is “wait… what?… why?”.

This theorem is so important and widely used that it’s called the “fundamental theorem of calculus”, and it ties together the integral (area under a function) with the antiderivative (opposite of the derivative) so tightly that the two words are essentially interchangeable. However, there are some mathematicians who may take issue with mixing up the two terms.

It comes back (in a roundabout way) to the fact that the derivative of a function is the slope of that function or the “rate of change”. In what follows “f” is a function, and “F” is its anti-derivative (that is: F’ = f).

Intuitively: Say you’ve got a function f(x), and the area under f(x) (up to some value x) is given by A(x).

Then the statement “the area, A, is given by the anti-derivative of f” is equivalent to “the derivative of A is given by f”.

In other words, the rate at which the area increases (as you slide x to the right) is given by the height, f(x).

For a constant function the area is given by A=cx, and the rate of increase (the amount that the area increases if x increases by 1) is c. Whether or not the function moves around makes no difference. From moment-to-moment the rate of increase is always equal to the height (the value of f).

For example, if the height of the function were 3, then, for a moment, the area under the function is increasing by 3 for every 1 unit of distance you slide to the right. Keep in mind that the function can move up and down as much as it wants. As far as the function “knows”, at any particular moment it may as well be constant (dotted line in picture above).

So if the height of the function (which is just the function) is the rate at which the area changes, then f is the derivative of the area: A’=f. But that’s exactly the same as saying that the area is the anti-derivative of the function.

Mathematically: There’s a theorem called the mean value theorem that states that if you have a “smooth” function with no sudden bends or kinks, then over any interval the derivative will be equal to the average slope at least once. This needs a picture:

Given a smooth function f, there's a point c where the function has the same slope as the overall average slope.

More precisely, if you have a function on the interval [A,B], then there’s a point c between A and B such that $f^\prime (c) = \frac{f(B)-f(A)}{B-A}$ . You can just as easily write this as $f^\prime (c) (B-A) = f(B)-f(A)$ or $f(c) (B-A) = F(B)-F(A)$ (since F’ =f).

So if you drive 60 miles in one hour, then at some instant you must have been driving at exactly 60 mph, even though for almost the entire trip you may have been traveling much faster or much slower than 60 mph.

Keep that stuff in the back of your mind for a moment, and ponder instead how to go about approximating the area under a function.

You can approximate the area under a function by dividing it up into a whole lot of tiny rectangles. The area of each is the width times the height, where the height is any value of f in that particular interval. Choosing different values does change the area of that rectangle, but it turns out that that doesn't matter.

You can divide up the area between x=A and x=B under a function by putting a mess of rectangles under it. Divide up the interval [A,B] by picking a string of points x₀, x₁, x₂, …, x_N, and use these as the left and right sides of your rectangles (and set x₀=A and x_N=B).

The point, c_i, that you pick in between each x_i-1 and x_i is unimportant. To get the exact area you let N, the total number of rectangles, go flying off to infinity, and you’ll find that the highest value of f and the lowest value of f in each tiny interval gets squeezed together.

So, why not choose a value of c_i so that in each rectangle you can say $f(c_i) (x_i-x_{i-1}) = F(x_i)-F(x_{i-1})$ ?

$\begin{array}{ll}area \\\approx \sum_{i=1}^N f(c_i) (x_i-x_{i-1}) \\= \sum_{i=1}^N F(x_i)-F(x_{i-1}) \\= \left\{ \begin{array}{ll}F(x_1)-F(x_0)\\+F(x_2)-F(x_1)\\+F(x_3)-F(x_2)\\ \cdots \\ +F(x_{N-1})-F(x_{N-2})\\+F(x_N)-F(x_{N-1})\end{array} \right\}\\= F(x_N) - F(x_0)\\= F(B) - F(A)\end{array}$

Holy crap! The area under the function (the integral) is given by the antiderivative! Again, this approximation becomes an equality as the number of rectangles becomes infinite.

As an aside (for those of you who really wanted to read an entire post about integrals), integrals are surprisingly robust. That is to say, if your function has a kink in it (the way |x| has a kink at zero, for example) then you can’t find a derivative at that kink, but integrals don’t have that problem. If there’s a kink or even a discontinuity; no problem!

You can just put the edge of a rectangle at the problem point, and then ignore it. In fact, think of (almost) any function in your head… You can take the integral of that. It may have an infinite value, or something awful like that, but you can still take the integral.

To make a function that can’t be integrated you have to make it infinitely messed up. Mathematicians live for this sort of thing. There is almost nothing in the world they enjoy more than coming up with ways to break each other’s theories. One of the classic examples is the function $f(x) = \left\{ \begin{array}{ll} 0,&\textrm{when x is a rational number}\\1,&\textrm{when x is an irrational number}\end{array}\right.$

Over any interval you pick, f still jumps around infinitely often, so the whole “things will get better as the number of rectangles increases” thing can never get off the ground. There are fixes to this, but they come boiling and howling up out of the ever-darker, stygian abyss that is measure theory.

Posted in -- By the Physicist, Equations, Math | 59 Comments

Thank you!

Posted on April 2, 2011 by The Physicist

Dear reader, we are excited to announce that, for the first time in the history of the universe, AskAMathematician.com has broken 100,000 page views in a single month! This is significant because it is approximately the number of fingers that 10,000 people have.

Thank you to our readers for:

1. Being awesome.
2. Having good taste.
3. Getting our jokes (such as they are).

$\vec{U} \cdot \vec{R} = 2 G(R)^8$

May
$e^{i \pi} + 1 = 0$
forever.