Q: If you zoom in far enough, what do particles look like?

Posted on November 15, 2010 by The Physicist

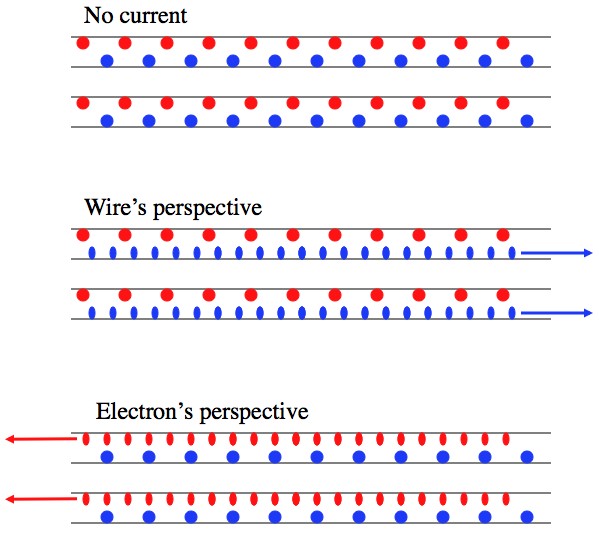
The original question was: According to Gertrude Stein “There is no there there.”  But is there “something” there?  I guess we know that atoms are “there,” but what about the constituent parts?  Electrons, protons, quarks, etc.  Are they really “there,” or do we fall back on Heisenberg and say there is nothing really there, just a point particle at some probability?  When and/or where is there finally something with a spatial dimension?  At the Planck length?  But my real question is this:  If we could completely stop an elementary particle’s forward motion and say “there it is,” and then take a really, really high res picture of it (indulge me here), what would we see and what would it look like?  A cloud of energy whizzing around looking like heat waves in the desert?  A sphere made of “quark matter”?  Or suppose we had a few billion-billion-billion pure quark particles stuck together in a jar (further indulge me here), what would we see?

Physicist: The best way to think about matter is as a “quantized field”, like an electric field (the “quanta” of the electric field are photons).  Unfortunately, nothing really has a size on the atomic level.  If you see an ocean wave you can ask “where is that wave?” or “how big is that wave?” and get a good ballpark answer.  But when you start asking “where exactly is that wave?” you start running into problems (try it).

You can see more or less where the wave is, but can you say for sure where it is to within a millimeter? Hells no! Fundamental uncertainty!

The Heisenberg uncertainty principle isn’t so much about having uncertainty about some definite particle, it’s a statement about the nature of particles in general.  That is to say, the particle itself is uncertain, not just the measurement apparatus.  It’s not just that we can’t tell where a particle is, and how fast it’s moving, it’s that the particle isn’t actually at a particular place/speed.  There’s a pretty nasty post here that covers how we can tell the difference.

Fortunately, it barely matters.  You can never say exactly where a wave is, but that doesn’t change the fact that it’s there.  You can still interact with it, and if you’re working on a large enough scale, the wave seems to be in a very definite (but not perfectly definite) place, and may as well be a particle.

A really high res picture would look like a blurry dot (sorry, no way around it).

Color, on the other hand, you can talk about.  Matter can absorb, transmit, reflect, or emit light.  For example: rocks absorb most light and reflect rock-colored light, glass transmits visible light and absorbs infrared and ultraviolet light, and glow in the dark stuff emits light.

In order to absorb light the material in question has to have energy levels such that the difference between two of them is equal to the energy of the incoming photon.  For individual atoms this is just the atomic spectra.  For more complex materials you get energy levels from the vibrations, and interactions between atoms.  For something like a rock this covers pretty much every possible incoming energy, so it can absorb almost any kind of light.

There’s a lot of nasty mathematical physics that governs how likely a photon with a given energy is to be absorb, reflected, or transmitted.  The situation is so complicated and difficult to predict, that (by far) the best way to figure out the optical properties of a material are to make that material and then look at it.

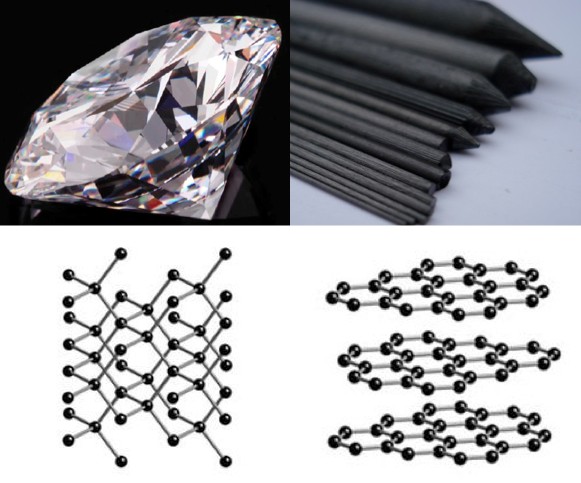
Diamond and graphite are made of exactly the same stuff (pure carbon). Their wildly different optical properties are caused entirely by how the atoms are arranged. If we hadn't dug this stuff out of the ground, I don't think anyone would have predicted this. Theoretical optical physics is nasty.

Due to quantum chromodynamics (one of the best buzzwords ever), a bunch of quarks will always appear in the form of larger composite particles (protons, neutrons, kaons, pions, etc).  So being unable, even in theory, to make this stuff, you’re left with guesswork.  With a wild guess, for not particularly good reasons, I would guess that a jar full of quarks would be clear in the visible spectrum.

Posted in -- By the Physicist, Particle Physics, Physics, Quantum Theory | 2 Comments

Q: What would you experience if you were going the speed of light?

Posted on November 13, 2010 by The Mathematician

The original question was: If I’m moving at the speed of light towards you and I throw a tennis ball at you (at, say, 5 m/s), what do you observe? Will I hit you first, the tennis ball, or both at the same time, or will something else happen entirely? The problem that I’m having thinking about this is that if the tennis ball did hit you first, then it would have been moving faster than the speed of light (relative to you). On the other hand, if it were moving at the speed of light relative to you, then it was moving at the same speed as me relative to you, thus both myself and the tennis ball will hit you simultaneously. If this is the case, however, then the tennis ball would have been moving at a speed of 0 m/s with respect to me.

Mathematician: First of all, let me point out that you will never travel at the speed of light (see this for details). It would take an infinite amount of energy to get anything with mass (e.g. you or your mama) going at that speed. Burning all the oil (and plants, and animals) on our planet and converting them into kinetic energy would get you going really fast, but would give you exactly 0% of the total energy that you would actually need to get going that speed (since any number divided by infinity is zero). But that doesn’t mean that it wouldn’t be fun to speculate about what would happen if you were going at light speed.

Due to a relativistic effect known as time dilation, the faster that you move with respect to some object O, the more time slows down for the object O (from your perspective). This isn’t just an issue of you seeing clocks attached to O tick slowly, they actually DO tick slowly from your vantage point (no experiment you could possibly do would conclude otherwise). There is symmetry though. You moving past O at 10,000 miles per hour is indistinguishable (as far as the laws of physics are concerned) from O moving past you at 10,000 miles per hour. That means, from the perspective of a person strapped to O, clocks attached to you are ticking slowly (i.e. your time is slowed down). As you approach the speed of light (with respect to O), this time dilation effect becomes more and more pronounced. When you are exactly AT the speed of light (impossible, but bare with me) no time whatsoever will elapse for O (i.e. a clock strapped to O will stop ticking completely) from your perspective. The upshot of this is that you’ll get wherever you are going without witnessing any time pass for anything not moving along with you. One reason this is really trippy is because if we view light coming from a distance object (such as a far away sun), from our perspective it might have taken years to get from us. But from the perspective of the photon (i.e. the light particle) no time will have elapsed on the journey! Yes, true physics is even weirder than crazy person made up physics.

A possibly even wackier effect crops up as the result of length contraction (another consequence of relativity). If you move towards object O at a fast speed you will notice that O will be compressed (i.e. shrunk) along the direction of your motion. So if O is a hippo, and you are going fast enough, it will look like one damn flat hippo.

Um, something like this I guess?

As you approach the speed of light, this effect becomes increasingly pronounced, and at at the speed of light itself O will have zero length in the direction you are traveling. In particular, if you are on a straight race track, and traveling at the speed of light, the race track will be compressed to zero length so that the starting line and the finishing line will be on top of each other. The race will be over as soon as it begins.

Another consequence of light speed travel is that you’d become the most dangerous thing imaginable (move over, Chuck). Since your mass is positive, infinite speed implies that your momentum is infinite. Hence, if you crashed into anything (and you would…after all, from your perspective the universe is flatter than a pancake in the direction you’re heading) it would get hurtled at insane speeds (since it would absorb some of your momentum). Of course, you’d also be dead pretty much instantly as you collided with object after object (each traveling at the speed of light with respect to you). And no, armor wouldn’t help.

Okay, so now to address the original question. What would happen if while traveling at the speed of light towards me you attempted to throw a ball at me? The answer is that you would have no time to actually do the throwing, because from your perspective you would run into me instantly. At which point, if I had any ninja skills, I would probably break those out. If you were traveling at near the speed of light (with respect to me), but not quite at it, and then threw the ball at 5 m/s (with respect to you) in my direction, the velocities would not simply add like you would expect based on Newtonian mechanics. Instead, you’d have to apply relativistic velocity addition which is a bit more complicated. In particular, the speed of the ball with respect to me will be less than the sum of your speed and 5 m/s. At low speeds this effect is not noticeable (speeds are additive to very close approximation), but at speeds close to the speed of light the effect becomes very pronounced.

Posted in -- By the Mathematician, Physics, Relativity | 56 Comments

Q: Why is pi not a definite number?

Posted on November 13, 2010 by The Physicist

The original question was: If the diameter of a circle is a fixed number, say 10cm, why does Pi go on for billions of numbers even though it extrapolates from the diameter’s value? Why is it not a definite number?

Mathematician: Basically, a diameter of 10cm leads to a circumference that is an irrational number. There is a very simple relationship between diameter and circumference, given by circumference = \pi\timesdiameter. It just so happens that the proportionality constant is an irrational number, which means that it has no [finite length] patterns in its digits that repeat forever. This occurs because, well, that is a property that circles have. \pi is however a “definite” number, in the sense that it is a single fixed number that is well defined. It’s just that since its digits don’t have a single pattern, there is no way to write them out nicely. Note that when you write 10 this is the same thing as 10.00000000000000…. with an infinite number of zeros. We can write this just as 10 because it’s understood that this implies that all other digits after the decimal point are 0. So, it’s not that 10 doesn’t have an infinite number of digits (like \pi), it’s just that those digits are zero (unlike \pi) which makes it easy to come up with a notation for ten (namely, 10).

The definition of Pi. This image is from http://www.mathsisfun.com/geometry/circle.html

Posted in -- By the Mathematician, Geometry, Math | 7 Comments

Q: What came before the big bang?

Posted on November 11, 2010 by The Physicist

Physicist: Sadly, very few photographs survive from the early universe, and even fewer from before the big bang.  So the best answers to this question are speculation or (if you’re feeling generous) informed speculation.

Other Big Bangs: Maybe the universe has expansion/collapse cycles?  Each cycle would start with a Big Bang, expand, level out, contract, and end with a “Big Crunch” and a “Big Bounce” (the next cycle’s Big Bang).  One of the advantages of this theory is that it removes the difficulties that come with the universe being infinitely small at some point (singularities = bad).  This is an old theory, but worth mentioning.  Since it was first proposed we’ve gotten much better measurements of the universe’s rate of expansion and found that it’s speeding up (not slowing down, as this theory would imply).  So it’s pretty unlikely that previous incarnations would have gotten around to crunching.  Or this is the last incarnation of the universe.  If so, live it up.

The Big Bounce.

Nothing Special: In some versions of M-theory the universe is seen as a four dimensional (3+1 dimensional) “brane” (as in “membrane”) floating around in an even higher dimensional space.  If this higher dimensional space were then full of more branes, and should they collide, you’d have a whole lot of energy exploding out of the impact area (in both universes).  A really “Big Bang”, as it were.  These collisions would need to be very, very rare and/or very, very far apart to get the universe we see today (the result of only one collision, not more).  Although the people working on this theory are seriously hot shit, it still seems a little out there.  That empty space can collide with other empty space is a little unintuitive.  But, you know…  String theory.

Colliding universes, infinite energy, and good times. This image stolen from http://media.radiosai.org/Journals/Vol_06/01APR08/04-musings.htm

South of the south pole: I think the best, and also least satisfying, answer is: “It’s the beginning.”  With respect to this very question, Hawking was once heard to type “[Asking about time before the universe] would be like asking for a point south of the South Pole.  It is not defined.”  So, “time” and “space” are strictly native to the universe.  Questions that start with “How long before the universe…” and “How far from the universe…” are like “how much paper to blue?”.  They don’t make sense.  A nice thing about this theory is there’s no longer any reason to ask about “before” or “cause”.  This also removes any need for asking questions like “why did the big bang happen when it did?”.

Time has a farthest back the same way Earth has a farthest south.

Super-universe: The universe may have “bubbled off” of a much larger universe.  One proposed mechanism for this bubbling is black holes.  Stuff that falls into black holes is completely isolated from everything outside, and weirdly the time it experiences must be completely independent of the flow of time outside the black hole.  So, dude… What if all the matter that falls into a black hole is fed into the big bang of a new universe, that buds off of our own?  And what if our universe  is just all the stuff that fell into a really big black hole in a universe farther up stream?  If the new universes thus created had slightly different laws, then that would lead to universes with more black holes being more likely.  Universe evolution!  Although interesting, this theory is not being widely pursued.

Perhaps new universes are created by old universes through black holes. These images stolen from: "http://revolution.groeschen.com/2009/05/15/birth-of-a-universe.aspx" and "http://www.onset.unsw.edu.au/issue7/mutiverse.html"

This guy: Hey, you can’t prove God doesn’t exist.  Can you?  No you can’t.  This theory is very popular.

Despite being outside the purview of even basic scientific investigation, I would be remiss in not mentioning this guy. This image stolen from: http://www.creativeuncut.com/gallery-05/gow2-zeus.html

Posted in -- By the Physicist, Philosophical, Physics | 18 Comments

Q: How do “Numerology Math Tricks” work? (adding digits and tricks with nines)

Posted on November 5, 2010 by The Physicist
The original question was: Please help me solve this math card trick, I do not understand how it works:
If you take a deck of 52 cards and split it into any number of piles (lets say 3) and count up how many cards are in the first pile (hold pile), for example: 16 cards, and add up the digits of the number of cards in the first pile, for example:1+6=7, subtract that number from:
if the digits add up to 1-6, subtract from 7 or if the digits add up to 7-9 subtract from 16
7 fall in the category of 7-9 so subtract 7 from 16 to get 9
this answer becomes the prediction number, remember 9 is the prediction number.
now count up how many cards are in the second and third piles and add their digits all together
for example if there are 15 cards in the second pile and 21 cards in the third pile that would be 1+5+2+1=9  and 9 was the predicted number.
Physicist: The trick to this trick is hidden away in modular arithmetic.  Modular arithmetic is regular arithmetic, except that you state that some number is now equal to zero.  This has the effect of wrapping the number line into a loop.  The number you set to zero is called the “mod” or “modulus”.  So for example, counting in “mod 4” would be: 1, 2, 3, 0, 1, 2, 3, 0, 1, 2, …

mod 12

Clocks are just numbers mod 12.  If someone told you “it’s 17 o’clock”, you might be inclined to say “do you mean 5 o’clock?”  A mathematician would say “17 mod 12 equals 5 mod 12”.  You can write this [17]12 = [5]12, or 17 mod 12 = 5 mod 12.

You can pass addition, subtraction and multiplication through the mod (though not division, that’s a whole thing).  For example,

\begin{array}{ll}[13+18]_{12}=[13]_{12}+[18]_{12}\\\Leftrightarrow [31]_{12}=[1]_{12}+[6]_{12}\\\Leftrightarrow [7]_{12}=[1]_{12}+[6]_{12}\end{array}

An example for multiplication:

\begin{array}{ll}[13\cdot 5]_{12}=[13]_{12}[5]_{12}\\\Leftrightarrow [65]_{12}=[1]_{12}[5]_{12}\\\Leftrightarrow [5]_{12}=[1]_{12}[5]_{12}\end{array}

So we’ve got

[AB]_{M}=[A]_{M}[B]_{M} and [A+B]_{M}=[A]_{M}+[B]_{M}

But when you do math mod nine you get one more, strange property: a number mod 9, is equal to the sum of its digits mod 9.  In other words, if you write down a number as “abcd”, then [abcd]9=[a+b+c+d]9.  All the fancy tricks you may have learned in grade school involving nines all boil down to this property.  Personally, I’d say that this “nine property” is also what makes this seem more like a magic trick.  It doesn’t feel like any useful information would survive digit adding.

Here’s a quick proof!

Any number of the form “9…99” is equal to 0 mod 9, because they’re all multiples of 9, so when you subtract 9 enough times (1…11 times, in fact) you’re left with zero.

So [9…99]9 = [0]9, and adding 1 to both sides you get, [10…00]9=[1]9.  Now check this out!  Take a number like 3427.  You can figure out (with the help of a calculator or something) that [3527]9=[8]9.

Here’s a better way:

\begin{array}{ll}[3527]_9\\=[3\cdot 1000+5\cdot 100+2\cdot 10+7]_9\\=[3\cdot 1000]_9+[5\cdot 100]_9+[2\cdot 10]_9+[7]_9\\=[3]_9[1000]_9+[5]_9[100]_9+[2]_9[10]_9+[7]_9\\=[3]_9[1]_9+[5]_9[1]_9+[2]_9[1]_9+[7]_9\\=[3]_9+[5]_9+[2]_9+[7]_9\\=[3+5+2+7]_9\\=[17]_9\\=[8]_9\end{array}

The trick: [52]9=[7]9.  This trick amounts to saying: if [x+y]9=[7]9, then [x]9=[7-y]9.

y is the number of cards in the “hold pile”.  Adding up the digits has no effect on the value mod 9.  The subtraction (from 7 for 1≤y≤6, and from 16 for 7≤y≤9) amounts to subtracting from 7 mod 9.  So the “prediction number” is exactly [7-y]9.

x is the number of cards in all of the other piles.  It doesn’t matter if you look at x or the sum of x’s digits (don’t change mod 9).  It also doesn’t matter if you split x into different piles, since [A+B]M=[A]M+[B]M.

So there it is.  It also doesn’t matter that you’re using cards.  Were you so inclined, you could do this same trick with any number of arbitrary objects.  The only thing that changes is what number you subtract from (mod 9) to get the prediction number.  Cards: [52]9=[7]9.  With jokers: [54]9=[9]9.  A big box of matches: [300]9=[3]9.  Your mom: [1]9=[1]9.

Got friends who love counting stuff? If you have the time, this trick will work with any number of objects.

By the by, the “mod 9 invariance” thing was used by accountants in ye olde times to double check their summations.  Take the sum you got mod 9, and compare it to the sum of all the digits involved mod 9 (which is easier to do).  If you get the same number, then you probably did the original sum right.

Or, at the very least, if you did the sum wrong there’s a 8 in 9 chance you’ll catch it.

Update: Since this post was put up we’ve received more “nine-based” questions.  Rather than put them in a new post:
The original question was: I have been searching for dayyyys for this trick’s proof. I have had one professor tell me it involves mod 9 arithmetic and another say it’s digital root theorem. Any help??

STEP 1
Ask a friend to write down a number (any number with more than 3 digits will do, but to save time and effort you might suggest a maximum of 8 digits).
Example: 83 972 105
STEP 2
Ask them to add the digits.
Example: 8+3+9+7+2+1+0+5 = 35
STEP 3
Ask them to subtract this number from the original one.
Example: 83 972 105 – 35 = 83 972 070
STEP 4
Ask them to select any digit from this new number and strike it out, without showing you.
Example: 83 972 070
STEP 5
Ask them to add the remaining digits and write down the answer they get.
Example: 8+3+9+7+0+7+0 = 34
STEP 6
Ask them to tell you the number they get (34) and you will tell them which number they struck out.

SOLUTION
The way you do this is to subtract the number they give you from the next multiple of 9. The answer you get is the number they struck out.
Example: The next multiple of 9 here is 36 (9 x 4 =36)
36 – 34 = 2 and there you have your answer, easy isn’t it!
Note: If the number they give you after step 5 is a  multiple of 9, there are two possible answers  then you simply tell them that this time they crossed out either a 9 or a zero.

Physicist: In steps 2 and 3 you’re forcing your friend to create a new number X, such that [X]9 = [0]9.  Since [83972105]9 = [8+3+9+7+2+1+0+5]9 = [35]9 you have [83972105 – 35]9 = [0]9.  So X = 83972070.  Let’s call the digit they remove “D” (in this case, D=2).  Since the sum of X’s digits is [0]9, subtracting D leaves you with [-D]9 which is what you have at the end of step 5: [-D]9 =[34]9.The final subtraction done in the solution is subtracting negative D (leaving D).  That multiple of 9 that you find is still equal to [0]9.  It’s only purpose is to make the math a little easier to do in your head.  So in this case [-(-D)]9 = [-34]9 = [-25]9 = [-16]9 = [-7]9 = [2]9.  Or equivalently, [-34]9 = [0]9 + [-34]9 = [36]9 + [-34]9 = [2]9.  It may seem strange that negative numbers are the same as positive numbers, but in modular arithmetic “positive” and “negative” don’t mean much, since you can add or subtract the modulus whenever.  It’s like saying “negative 2 o’clock is equal to 10 o’clock” (just add 12!).

Another update:
The original question was: ok write three numbers, mix them around, subtract the two, pick a number out of those three and tell me the other two and i can guess your answer. how is it done?

Say you have a number like 537 then you you mix them up to make another number like 375 then subtract 375 from 537 and its 162 and ill pick a number lets say 6( if its a zero you cant use it) ill tell you 1 and 2 and you guess 6.

Physicist: Clever!  This trick is similar to the last one, and again involves forcing someone to come up with a number that’s equal to [0]9.

Say the original number is written “abc” (in the example a=5, b=3, c=7).  The new number is X = abc-bca (any rearrangement is fine).  Now check this out!

[X]9=[abc-bca]9=[abc]9-[bca]9=[a+b+c]9-[b+c+a]9=[a+b+c-b-c-a]9=[0]9.  This follows from the rules for mod 9 arithmetic (near the top of this post), namely that [A+B]M=[A]M+[B]M and [abcd…]9=[a+b+c+d+…]9.

[X]9=[0]9 is just a fancy way of saying that X is a multiple of 9.  Moreover (using that same rule again) the sum of X’s digits must also be a multiple of 9.  With this information in hand, given all but 1 digit (or even just the sum of all but one digit), you can find the remaining digit.

In the example X=162.  You know the sum of the digits must be a multiple of 9, so given 1 and 2, the remaining digit must be 6.

This system breaks down when the sum of the given digits is already 9.  For example, if you’re given 5 and 4, you might guess 0 or 9 (540 and 549 are both multiples of 9, after all), but only one of them can be the hidden digit.

Posted in -- By the Physicist, Brain Teaser, Math, Number Theory | 10 Comments

Q: What is a magnetic field?

Posted on November 3, 2010 by The Physicist

Physicist: Magnetic fields are nothing more than the result of combining an electric field with the effects of relativity.

First some background.  Magnetic fields were first defined in such a way that iron filings will point in the direction of (what became defined as) the field.  The reason why filings point in the direction of the field is a little round about, but luckily the physics turned out pretty clean.

The magnetic field was defined so that pictures like this made sense.

Classical physics: A when a charge moves in some direction it creates a magnetic field that wraps around it.  The direction of the field can be found using (one of) the “right hand rule(s)”.

When a charge moves it's called "current". A current (even a single moving charge) produces a field that wraps around it's direction of motion according to a right hand rule: point your right thumb along the current, and your fingers will curl in the direction of the field. The greater the charge or speed, the greater the field. Physicists, being clever, generally label the magnetic field as "B" and current as "I".

In addition to creating magnetic fields, moving charges also experience force from a magnetic field that can be found using another right hand rule.  Point your fingers (on your right hand, of course) in the direction the charge is moving, curl your fingers in the direction of the field, and your thumb will be pointing in the direction of the force.

The charge is moving up. When the magnetic field points into your computer screen, the charge will feel a force pushing left. When the magnetic field points out of your screen the charge is pushed to the right.

By carefully twisting your hand about you should be able to figure out that two like charges, traveling side by side in the same direction, will experience a magnetic attraction.  Of course, likes repel (and opposites attract) so the two charges will feel an electric force repulsion.  This electric repulsion is always greater than the magnetic attraction, so the particles still fly apart, just slower.

The exception is things like electrical wires.  There’s an equal amount of positive and negative charge in the wires, so the magnetic force is all that’s left.

But this all begs the question; how fast do the particles have to be moving so that the magnetic field they generate to pull them together is strong enough to balance the electric force pushing them apart?  The answer is exactly the speed of light (not a coincidence).

Also, if wires with current in them experience magnetic forces, but not electric forces, doesn’t that make magnetic fields “real”?  Not quite.

Relativistic physics: Consider the two particles flying along.  If you’re moving with them, then they’re sitting still from you’re point of view, and they just fly apart (stationary charges don’t generate a magnetic field).  But (classically) if the charges are moving past you they generate a magnetic field, and that keeps them from flying apart quite as fast.

However, if you write down how much time dilation the charges will experience from moving past you, the slowness of their separation is explained away.  The “magnetic field” is just an illusion created by the slowing of time.

Classically, two charges moving in the same direction will fly apart slower than normal because of their magnetic field. Relativity shows that this effect is more correctly explained using time dilation: they move through time slower, so they fly apart slower.

From this point of view it makes perfect sense that in order to arrest the repulsion of the two charges, they would need to be moving at the speed of light.  Like charges repel, period.  So, in order to not fly apart, they must not experience any time at all (no time passes at light speed).

Now consider two parallel, current carrying wires.  When current is flowing electrons (negative charge) are moving through the wire, and the protons (positive charge) in the nuclei of the atoms stay where they are.  The protons see the protons in the other wire normally, but since the electrons in the other wire are moving, the protons see the electrons as closer together because of a relativistic effect called “length contraction“.  The electrons, in turn, see the protons as moving and so experience the same effect.  The end result is that every particle in both wires sees the other wire as have an opposite net charge.

The protons in both wires see the electrons in both wires as denser due to length contraction. So they're happy in their own wire and also try to attract the other wire. The electrons experience the same thing. If the current in the wires run in opposite directions, then (for the same reasons) the wires repel each other.

So if magnetic fields are just weird relativistic effects, then where do magnetic poles, bar magnets, and whatnot come from?

So far we’ve got that current flowing in the same direction down two wires makes those wires attract, and current flowing in opposite directions makes them repel.  To get the magnets of your childhood (some of our childhoods) all you have to do is turn that wire into a loop.

A loop of wire behaves like a bar magnet. When two are oriented the same way they attract, and when oriented oppositely they repel.

This perspective, that all magnetic dipoles (bar magnets, big and small) are reducible to current loops, helps explain a lot of things; like why there are no magnetic monopoles, and why atoms generate magnetic fields (an orbiting electron is essentially a current loop).

The more common view of the magnetic field (among physicists) is that it is merely part of a larger structure called an “electromagnetic field”.  Funny story: when Einstein first wrote his big-shit paper about relativity the whole “time and space” thing was more of a side note.  What he was really interested in  was combining the electric and magnetic forces under one roof.  In a nutshell, if a charge is moving through space in the presence of a magnetic field, it feels a push in a new direction dictated by the right hand rule (above).  If a charge is moving through time, in the presence of an electric field it also feels a push in a new direction (generally to or away from another charge).  So electric fields work on charges moving through time, and magnetic fields work on charges moving through space.  Special relativity shows that the distinction between time and space are academic (Without batting an eye a physicist will say things like “north and east and future are three orthogonal directions”).  So the electromagnetic field is just a more compact way of dealing with the two fields all at once.

Personally, I find that when you consider the source of a magnetic field (moving charges), combining relativity with the Coulomb force explains everything you need.  Albeit, not very clearly, or succinctly.  The rules about conservation of magnetic field lines, for example, fall out of conservation of charge and momentum.  But most people would rather just work with the (illusionary) magnetic field lines than go back to basics every five minutes.  And why not?  It makes the math much easier.

Posted in -- By the Physicist, Physics, Relativity | 32 Comments